Complete Solutions to LEVEL-III of chapter MOVING CHARGES AND MAGNETISM of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | MOVING CHARGES AND MAGNETISM |
| NARAYANA | LEVEL-III |
| Number Of Questions | 45 |
| Academic Year | 2023-24 |
Two wires AO and OC carry equal currents i as shown in Fig. One end of both the wires extends to infinity. Angle AOC is `alpha`. The magnitude of magnetic field is point P on the bisector of these two wires at a distance r from point O is

View SolutionA current of `1A` is flowing through a straight conductor of length `16cm`. The magnetic induction `(` in tesla `)` at a point `10cm` from the either end the wire is `:`
View SolutionEach of two long parallel wires carries a constant current `i` along the same direction . The wires are separated by a distance `2I`. The magnitude of resultant magnetic induction in the symmetric plane of this system located between the wires at a distance `R` from each wire will be
View SolutionA long straight wire of radius `a` carries a steady current `i`. The current is uniformly distributed across its cross section. The ratio of the magnetis field at `(a)//(2) and (2a)` is
View SolutionA horizontal rod of mass `10g` and length `10cm` is placed on a smooth plane inclined at an angle of `60^@` with the horizontal with the length of the rod parallel to the edge of the inclined plane. A uniform magnetic field induction B is applied vertically downwards. If the current through the rod is `1*73ampere`, the value of B for which the rod remains stationary on the inclined plane is
View Solution`PQ` is a uniform rod of length `l` and mass `m` carrying current `i` and is suspended in uniform magnetic field of induction `vec(B)` acting inward as shown in figure. The tension in each string is

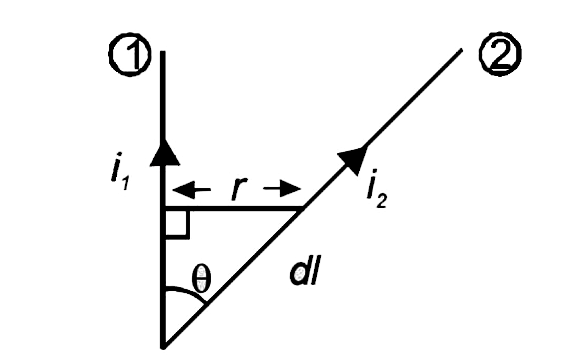
View SolutionWires `1 and 2` carrying currents `i_(1) and i_(2)` respectively are inclined at an angle `theta ` to each other. What is the force on a small element `dl` of wire `2` at a distance of `r` from wire 1 ( as shown in figure) due to the magnetic field of wire 1`?
View SolutionLet current `i=2A` be flowing in each part of a wire frame as shown in Fig. 1.138. The frame is a combination of two equilateral triangles ACD and CDE of side 1 m. It is placed in uniform magnetic field ` B= 4 T` acting perpendicular to the plane of frame. The magnitude of magnetic force acting on the frame is
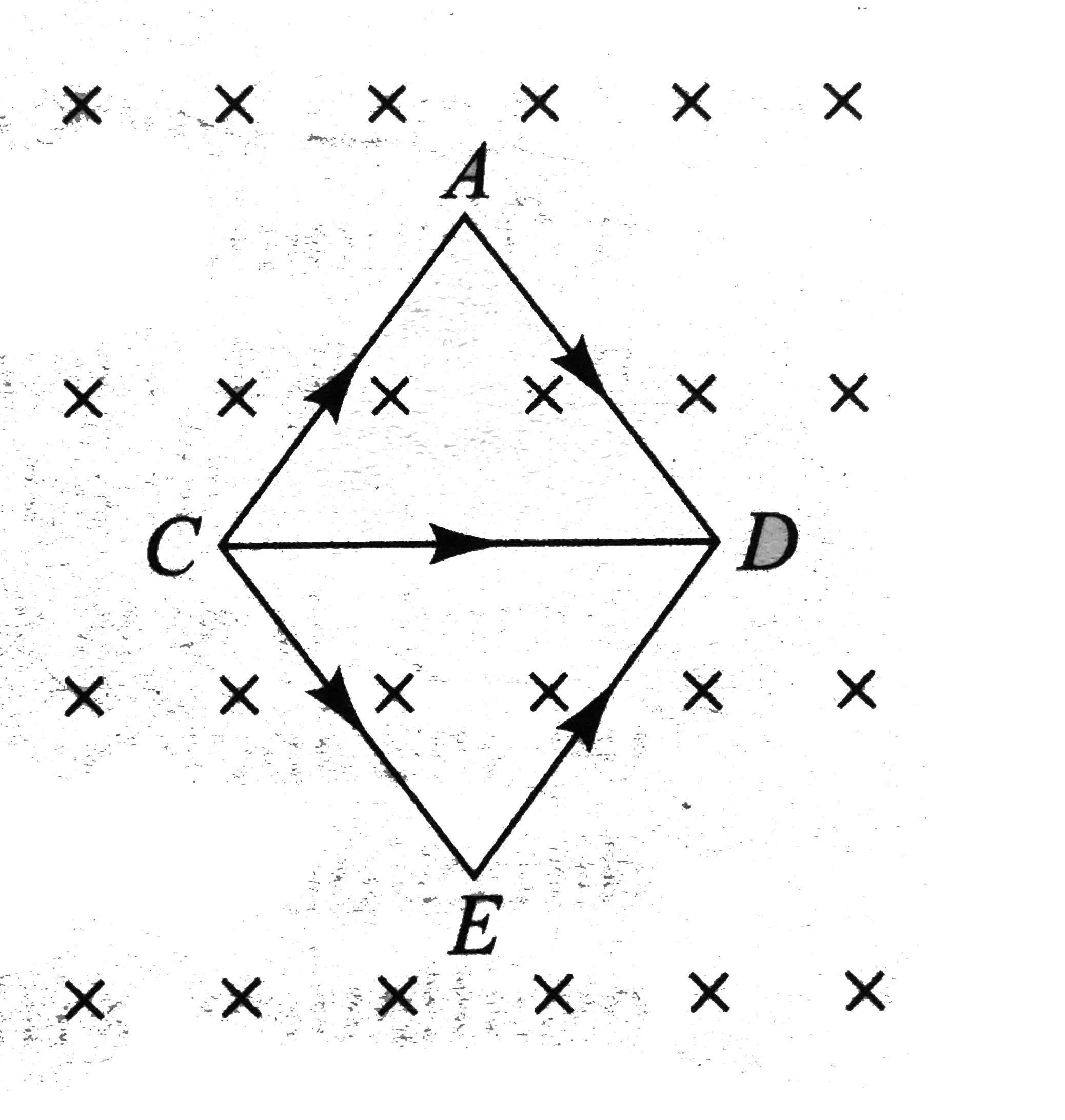
The pithc of the helical path followed by the particle is p. The radius of the helix will be
View SolutionA wire bent in the form a right angled triangle `PQR`, carries a current `1A`. It is placed in a region of a uniform magnetic field `B=0.2T`. If `PR=1m`, the net force on the wire is

View SolutionTwo long parallel conductors carry currents `i_(1)=3A` and `i_(2)=3A` both are directed into the plaane of paper. The magnitude of resultant magnetic field at point `'P' ,` is

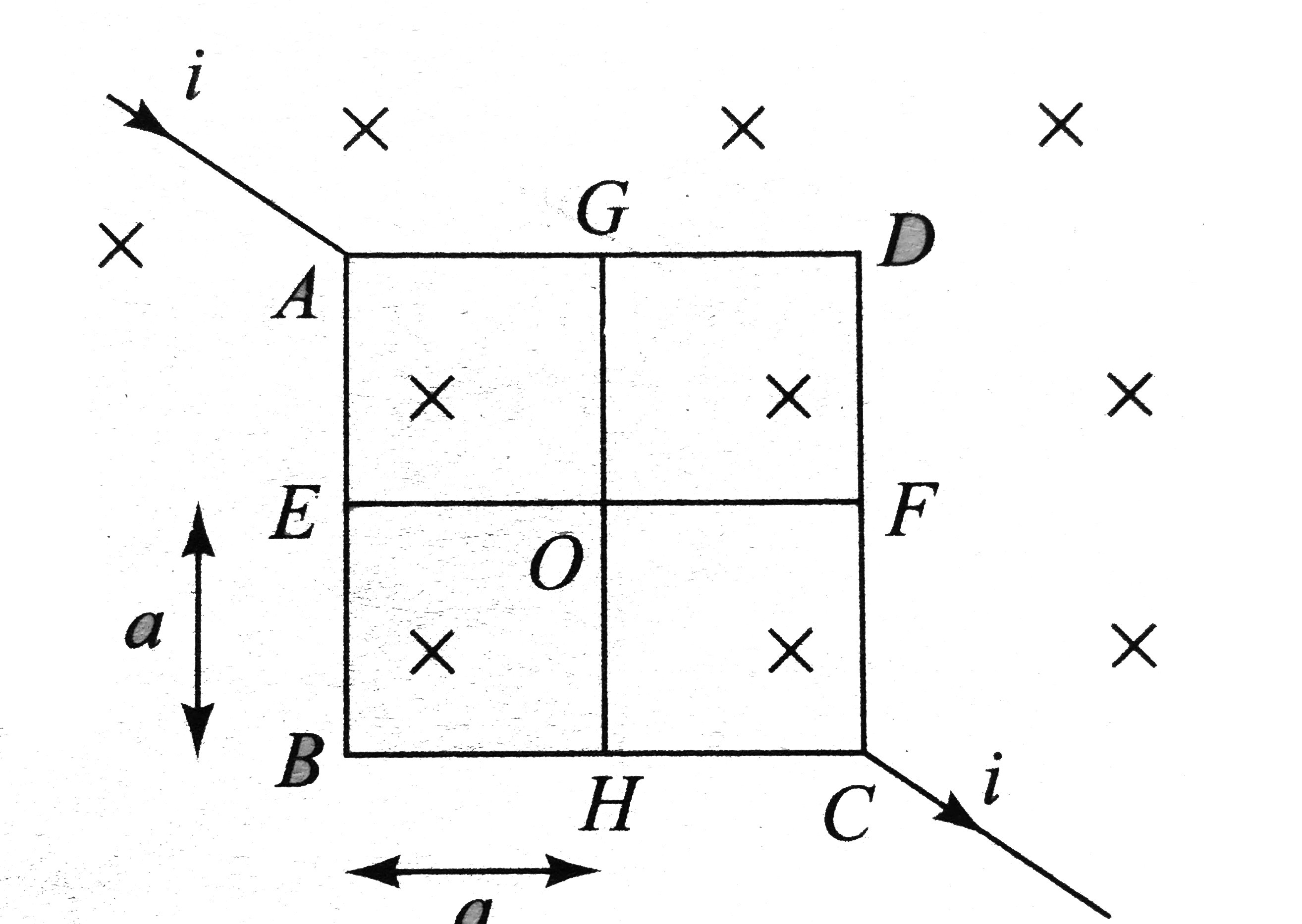
View SolutionIn Fig. there is a uniform conducting structure in which each small square has side a. The structure is kept in a uniform magnetic field B. Then the magnetic force on the structure will be
View SolutionA current `-` carrying circular loop of radius `R` is placed in the `XY-` plane with centre at the origin. Half of the loop with `xgt0` is now bent so that is now lies in the `XY-` plane
View SolutionA long straight wire along the `z`- axis carries a current ` I` in the negative ` z- direction` . The magnetic vector field ` vec(B)` at a point having coordinates (x,y) in the ` Z = 0` plane is
View SolutionThe magnetic induction at `O` due to a current in conductor shaped as shown in figure

View SolutionA wire is bent in the form of a circular arc with a straight portion AB. Magnetic induction at O when current flowing in the wire, is
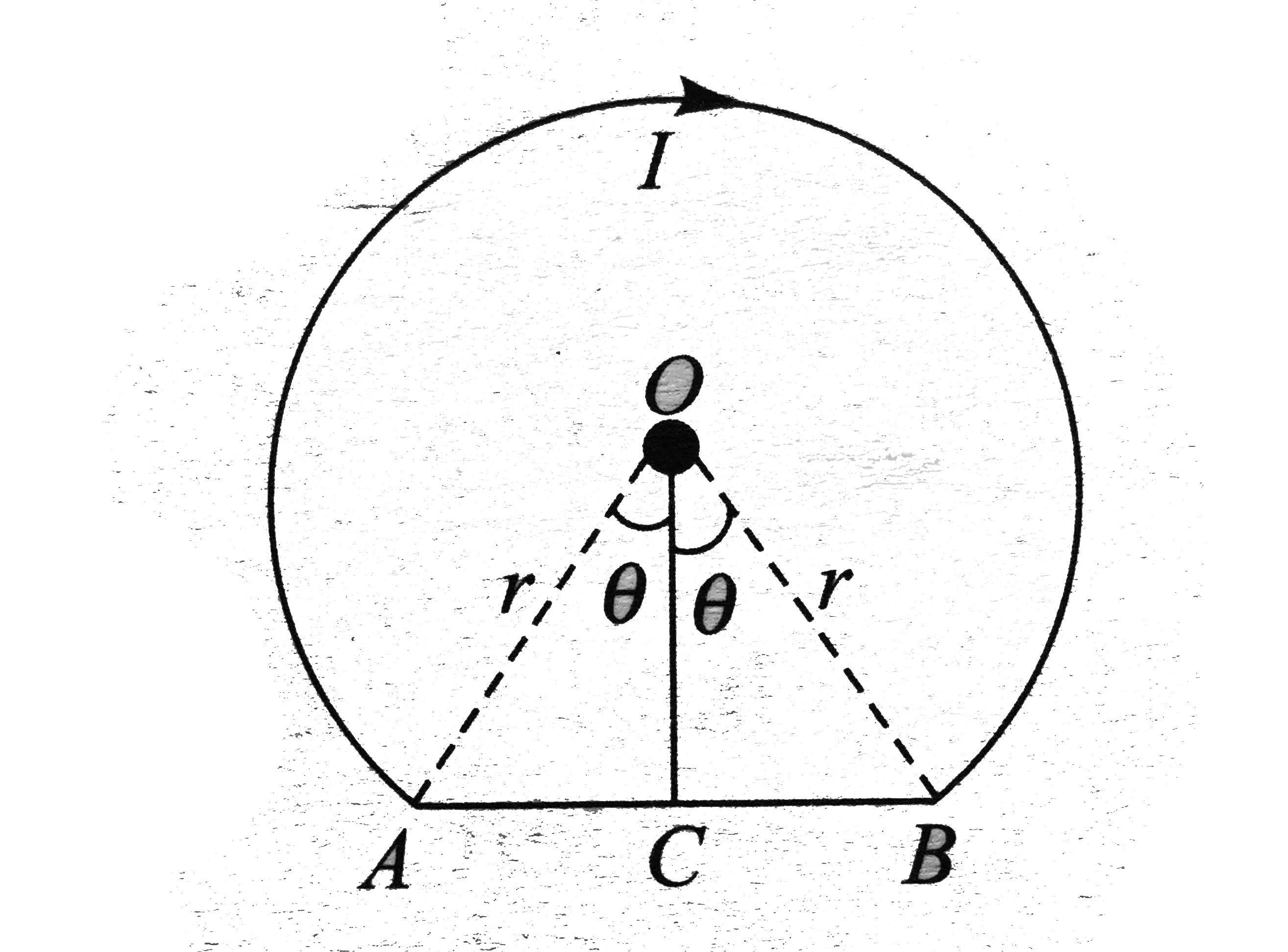
View SolutionA wire of length `'L'` is bent in to an arc of a circle and found to subtentd and angle of `'theta'` radians at the centre.If a current of `'I'` is passed through it, the magnetic induction at the center of the circle is
View SolutionA wiere of length `L` is shaped into a cricle and then bent in such a way that the two semi`-` circle are perpendicular. The magnetic moment of the system when current `I` flows through the system is
View SolutionA square frame of side `l` carries a current produces a field `B` at its centre. The same current is passed through a circular loop having same perimeter as the square. The field at its centre is `B'`, the ratio of `B//B'` is
View SolutionA circular coil of 100 turns and effective diameter `20cm` carries a current of `0.5A`. It is to be turned in a magnetic field of `B=2.0T` from a position in which the normal to the plane of the coil makes an angle `theta` equals to zero to one in which `theta` equals to `180^(@)`. Thw work required in this process is
View SolutionA long solenoid has `200 turns per cm` and carries a current `i`. The magnetic field at its centre is `6.28xx10^(-2) weber//cm^(2)`. Another long soloenoid has `100 turns per cm` and it carries a current `(i)/(3)`. The value of the magnetic field at its centre is
View SolutionFind the magnetic induction at point `O` if the current carrying wire is in the shape shown in the figure.
 .
.
View SolutionTwo circular coils `P` and `Q` are made from similar wire but radius of `Q` is twice that of `P`. Relation between the values of potential difference across them so that the magnetic induction at their centers may be the same is
View SolutionA charged particle of charge `5mc` and mass `5 gm` is moving with a constant speed `5m//s` . In a uniform magnetic field on a curve `x^(2)+y^(2)=25`. Where `x` and `y` are in meter. The value of magnetic filed required will be
View SolutionVelocity and acceleration vectors of a charged particle moving in a magnetic field at some instant are `vec(v)=3hat(i)+4hat(j)` and `vec(a)=2hat(i)+xhat(j)`. Selcet the wrong alternative.
View SolutionA charged particle enters a magnetic field at right angles to the field. The field exists for a length equal to `1.5` times the radius of circular path of particle. The particle will be deviated from its path by
View SolutionA particle of mass `m`and charge `q`, moving with velocity `v` enters Region `II` normal to the boundary as shown in the figure. Region `II` has a uniform magnetic field `B` perpendicular to the plane of the paper . The length of the region `II` is `l`. Choose the correct choice(s).

View SolutionA proton accelerated by a potential difference `500 KV` moves though a transverse field of `0.51 T` as shown in figure. The angle `theta` through which the proton deviates from the intial direction of its motion is
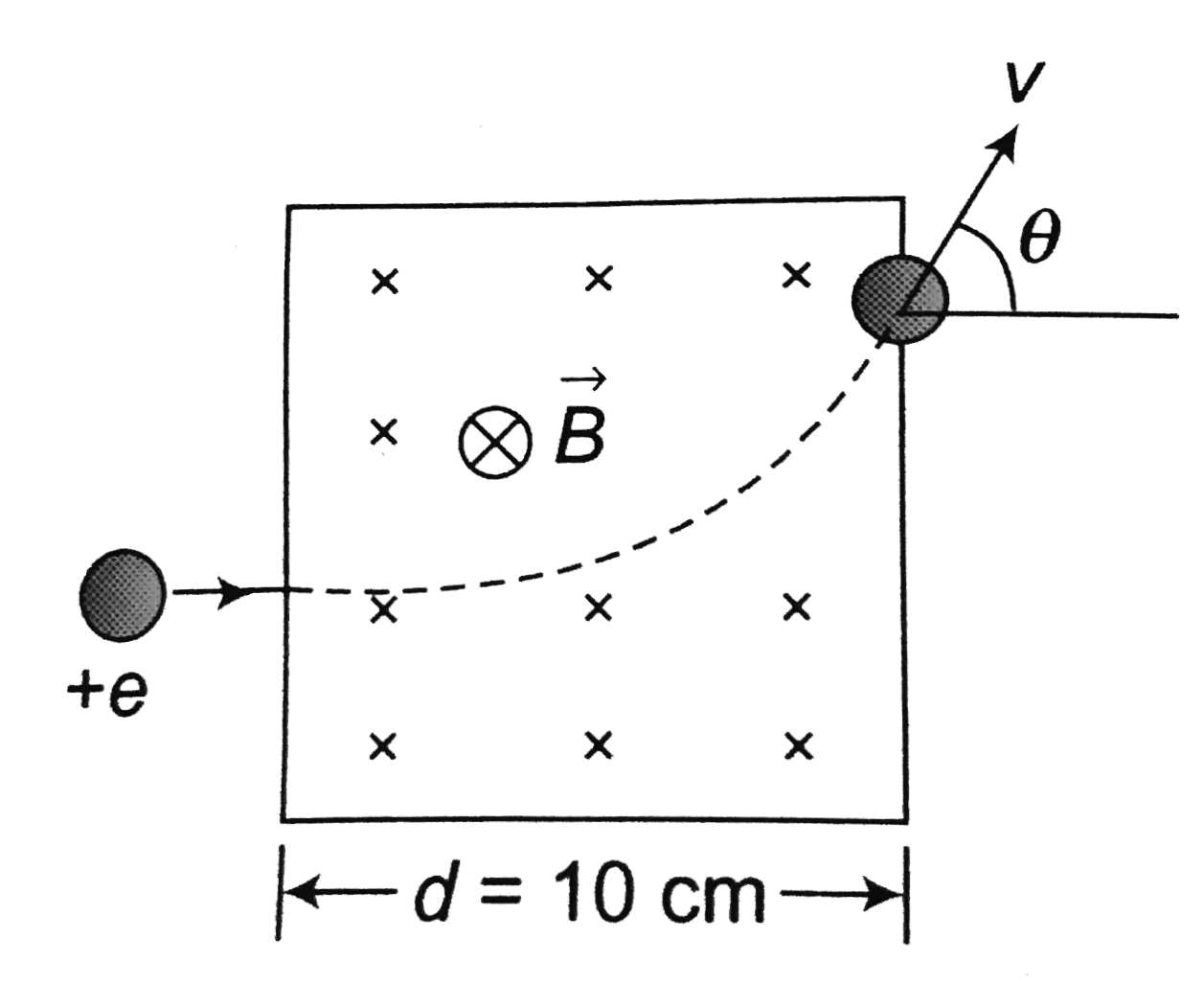
View SolutionTwo identical charged particles enter a uniform magnetic field with same speed but at angles `30^(@)` and `60^(@)` with field Let a,b and c be the ratio of their time periods, radii and pitches of the helical paths than .
View SolutionAn electron whose `e//m` is `1.76X10^(11)c//kg` enter a region `2X10^(-3)` tesla with a velocity of `3X10^(6)m//sec` in a direction making an angle of `45^(@)` with the field. The pitch of its helical path in the region is
View SolutionTwo charged particles traverse identical helical paths in a completely opposite sense in a uniform magnetic field `vec(B)=B_(0)hat(K)`
View SolutionFor a positively charged particle moving in a `x-y` plane initially along the `x-axis` , there is a sudden change in its path due to the presence of electric and//or magnetic fields beyond `p` . The curved path is shown in the ` x- y `plane and is found to be non - circular. Which one of the following combinations is possible ?
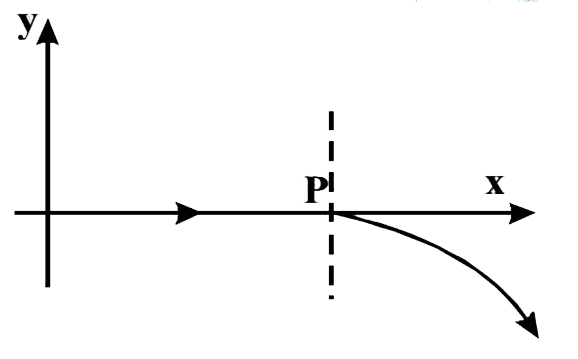
View SolutionA metallic block carrying current `I` is subjected to a uniform magnetic induction `vec(B) as shown in Figure . The moving charges experience a force ` vec(F) given by ….. Which results in the lowering of the potential of the face ……. Assume the speed of the carries to be `v` .
View SolutionTwo parallel beams of protons and electrons, carrying equal currents are fixed at a separation d. The protons and electrons move in opposite directions. There is a point P on the straight perpendicular line joining the two beams at a distance x from one beam. The magnetic field at this point is B. If B is plotted against x, it can be represented by the curve.
View SolutionThree very long straight conductors are arranged parallel to each other in a plane and have resistances in the ration `1:2:3` . They are connected in parallel to a battery of negligible internal resistance such that the currents in all three wires are in the same direction. The distance between the first two conductors is `x` and the distance between the second and third conductors is `y`. If the middle conductors is in equilibrium, the ratio `x:y` is
View SolutionTwo long wires are placed parallel to each other `10cm` apart as shown in figure. The magnetic field at point `P` is

View SolutionTwo long parallel wires are at a distance ` 2d` apart. They carry steady equal currents flowing out of the plane of the paper , as shown. The variation of the magnetic field `B` along the line `XX` is given by
View SolutionA circular loop of radius `R=20` cm is placed in a uniform magnetic field `B=2T` in `xy`-plane as shown in figure. The loop carries a current `i=1.0 A` in the direction shown in figure. Find the magnitude of torque acting on the loop.

View SolutionA non conducting disc of radius `R`, charge `q` is rotating about an axis passing through its centre and perpendicular to its plane with an angular velocity `omega` charge `q` is uniformly distributed over its surface. The magnetic moment of the disc is
View SolutionA galvanometer of resistance `5 Omega` is connected in series with a resistance of `0.2Omega` to a battery of negligible internal resistance. The deflection is noted. If the `0.2Omega` resistance is replaced by `2 Omega` resistance, the value of shunt resistance to be connected to the galvanometer to maintain the same deflection is
View SolutionA thin wire of length `L` is made of an insulating material. The wire is bent to form a circular loop, and a positive charge `q` is distributed uniformly around the circumference of the loop. The loop is then set into rotation with angular speed `omega` around an axis through its centre. If the loop is in the region where there is a uniform magnetic field `vec(B)` directed parallel to the plane of the loop, calculate the magnitude of the magnetic torque on the loop.
View SolutionA rigid circular loop of radius r & mass m lies in the xy plane on a flat table and has a current I flowing in it At this particular place the earth's magnetic filed is `B =B_(x) hati +B_(y) hatj` How large must I be before one edge of the loop will lift from table
Repeat if ,`oversetrarr(B) = oversetrarr(B_(x)) hati + oversetrarr(B_(z)) hatk` .
View SolutionA hollow sphere has charge `'q'` which is uniformly distributed over its surface and rotating about its axis .Find the magnetic moment of the sphere
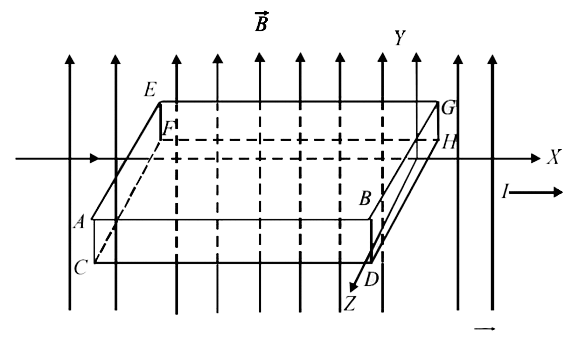
View SolutionA loop carrying current `I` lies in the ` x-y ` plane as shown in the figure . The unit vector ` hat k` is coming out of the plane of the paper . The magnetic moment of the current loop is
View SolutionElectric field strength `bar(E)=E_(0)hat(i)` and magnetic induction `bar(B)=B_(0)hat(i)` exists in a region. A charged particle `'q'` is released from rest at origin. Work done by both the fields is after certain time is
View SolutionA long current carrying wire, carrying current such that it is flowing out from the plane of paper, is placed at `O`. A steady state current is flowing in the loop `ABCD`. Then

View Solution
LEVEL-III questions and complete solutions for chapter MOVING CHARGES AND MAGNETISM of NARAYANA PHYSICS (HINGLISH) of Class 12
All Exercises from NARAYANA Class 12 PHYSICS MOVING CHARGES AND MAGNETISM
- C.U.Q (AMPERE.S CIRCUITAL LAW MAGNETIC FIELD DUE TO STRAIGHT CONDUCTOR )
- C.U.Q (CONVERSION OF MCG INTO AMMETER)
- C.U.Q (CONVERSION OF MCG INTO VOLTMETER)
- C.U.Q (CURRENT LOOP AND MAGNETIC DIPOLE)
- C.U.Q (FORCE BETWEEN TWO PARALLEL CURRENT CARRYING CONDUCTORS)
- C.U.Q (FORCE ON A MOVING CHARGE IN MAGNETIC FIELD)
- C.U.Q (FORCE ON A CURRENT CARRYING CONDUCTOR IN A MAGNETIC FIELD)
- C.U.Q (MOVING COIL GALVANOMETER)
- C.U.Q (TANGENT GALVANOMETER)
- C.U.Q (TORQUE ON CURRENT LOOP)
- C.U.Q-KEY
- EXERCISE - 1(C.W) (AMPERE.S CIRCUITAL LAW BIOT-SAVART LAW AND ITS APPLICATIONS)
- EXERCISE - 1(C.W) (CONVERSION OF MCG INTO AMMETER AND VOLTMETER)
- EXERCISE - 1(C.W) (CURRENT LOOP AND MAGNETIC DIPOLE)
- EXERCISE - 1(C.W) (CYCLOTRON)
- EXERCISE - 1(C.W) (FORCE ACTING A MOVING CHARGE IN MAGNETIC FIELD)
- EXERCISE - 1(C.W) (FORCE BETWEEN TWO PARALLEL CURRENT CARRYING CONDUCTORS)
- EXERCISE - 1(C.W) (FORCE ON A CURRENT CARRYING CONDUCTOR IN A MAGNETIC FIELD)
- EXERCISE - 1(C.W) (MOVING COIL GALVANOMETER)
- EXERCISE - 1(C.W) (SOLENOID AND TOROID)
- EXERCISE - 1(C.W) (TANGENT GALVANOMETER)
- EXERCISE - 1(C.W) (TORQUE ON CURRENT LOOP)
- EXERCISE - 1(H.W) (AMPERE.S CIRCUITAL LAW BIOT-SAVART LAW AND ITS APPLICATIONS
- EXERCISE - 1(H.W) (CONVERSION OF MCG INTO AMMETER AND VOLTMETER)
- EXERCISE - 1(H.W) (CYCLOTRON)
- EXERCISE - 1(H.W) (FORCE ACTING ON A MOVING CHARGE IN MAGNETIC FIELD)
- EXERCISE - 1(H.W) (FORCE BETWEEN TWO PARALLEL CURRENT CARRYING CONDUCTORS)
- EXERCISE - 1(H.W) (FORCE ON CURRENT CARRYING CONDUCTOR IN A MAGNETIC FIELD)
- EXERCISE - 1(H.W) (MOVING COIL GALVANOMETER)
- EXERCISE - 1(H.W) (SOLENOID AND TOROID)
- EXERCISE - 1(H.W) (TORQUE ON CURRENT LOOP)
- EXERCISE - 2(C.W) (AMPERE.S CIRCUITAL LAW BIO-SAVART LAW AND ITS APPLICATIONS)
- EXERCISE - 2(C.W) (CONVERSION OF MCG INTO AMMETER AND VOLTMETER)
- EXERCISE - 2(C.W) (CURRENT LOOP AND MAGNETIC DIPOLE)
- EXERCISE - 2(C.W) (FORCE ACTING ON A MOVING CHARGE IN MAGNETIC FIELD)
- EXERCISE - 2(C.W) (FORCE BETWEEN TWO PARALLEL CURRENT CARRYING CONDUCTORS)
- EXERCISE - 2(C.W) (MOVING COIL GALVANOMETER)
- EXERCISE - 2(C.W) (SOLENOID AND TOROID)
- EXERCISE - 2(C.W) (TANGENT GALVANOMETER)
- EXERCISE - 2(C.W) (TORQUE ON CURRENT LOOP)
- EXERCISE - 2(H.W) (AMPERE.S CIRCUITAL LAW BIO-SAVART LAW AND ITS APPLICATIONS)
- EXERCISE - 2(H.W) (CURRENT LOOP AND MAGNETIC DIPOLE)
- EXERCISE - 2(H.W) (FORCE ACTING A MOVING CHARGE IN MAGNETIC FIELD)
- EXERCISE - 2(H.W) (SOLENOID AND TOROID)
- EXERCISE - 2(H.W) (TANGENT GALVANOMETER)
- EXERCISE - 3
- EXERCISE - 4
- ILLUSTRATION
- Level -II (H.W)
- Level =I (H.W)
- LEVEL-I(C.W)
- Level-II(C.W)
- LEVEL-III
- LEVEL-V (SINGLE ANSWER QUESTION)
- LEVEL-VI (SINGLE ANSWER QUESTION)
- NCERT Based Questions
- W.E.