Complete Solutions to INEQUALITIES of chapter CHAPTERWISE REVISION (STAGE 3) of Class 9 book with complete answers and questions
| Class 9 | MATHS |
| Chapter | CHAPTERWISE REVISION (STAGE 3) |
| SELINA PUBLICATION | INEQUALITIES |
| Number Of Questions | 7 |
| Academic Year | 2023-24 |
In triangle ABC , the internal bisector of ` angle A angle B and angle C ` meet at point I . Prove that :
` (1)/(2) (AB+ BC + CA) lt AI+ BI + CI `
View SolutionIn triangle ABC, `AB gt AC` and D is a point in side BC. Show that : `AB gt AD`.
View SolutionThe P lies on side AB of an equilateral triangle ABC. Arrange AC, AP and CP in descending order.
View SolutionIn triangle ABC, side AC is greater than side AB. If the internal bisector of angle A meets the opposite side at point D, prove that : `angleADC` is greater than `angleADB`.
View SolutionIn a triangle ABC, side AC is greater than side AB and point D lies on side BC such that AD bisects angle BAC. Show that:
` angle ADB ` is acute.
View SolutionO is any point in the interior of a triangle ABC.
Prove that :
` OB + OC lt AB + AC.`
 _Q01.png" width="60%">
_Q01.png" width="60%">
View SolutionThe given figure shows an equilateral triangles ABC and D is point in AC.
Prove that :
(i) ` AD lt BD `
(ii)` BC gt BD `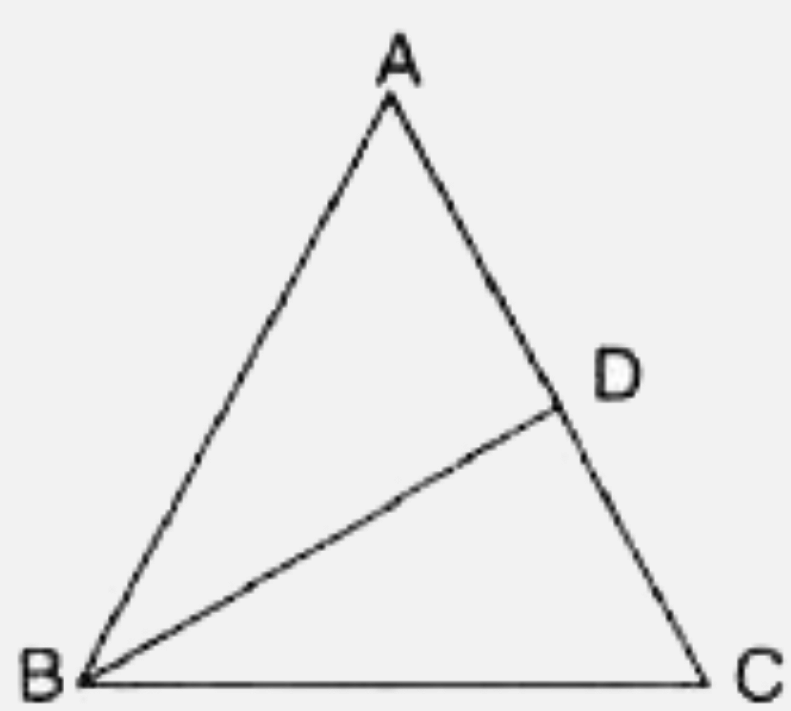
View Solution
INEQUALITIES questions and complete solutions for chapter CHAPTERWISE REVISION (STAGE 3) of ICSE of Class 9
All Exercises from SELINA PUBLICATION Class 9 MATHS CHAPTERWISE REVISION (STAGE 3)
- AREA
- AREA AND PERIMETER OF PLANE FIGURES
- CIRCLE
- CO-ORDINATE GEOMETRY
- COMPOUND INTEREST
- CONSTRUCTIONS OF POLYGONS
- DISTANCE FORMULA
- FACTORS
- GRAPHICAL SOLUTION
- INDICES
- INEQUALITIES
- ISOSCELES TRIANGLES
- LOGARITHM
- MEAN AND MEDIAN
- MID- POINT THEOREM
- PYTHAGORAS THEOREM
- RATIONAL AND IRRATIONAL NUMBERS
- RECTILINEAR FIGURES
- SIMUTANEOUS EQUATIONS
- SOLIDS
- STATISTICS
- TRIANGLES
- TRIGONOMETRY