Complete Solutions to MID- POINT THEOREM of chapter CHAPTERWISE REVISION (STAGE 3) of Class 9 book with complete answers and questions
| Class 9 | MATHS |
| Chapter | CHAPTERWISE REVISION (STAGE 3) |
| SELINA PUBLICATION | MID- POINT THEOREM |
| Number Of Questions | 9 |
| Academic Year | 2023-24 |
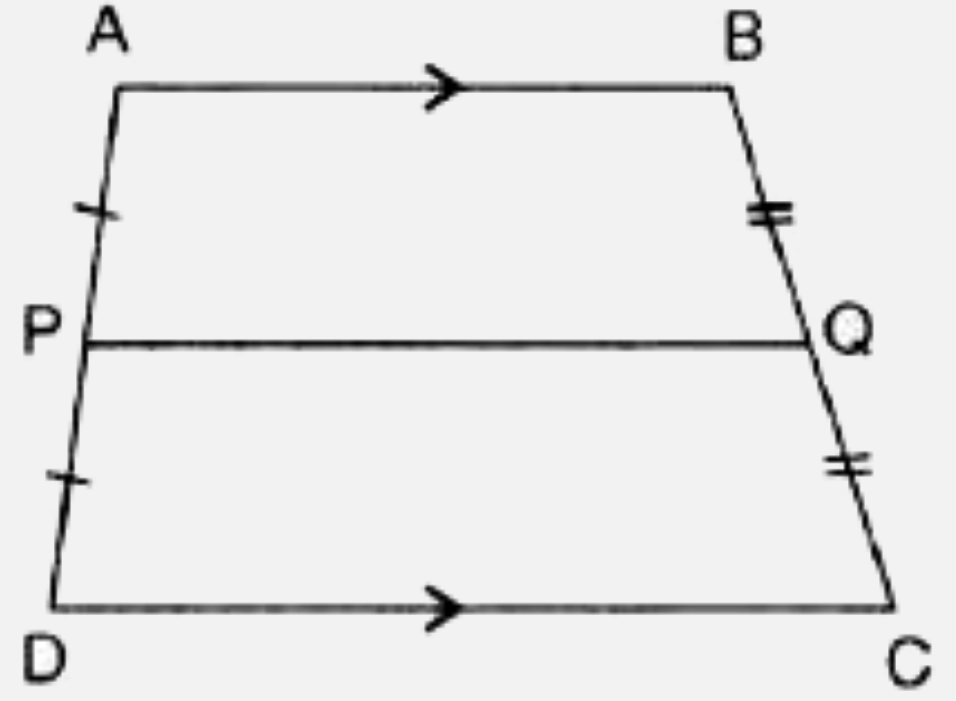
In the figure , given below. ABCD is a trapezium in which AB /DC , P is mid- point of AD and Q is mid-point of Bc.
Write a relations connecting AB , PQ and DC.
(ii) Find DC. If AB = 16 cm and PQ = 23 cm
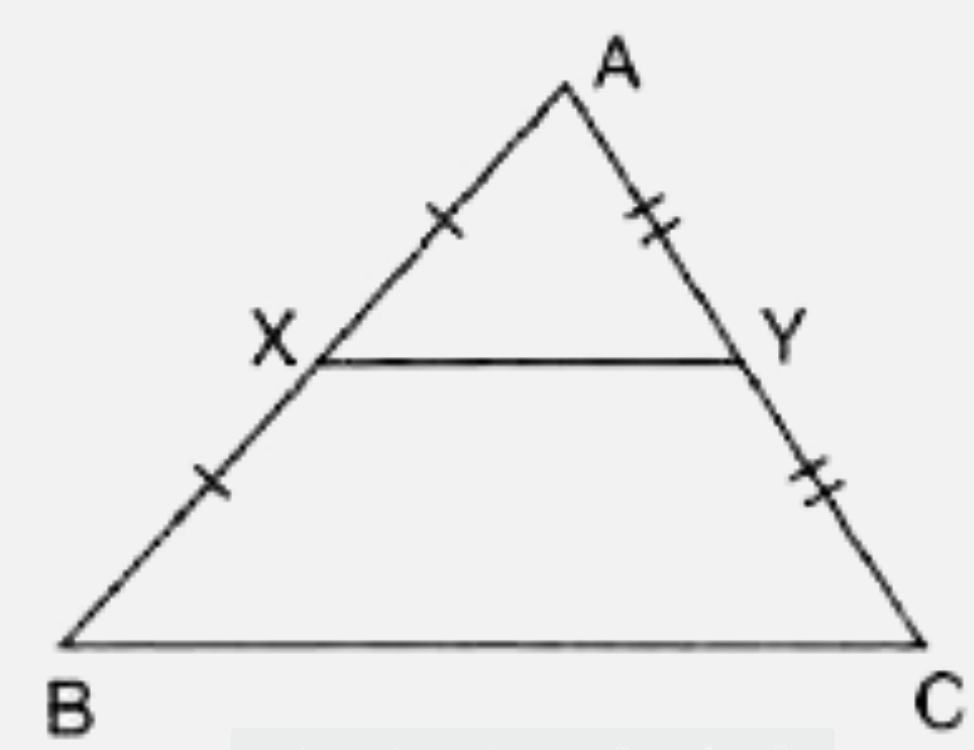
View SolutionIn the figure, given below X and Y are the mid-points of AB and AC respectively. Given that BC = 6 cm, AB = 5-4 cm and AC = 5-0 cm, calculate the perimeter of trapezium YXBC.
View SolutionIn triangle ABC, D is a point in side AB such that AB = 4AD and E is a point in AC such that AC = 4AE. Show that BC : DE = 4:1
View SolutionIn triangle ABC, D and E are mid-points of sides AB and BC respectively. Also, F is a point in side AC so that DF is parallel to BC
(i) Prove that DBEF is a parallelogram.
View SolutionIn triangle ABC, D and E are mid-points of sides AB and BC respectively. Also, F is a point in side AC so that DF is parallel to BC
Find the perimeter of parallelogram DBEF, if AB = 10 cm, BC = 8.4 cm and AC = 12 cm.
View SolutionM and N divide the side AB of a triangle ABC into three equal parts . Through M and N, lines are drawn parallel to BC and intersecting AC at points P and Q respectively. Prove that P and Q divide AC into three equal parts.
View SolutionIn parallelogram ABCD, E is mid-point of CD and through D, a line is drawr parallel to EB to meet CB produced at point G and to cut AB at point F. Prove that:
` 2 xx AD = GC `
View SolutionIn parallelogram ABCD, E is mid-point of CD and through D, a line is drawn parallel to EB to meet CB produced at point G and to cut AB at point F. Prove that :
DG = 2EB
View SolutionThe given figure shows a quadrilateral ABCD in which E, F, G and H are the midpoints of consecutive sides of ABCD. Again P, Q, R and S are the mid-points of the consecutive sides of quadrilateral EFGH. If EFGH is a rectangle, show that : PQRS is a rhombus.
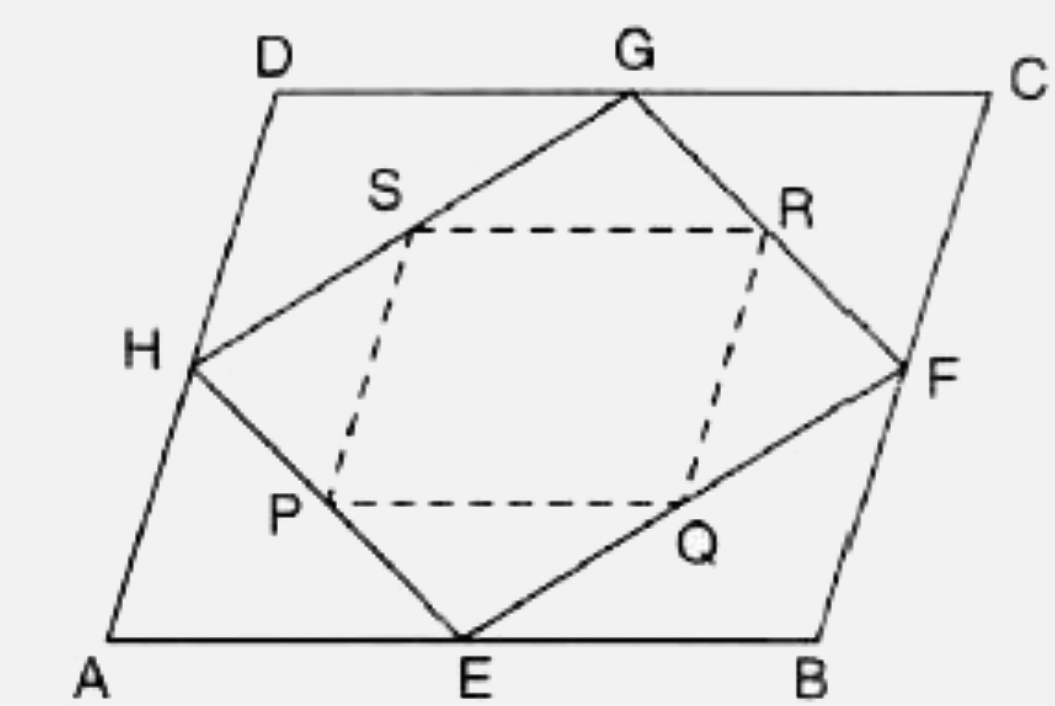
View Solution
MID- POINT THEOREM questions and complete solutions for chapter CHAPTERWISE REVISION (STAGE 3) of ICSE of Class 9
All Exercises from SELINA PUBLICATION Class 9 MATHS CHAPTERWISE REVISION (STAGE 3)
- AREA
- AREA AND PERIMETER OF PLANE FIGURES
- CIRCLE
- CO-ORDINATE GEOMETRY
- COMPOUND INTEREST
- CONSTRUCTIONS OF POLYGONS
- DISTANCE FORMULA
- FACTORS
- GRAPHICAL SOLUTION
- INDICES
- INEQUALITIES
- ISOSCELES TRIANGLES
- LOGARITHM
- MEAN AND MEDIAN
- MID- POINT THEOREM
- PYTHAGORAS THEOREM
- RATIONAL AND IRRATIONAL NUMBERS
- RECTILINEAR FIGURES
- SIMUTANEOUS EQUATIONS
- SOLIDS
- STATISTICS
- TRIANGLES
- TRIGONOMETRY