Complete Solutions to Exercise of chapter Areas of Parallelograms and Triangles of Class 9 book with complete answers and questions
| Class 9 | MATHS |
| Chapter | Areas of Parallelograms and Triangles |
| MBD | Exercise |
| Number Of Questions | 80 |
| Academic Year | 2023-24 |
Match the following:

 03:50View Solution
03:50View SolutionMatch the following :
 .01:40View Solution
.01:40View SolutionA diagonal of parallelogram divides it into four triangles of equal area.
04:33View SolutionParallelogram on the same base and between the same parallels (i.e. of equal altitude) are equal in area.
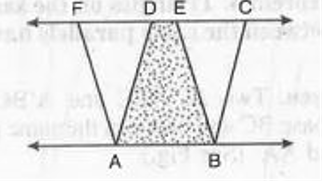 08:57View Solution
08:57View SolutionMatch the following :
 .01:40View Solution
.01:40View SolutionParallelogram on equal bases and between the same parallels are equal in area.
05:50View SolutionIn Fig.
 ABCD is a parallelogram , `AE _|_ DC` and `CF _|_ AD`. If AB = 16 cm, AE = 8 cm and CF = 10 cm, find AD.05:43View Solution
ABCD is a parallelogram , `AE _|_ DC` and `CF _|_ AD`. If AB = 16 cm, AE = 8 cm and CF = 10 cm, find AD.05:43View SolutionIf E, F, G and H are respectively the mid-points of the sides of a parallelogram ABCD, show that ar(EFGH)`=1/2`ar(ABCD).
02:51View SolutionP and Q are any two points lying on the sides DC and AD respectively of a parallelogram ABCD. Show that ar (APB) = ar (BQC).
02:00View SolutionIn Fig.
 , P is a point in the interior of a parallelogram ABCD. Show that `ar(APB)+ar(PCD)=1/2ar(ABCD)`.09:29View Solution
, P is a point in the interior of a parallelogram ABCD. Show that `ar(APB)+ar(PCD)=1/2ar(ABCD)`.09:29View SolutionIn Fig.
 , P is a point in the interior of a parallelogram ABCD. Show that `ar(APD)+ar(PBC)=ar(APB)+ar(PCD)`.12:29View Solution
, P is a point in the interior of a parallelogram ABCD. Show that `ar(APD)+ar(PBC)=ar(APB)+ar(PCD)`.12:29View SolutionIn fig.
 PQRS and ABRS are parallelograms and X is any point on side BR. Show that `ar(PQRS)=ar(ABRS)`.03:25View Solution
PQRS and ABRS are parallelograms and X is any point on side BR. Show that `ar(PQRS)=ar(ABRS)`.03:25View SolutionIn fig.
 PQRS and ABRS are parallelograms and X is any point on side BR. Show that `ar(AXS)=1/2ar(PQRS)`.04:49View Solution
PQRS and ABRS are parallelograms and X is any point on side BR. Show that `ar(AXS)=1/2ar(PQRS)`.04:49View SolutionA farmer was having a field in the form of a parallelogram PQRS. She took any point A on RS and joined it to points P and Q.In how many parts the field is divided ? What are the shapes of these parts ? The farmer wants to sow wheat and pulses in equal portions of the field separately. How should she do it ?
06:10View SolutionIn `||gm` ABCD, AB = 14 cm. The altitude corresponding to sides AB and AD are 8 cm and 7 cm. Find AD.
03:05View SolutionFind x+y+z
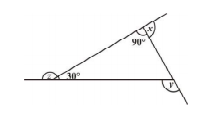 02:30View Solution
02:30View SolutionMatch the following :
 .01:40View Solution
.01:40View SolutionMatch the following :
 .01:40View Solution
.01:40View SolutionGiven figure
 is a parallelogram. P is a point on DC such that ar (`DeltaAPD) = 24 cm^2` and ar `(DeltaBPC) = 16 cm^2`. Find `CP : PD`.08:03View Solution
is a parallelogram. P is a point on DC such that ar (`DeltaAPD) = 24 cm^2` and ar `(DeltaBPC) = 16 cm^2`. Find `CP : PD`.08:03View SolutionMatch the following :
 .01:40View Solution
.01:40View SolutionMatch the following :
 .01:40View Solution
.01:40View SolutionFind x+y+z
 02:30View Solution
02:30View SolutionFind x+y+z
 02:30View Solution
02:30View SolutionIn the given figure
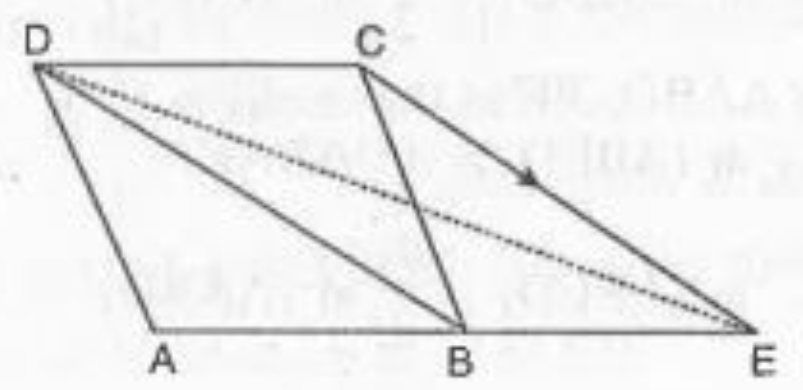 `CE || DB`. If ar `(DeltaDBE) = 36 cm^2` and ar `(DeltaADB) = 44 cm^2` find ar `(DeltaDAE)`.02:36View Solution
`CE || DB`. If ar `(DeltaDBE) = 36 cm^2` and ar `(DeltaADB) = 44 cm^2` find ar `(DeltaDAE)`.02:36View SolutionABCD is parallelogram. X and Y are the mid-points of BC and CD respectively. Prove that ar `(DeltaAXY) =3/8` ar `(||)^(gm) ABCD`.
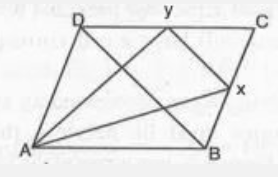 04:21View Solution
04:21View SolutionIn Fig.
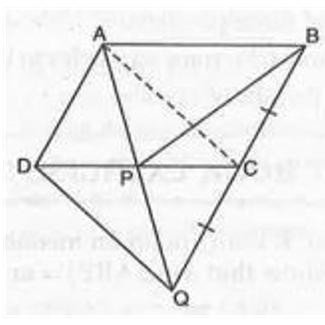
ABCD is a parallelogram. Prove that ar `(DeltaACP)` = ar `(DeltaDPQ)`.01:35View SolutionIn Fig.
 , ABCD is a parallelogram and EFCD is a rectangle. Also `AL _|_ DC`. Prove that : ar `(ABCD) = DCxxAL`.01:05View Solution
, ABCD is a parallelogram and EFCD is a rectangle. Also `AL _|_ DC`. Prove that : ar `(ABCD) = DCxxAL`.01:05View SolutionIn Fig. 9.13, ABCD is a parallelogram and EFCD is a rectangle. Also, `AL bot DC`. Prove that (i) ar (ABCD) = ar (EFCD) (ii) ar (ABCD) = DC × AL
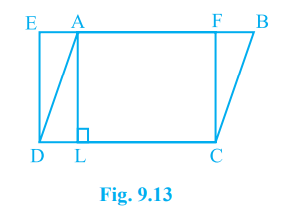 05:11View Solution
05:11View SolutionIn Fig.
 diagonals AC and BD of quadrilateral ABCD intersect at O such that OB = OD. If AB = CD, then show that : ar (DCB)=ar (ACB).03:48View Solution
diagonals AC and BD of quadrilateral ABCD intersect at O such that OB = OD. If AB = CD, then show that : ar (DCB)=ar (ACB).03:48View SolutionIn Fig.
 diagonals AC and BD of quadrilateral ABCD intersect at O such that OB = OD. If AB = CD, then show that : `DA||CB` or ABCD is a parallelogram.05:38View Solution
diagonals AC and BD of quadrilateral ABCD intersect at O such that OB = OD. If AB = CD, then show that : `DA||CB` or ABCD is a parallelogram.05:38View SolutionDiagonals AC and BD of a quadrilateral ABCD intersect at O in such a way that ar (AOD) = ar (BOC). Prove that ABCD is a trapezium.
05:04View SolutionD and E are points on sides AB and AC respectively of `DeltaABC` such that ar (DBC) = ar (EBC). Prove that `DE II BC`.
03:00View SolutionXY is a line parallel to side BC of triangle ABC. If `BE II AC` and `CF II AB` meet XY at E and F respectively,show that ar (ABE) = ar (ACF).
07:38View SolutionFind x+y+z
 02:30View Solution
02:30View SolutionDiagonals AC and BD of a quadrilateral ABCD intersect at O in such a way that ar (AOD) = ar (BOC). Prove that ABCD is a trapezium.
05:04View SolutionFind x+y+z
 02:30View Solution
02:30View SolutionD and E are points on sides AB and AC respectively of a `DeltaABC` such that ar `(DeltaBCE) = ar (DeltaBCD)`. Show that `DE || BC`.
02:42View SolutionDetermine a triangle equal in area to a given quadrilateral.
07:13View SolutionABCD is a parallelogram whose diagonals intersect at E. AC is produced to F such that CF = AE Prove that ar `(DeltaBDF)` = ar `(|| gm ABCD)`.
04:51View SolutionFind x+y+z
 02:30View Solution
02:30View SolutionParallelogram ABCD and rectangle ABEF are on the same base AB and have equal areas. Show that the perimeter of the parallelogram is greater than that of the rectangle.
07:43View Solution
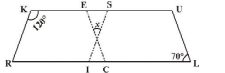 In the above figure both RISK and CLUE are parallelograms. Find the value of x.03:32View Solution
In the above figure both RISK and CLUE are parallelograms. Find the value of x.03:32View SolutionFind x+y+z
 02:30View Solution
02:30View SolutionIn fig., find tan P - cot R.
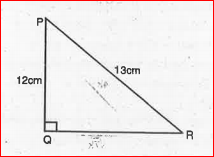 02:18View Solution
02:18View SolutionIn Fig.
 , ABC and BDE are two equilateral triangles such that D is the midpoint of BC. If AE intersects BC at F, show that : ar (BDE)=`1/2`ar(BAE).04:03View Solution
, ABC and BDE are two equilateral triangles such that D is the midpoint of BC. If AE intersects BC at F, show that : ar (BDE)=`1/2`ar(BAE).04:03View SolutionIn Fig.
 , ABC and BDE are two equilateral triangles such that D is the midpoint of BC. If AE intersects BC at F, show that : ar(BFE)=ar(AFD).02:31View Solution
, ABC and BDE are two equilateral triangles such that D is the midpoint of BC. If AE intersects BC at F, show that : ar(BFE)=ar(AFD).02:31View SolutionIn Fig.
 , ABC and BDE are two equilateral triangles such that D is the midpoint of BC. If AE intersects BC at F, show that : ar(BFE)=2ar(FED).07:42View Solution
, ABC and BDE are two equilateral triangles such that D is the midpoint of BC. If AE intersects BC at F, show that : ar(BFE)=2ar(FED).07:42View SolutionIn Fig.
 , ABC and BDE are two equilateral triangles such that D is the midpoint of BC. If AE intersects BC at F, show that : ar(BFE)=2ar(FED).07:42View Solution
, ABC and BDE are two equilateral triangles such that D is the midpoint of BC. If AE intersects BC at F, show that : ar(BFE)=2ar(FED).07:42View SolutionIn Fig.
 , ABC and BDE are two equilateral triangles such that D is the midpoint of BC. If AE intersects BC at F, show that : ar(FED)=`1/8` ar(AFC).07:02View Solution
, ABC and BDE are two equilateral triangles such that D is the midpoint of BC. If AE intersects BC at F, show that : ar(FED)=`1/8` ar(AFC).07:02View SolutionABC and BDE are two equilateral triangles such that D is the mid-point of BC. Then ar (BDE)=`1/4` ar(ABC).
03:07View SolutionDiagonals AC and BD of quadrilateral ABCD intersect each other at P. Show that ar `(APB) xx ar (CPD)` = ar `(APD)xx ar(BPC)`.
08:33View SolutionP and Q are respectively the midpoints of sides AB and BC or a triangle ABC and R is the mid-point of AP, show ar(PRQ)=`1/2` ar(ARC).
09:36View SolutionP and Q are respectively the midpoints of sides AB and BC or a triangle ABC and R is the mid-point of AP, show ar(RQC) = `3/8` ar(ABC).
08:15View SolutionP and Q are respectively the midpoints of sides AB and BC or a triangle ABC and R is the mid-point of AP, show ar(PBQ)=ar(ARC).
05:13View SolutionIn Fig.
 ABC is a right triangle right angled at A. BCED, ACFG and ABMN are squares on the sides BC, CA and AB respectively. Line segment `AX _|_ DE` meets BC at Y. Show that : `DeltaFCB ~= DeltaACE`.01:39View Solution
ABC is a right triangle right angled at A. BCED, ACFG and ABMN are squares on the sides BC, CA and AB respectively. Line segment `AX _|_ DE` meets BC at Y. Show that : `DeltaFCB ~= DeltaACE`.01:39View SolutionIn Fig.
 ABC is a right triangle right angled at A. BCED, ACFG and ABMN are squares on the sides BC, CA and AB respectively. Line segment `AX _|_ DE` meets BC at Y. Show that : ar(BYXD)=ar(ABMN).04:11View Solution
ABC is a right triangle right angled at A. BCED, ACFG and ABMN are squares on the sides BC, CA and AB respectively. Line segment `AX _|_ DE` meets BC at Y. Show that : ar(BYXD)=ar(ABMN).04:11View SolutionIn Fig.
 ABC is a right triangle right angled at A. BCED, ACFG and ABMN are squares on the sides BC, CA and AB respectively. Line segment `AX _|_ DE` meets BC at Y. Show that : ar(CYXE)=ar(ACFG).04:29View Solution
ABC is a right triangle right angled at A. BCED, ACFG and ABMN are squares on the sides BC, CA and AB respectively. Line segment `AX _|_ DE` meets BC at Y. Show that : ar(CYXE)=ar(ACFG).04:29View SolutionIn Fig.
 ABC is a right triangle right angled at A. BCED, ACFG and ABMN are squares on the sides BC, CA and AB respectively. Line segment `AX _|_ DE` meets BC at Y. Show that : `DeltaMBC ~= DeltaABD`.01:39View Solution
ABC is a right triangle right angled at A. BCED, ACFG and ABMN are squares on the sides BC, CA and AB respectively. Line segment `AX _|_ DE` meets BC at Y. Show that : `DeltaMBC ~= DeltaABD`.01:39View SolutionIn Fig.
 ABC is a right triangle right angled at A. BCED, ACFG and ABMN are squares on the sides BC, CA and AB respectively. Line segment `AX _|_ DE` meets BC at Y. Show that : ar(CYXE)=ar(ACFG).04:29View Solution
ABC is a right triangle right angled at A. BCED, ACFG and ABMN are squares on the sides BC, CA and AB respectively. Line segment `AX _|_ DE` meets BC at Y. Show that : ar(CYXE)=ar(ACFG).04:29View SolutionIn Fig.
 ABC is a right triangle right angled at A. BCED, ACFG and ABMN are squares on the sides BC, CA and AB respectively. Line segment `AX _|_ DE` meets BC at Y. Show that : ar(BCED)=ar(ABMN)+ar(ACFG).02:19View Solution
ABC is a right triangle right angled at A. BCED, ACFG and ABMN are squares on the sides BC, CA and AB respectively. Line segment `AX _|_ DE` meets BC at Y. Show that : ar(BCED)=ar(ABMN)+ar(ACFG).02:19View SolutionIdentify (a),(b) and (c)
 02:13View Solution
02:13View SolutionFind x+y+z
 02:30View Solution
02:30View SolutionIf E, F, G and H are the mid-points of sides of a parallelogram ABCD then ar (EFGH) = ............ .
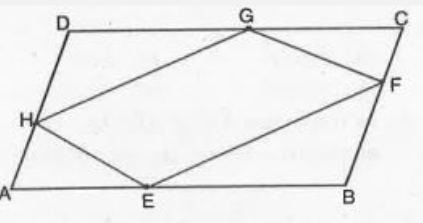 11:42View Solution
11:42View SolutionE is any point on median AD of `DeltaABC`. If ar `(DeltaABE) = 10 cm^2` then ar `(DeltaACE)` is :
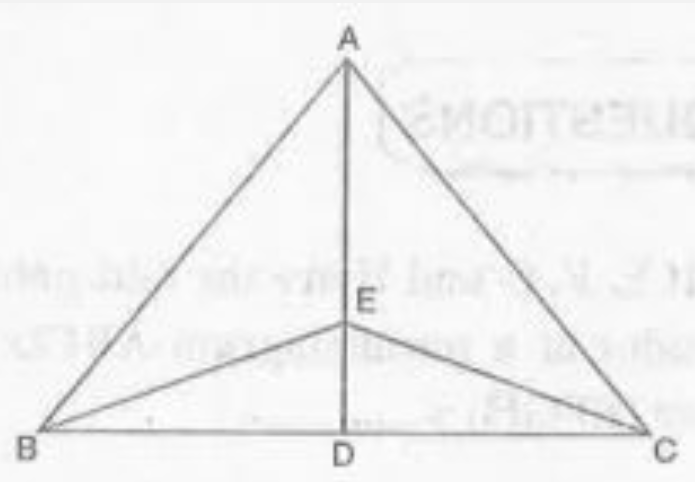 04:22View Solution
04:22View SolutionIn trapezium ABCD `AB||DC`. If ar (AOD) = `15 cm^2` then ar (BOC) is :
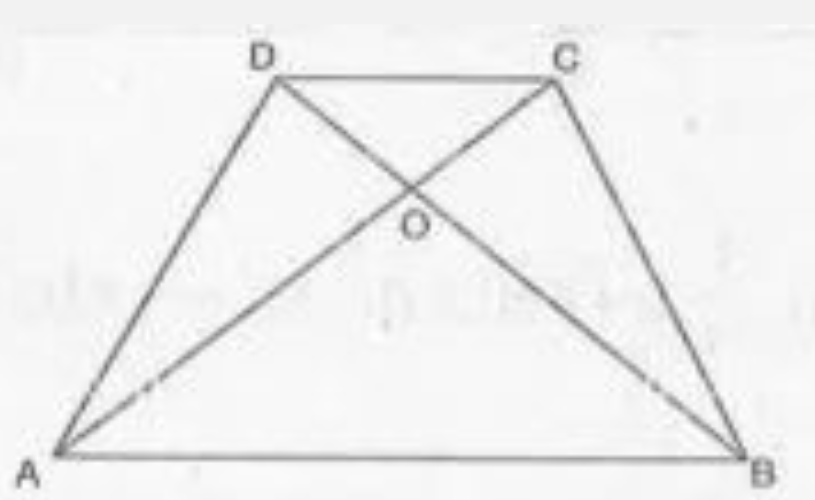 03:15View Solution
03:15View SolutionThe median of a triangle divides it into two
03:17View SolutionIn which of the following figures , you find two polygons on the same base and between the same parallels ?
02:32View SolutionThe figure obtained by joining the midpoints of the adjacent sides of a rectangle of sides 8 cm and 6 cm is :
02:45View SolutionIn Fig.
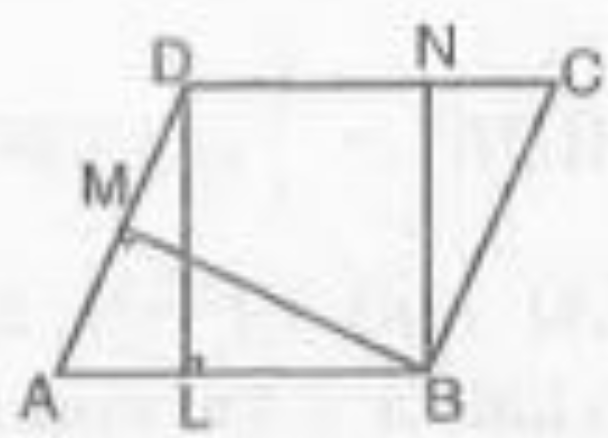
the area of parallelogram ABCD is :01:22View SolutionIn Fig.
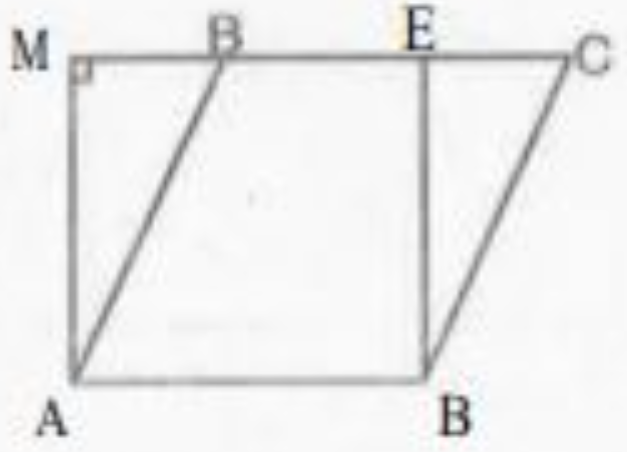
If parallelogram ABCD and rectangle ABEF are of equal area,
then :04:12View SolutionThe mid-point of the sides of a triangle along with any of the vertices as the fourth point make a parallelogram of area equal to
06:25View SolutionTwo parallelograms are on equal bases andbetween the same parallels. The ratio of their areas is
03:29View SolutionABCD is a quadrilateral whose diagonal AC divides it into two parts, equal in area, then ABCD
02:56View SolutionIf a triangle and a parallelogram are on the same base and between same parallels, then the ratio of the area of the triangle to the area of parallelogram is
02:27View SolutionABCD is a trapezium with parallel sides AB = a cm and DC = 6 cm E and F are the mid-points of the non-parallel sides. The ratio of ar (ABFE) and ar (EFCD) is
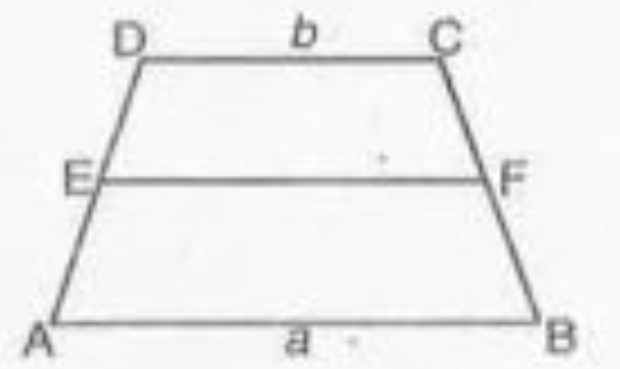 06:10View Solution
06:10View SolutionABCD is a parallelogram and X is the mid-point of AB. If ar (AXCD) = `24 cm^2`, then ar (ABC) = `24 cm^2`.
01:45View SolutionPQRS is a rectangle inscribed in a quadrant of a circle of radius 13 cm. A is any point on PQ. If PS = 5 cm, then ar (PAS) = `30 cm^2`.
04:51View SolutionPQRS is a parallelogram whose area is `180 cm^2` and A is any point on the diagonal QS. The area of `DeltaASR = 90 cm^2`.
03:53View SolutionABC and BDE are two equilateral triangles such that D is the mid-point of BC. Then ar (BDE)=`1/4` ar(ABC).
03:07View SolutionFind x+y+z
 02:30View Solution
02:30View Solution
Exercise questions and complete solutions for chapter Areas of Parallelograms and Triangles of MBD of Class 9
