Complete Solutions to EXAMPLE of chapter QUADRILATERALS of Class 9 book with complete answers and questions
| Class 9 | MATHS |
| Chapter | QUADRILATERALS |
| MODERN PUBLICATION | EXAMPLE |
| Number Of Questions | 100 |
| Academic Year | 2023-24 |
Three angles of a quadrilateral measure `68^@,43^@` and `100^@`. Find the measure of the fourth angle.
View SolutionIn a quadrilateral ABCD, `angleA,angleB,angleC` and `angleD` in the ratio 1:2:2:4. find the measure of each angle of the quadrilateral.
View SolutionIn a quadrilateral ABCD, AO and BO are bisectors of `angleA` and angle B respectively. Prove that
`angleAOB=1/2(angleC+angleD)`
View SolutionIn the fig. ABCD is a quadrilateral in which AB is the largest and CD is the shortest side. Prove that:
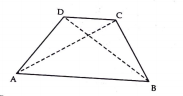
`angleC>angleA`
View SolutionIn the fig. ABCD is a quadrilateral in which AB is the largest and CD is the shortest side. Prove that:
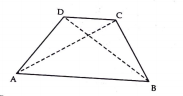
`angleD>angleB`
View SolutionIn the fig. the point O is in the inside of an equilateral quad. ABCD such that OB=OD. Prove that the points A,O and C are collinear
View SolutionIn a ||gm ABCD if `angleA=125^@` find `angleB,angleC` and `angleD`.
View SolutionIn the fig. ABCD is a rhombus if `angleA=66^@` find `angleCDB`
View SolutionIn the fig. ABCD is a square, A line segment DP cuts BP at P and diagonal AC at O such that `angleCOD=110^@` and `angleOPC=x^@`. Find the value of x.
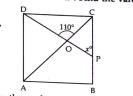
View SolutionABCD is a rhombus such that `/_ACB = 40^@`. Then `/_ADB` is
View SolutionABCD is a square. Determine `angleDCA`.
View SolutionABCD is a rectangle with `angleBCA=34^@` find `angleDBC`.
View SolutionIn the fig. ABCD is a parallelogram compute the values of x and y
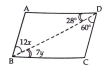
View SolutionIn Fig. find the four angles A, B, C and D in the parallelogram ABCD.
View SolutionThe diagonals of a prallelogram ABCD intersect at O. A line through O intersets AB at P and DC at Q. prove that OP=OQ.
View SolutionABCD is a parallelogram. AB is produced to E, so that BE = AB. Prove that ED bisects BC.
View SolutionABCD is a parallelogram and line segments AX,CY bisects the angles A and C respectively show that AX||CY.
View SolutionABCD is a parallelogram E is the mid point of AB and CE bisects `angleBCD`. Prove that:
AE=AD?
View SolutionABCD is a parallelogram E is the mid point of AB and CE bisects `angleBCD`. Prove that:
DE bisects `angleADC`
View SolutionABCD is a parallelogram E is the mid point of AB and CE bisects `angleBCD`. Prove that:
`angleDEC=90^@`
View SolutionD,E and F are respectively the mid points of the sides BC,CA and AB of an equilateral triangle ABC, prove that DEF is also equilateral triangle.
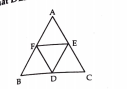
View SolutionIn the fig. ABC, is an isosceles triangle in which AB=AC. Also D,E and F are mid point of BC, CB and AB respectively. Show that `ADbotEF` and AD is determined by EF
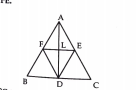
View SolutionIn the fig. AD is a median of triangle ABC and E is the mid point of AD, also BE meets AC in F. prove that `AF=1/3AC`
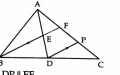
View SolutionIn the fig D,E and F are the mid points of the sides BC,CA and AB respectively of `triangleABC`. If BE and DF intersect at X while CF and DE ntersect at Y. prove that `XY=1/4BC`
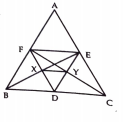
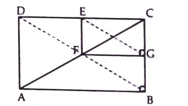
View SolutionIn the fig. ABCD and EFGC are rectangles, where F is the mid point of AC. Prove that:DE=EC
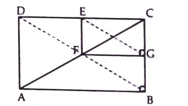
View SolutionIn the fig. ABCD and EFGC are rectangles, where F is the mid point of AC. Prove that:`EG=1/2AC`
View SolutionIn Fig.
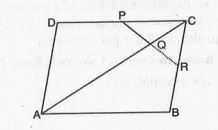 ,
,
ABCD is a parallelogram in which P is the mid-point of DC and Q is a point on AC such that `CQ = 1/4AC`. If PQ produced meets BC at R, prove that R is a mid-point of BC.
View SolutionE and F are the mid points of non-parallel sides AD and BC respectively of a trapezium prove that EF||AB.
View SolutionIn the fig. ABCD is paralllelogram and E is the mid point of AD. A line through D, drawn parallel to EB, meets AB produced at F and BC at L. prove that
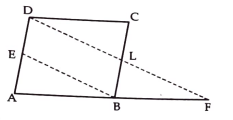
AF=2DC
View SolutionIn the fig. ABCD is paralllelogram and E is the mid point of AD. A line through D, drawn parallel to EB, meets AB produced at F and BC at L. prove that DF=2DL.
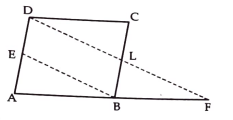
View SolutionShow that the st. Line joining the mid-points of two non-parallel sides of a trapezium is parallel to the bases and is equal to half of the sum of their lengths.
View SolutionWhile studying prperties of triangle, Rakesh, a student of class IX concluded that all four angle of quadrilateral must be acute.
Justify on behalf of Rakesh.
View SolutionWhile studying prperties of triangle, Rakesh, a student of class IX concluded that all four angle of quadrilateral must be acute.
Justify on behalf of Rakesh.
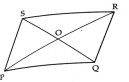
View SolutionPQRS is a rhombus where `anglePSR=120^@`. There are two fire stations R and S and fire is represent at O. Which fire stations team can reach early?
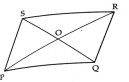
View SolutionPQRS is a rhombus where `anglePSR=120^@`. There are two fire stations R and S and fire is represent at O.Which value is depicted?
View SolutionIn a triangle ABC find the measures of all the angles of the traingle formed by joining the mid points of the sides of this triangle.
View SolutionThe angles of quadrilateral are in the ratio 3 : 5 : 9 : 13. Find all the angles of the quadrilateral.
View SolutionWhich of the following statements are True or False :
If the diagonals of a parallelogram are equal then it is a rectangle.
View SolutionIf diagonals of a quadrilateral bisect each other at right angles, then it is a :
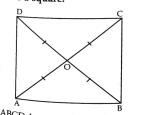
View SolutionShow that the diagonals of a square are equal and bisect each other at right angles
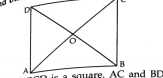
View SolutionShow that if the diagonals of a quadrilateral are qual and bisect each other at right angles, then it is a square
View SolutionDiagonal AC of a parallelogram ABCD bisects `angleA` show that
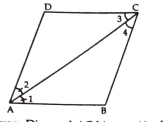
If bisects `angleC` also.
View SolutionDiagonal AC of a parallelogram ABCD bisects `angleA` show that
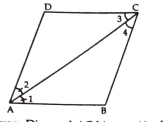
ABCD is a rhombus.
View SolutionABCD is a rhombus. Show that the diagonal AC bisects `/_A` as well as `/_C` and diagonal BD bisects `/_B` as well as `/_D`.
View SolutionABCD is a rectangle in which diagonal AC bisects `/_A as well as `/_C`. Show that ABCD is a square.
View SolutionABCD is a rectangle in which diagonal AC bisects `/_A as well as `/_C`. Show that Diagonal BD bisects both `/_B` as well as `/_D`.
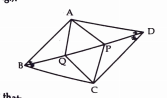
View SolutionIn parallelogram ABCD, two points P and Q are taken on diagonal BD such that DP=BQ
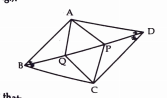
show that: `triangleAPDequivtriangleCQB`
View SolutionIn parallelogram ABCD, two points P and Q are taken on diagonal BD such that DP=BQ
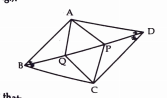
AP=CQ
View SolutionIn parallelogram ABCD, two points P and Q are taken on diagonal BD such that DP=BQ
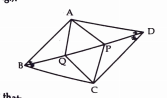
Show that: `triangleAQBequivtriangleCPD`
View SolutionIn parallelogram ABCD, two points P and Q are taken on diagonal BD such that DP=BQ
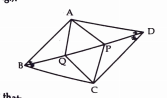
Show that:AQ=CP
View SolutionIn parallelogram ABCD, two points P and Q are taken on diagonal BD such that DP=BQ. Show that APCQ is a parallelogram.
View SolutionABCD is a parallelogam and AP and CQ are the perpendicualr form vertices A and C on diagonal
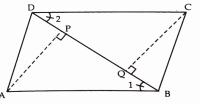
Show that: `triangleAPBequivtriangleCQD`
View SolutionABCD is a parallelogam and AP and CQ are the perpendicualr form vertices A and C on diagonal
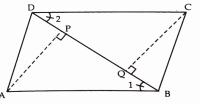
Show that: AP=CQ
View SolutionIn `triangleABC` and `triangleDEF`, AB=DE, AB||DE, BC=EF and BC||EF. Vertices A,B and C are joined to vertices D,E and F respectively
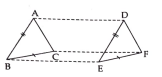
Show that: quadrilateral ABED is a parallelogram.
View SolutionIn `triangleABC` and `triangleDEF`, AB=DE, AB||DE, BC=EF and BC||EF. Vertices A,B and C are joined to vertices D,E and F respectively
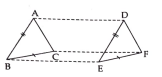
quadrilateral BEFC is a parallelogram.
View SolutionIn `Delta ABC` and `Delta DEF` , AB = DE, `AB|| DE`, BC = EF and `BC|| EF`. Vertices A, B and C are joined to vertices D, E and F respectively (See fig.)
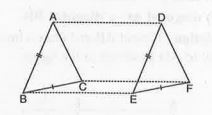 Show that `AD ||CF` and AD=CF.
Show that `AD ||CF` and AD=CF.
View SolutionIn `triangleABC` and `triangleDEF`, AB=DE, AB||DE, BC=EF and BC||EF. Vertices A,B and C are joined to vertices D,E and F respectively
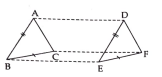
Quadrilateral ACFD is a parallelogram.
View SolutionIn `triangleABC` and `triangleDEF`, AB=DE, AB||DE, BC=EF and BC||EF. Vertices A,B and C are joined to vertices D,E and F respectively
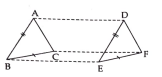
AC=DF
View SolutionIn `triangleABC` and `triangleDEF`, AB=DE, AB||DE, BC=EF and BC||EF. Vertices A,B and C are joined to vertices D,E and F respectively
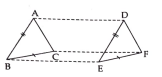
`triangleABCequivtriangleDEF`
View SolutionABCD s a trapezium in which AB||CD and AD=BC
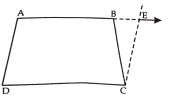
Show that: `angleA=angleB`
View SolutionABCD s a trapezium in which AB||CD and AD=BC
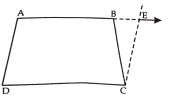
`angleC=angleD`
View SolutionABCD s a trapezium in which AB||CD and AD=BC then show that
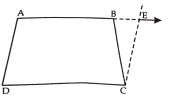
`triangleABCequivtriangleBAD`
View SolutionABCD s a trapezium in which AB||CD and AD=BC
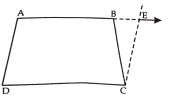
diagonal AC=diagonal BD
View SolutionABCD is a quadrilateral in which P,Q,R and S are the mid points of sides AB,BC,CD and DA respectively AC is a diagonal
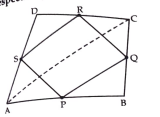
show that: SR||AC and `SR=1/2AC`
View SolutionABCD is a quadrilateral in which P,Q,R and S are the mid points of sides AB,BC,CD and DA respectively AC is a diagonal
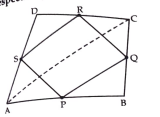
show that: PQ=SR
View SolutionABCD is a quadrilateral in which P,Q,R and S are the mid points of sides AB,BC,CD and DA respectively AC is a diagonal
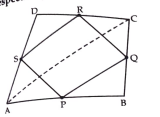
show that: PQRS is a parallelogram.
View SolutionABCD is a rhombus and P, Q, R, S are the mid-points of AB, BC, CD and DA respectively. Prove that quadrilateral PQRS is a rectangle.
View SolutionABCD is a rectangle and P, Q, R and S are the mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rhombus.
View SolutionABCD is a trapezium, in which AB||DC are a diagonal and E is the mid point of AD. A is drawn through E, parallel to AB intersect BC at F. Show that F is the mid point of BC
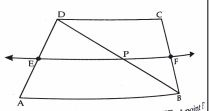
View SolutionIn a parallelogram ABCD, E and F are the mid points of sides AB and CD respectively show that the line segments AF and EC trisect the diagonal BD
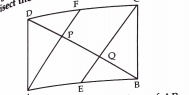
View SolutionShow that the line segments joining the mid-points of opposite sides of a quadrilateral bisect each other.
View SolutionABC is a triangle right angled at C. A line through the mid-point M of hypotenuse AB and parallel to BC intersects AC at D. Show that D is the mid-point of AC.
View SolutionABC is a triangle right angled at C. A line through the mid-point M of hypotenuse AB and parallel to BC intersects AC at D. Show that D is the mid-point of AC.
View SolutionABC is a triangle right angled at C. A line through the mid-point M of hypotenuse AB and parallel to BC intersects AC at D. Show that CM = MA = `1/2 AB` .
View SolutionDiagonals AC and BD of a parallelogram ABCD intersect each other at O. if OA=3 cm and OD=2 cm, determine the length of AC and BD.
View SolutionCan the angles `100^@,80^@,70^@` and `95^@` be the angles of a quadrilateral? Why or why not?
View SolutionIn a quadrilateral ABCD, `angleA,angleB,angleC` and `angleD` in the ratio 1:2:2:4. find the measure of each angle of the quadrilateral.
View SolutionAll the angles of a quadrilateral are equal. What special name is given to this quadrilateral?
View SolutionDiagonals of a rectangled are equal are perpendicular. Is this statement true? Give reasons for your answer.
View SolutionCan you all four angles of a quadrilateral obtuse angles? Give reasons for your answer.
View SolutionIf in a triangle AB=a,AC=b and D,E are the mid-points of AB and AC respectively, then DE is equal to
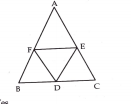
View SolutionIn the given figure, it is given that BDEF and FDCE are aprallelograms. Can you say that BD=CD? Why or why not?
View SolutionIn fig. ABCD and AEFG are two parallelograms. If `angleC=55^@` and determine `angleF`
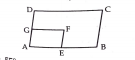
View SolutionCan all the angles of a quadrilateral be acute angles? Give reasonf for you answer.
View SolutionIf one of the angles formed by two intersecting lines is a right angle, what can you say about the other three angles? Give reasonf for your answer.
View SolutionDiagonals of a quadrilateral ABCD bisect each other. If `angleA=35^@` determine `angleB`.
View SolutionOpposite angles of a quadrilateral ABCD are equal. If AB=4 cm determine CD.
View SolutionOne angle of a quadrilateral is of `108^@` and the remaining three angles are equal. Find each of the three equal angles.
View SolutionABCD is a trapezium in which AB||DC and `angleA=angleB=45^@`. Find angled C and D of the trapezium.
View SolutionThe angle betweeen two altitudes of a parallelogram through the vertex of an obtuse angle of parallelogram is `60^@`. Find the angles of the parallelogram.
View SolutionIf the length of the side of a rhombus is equal to the length of one diagonal Find the angles of the rhombus .
View SolutionE and F are points on diagonals AC of a parallelogram ABCD such that AE=CF. show that BFC parallelogram
View SolutionABCD is a trapezium, in which AB||DC are a diagonal and E is the mid point of AD. A is drawn through E, parallel to AB intersect BC at F. Show that F is the mid point of BC

View SolutionThrough A,B and C lines RQ,PR and QP have been drawn, respectively parallel to sides BC, CA and AB of a `triangleABC` as shown in fig. show that `BC=1/2QR`
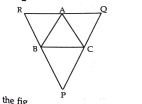
View SolutionD,E and F are respectively the mid points of the sides BC,CA and AB of an equilateral triangle ABC, prove that DEF is also equilateral triangle.

View SolutionPoints P and Q have been taken on opposite sides AB and CD. Respectively of a aprallelogram ABCD such that AP=CQ. Show that AC and PQ bisect each other
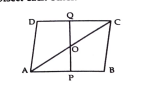
View SolutionIn fig. P is the mid point of side BC of a parallelogram ABCD such that `angleBAP=angleDAP` prove that AD=2CD
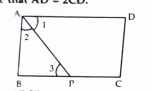
View SolutionA square is inscribed in an isosceles right triangle so that the square and the triangled on angle common. Show that the vertex of a square opposite in vertex of the common angle bisects the hypotenuse.
View SolutionIn a parallelogra ABCD, AB=10 cm and AD=6 cm. The bisectors of `angleA` meets DC in an AE and BC produced at F. find the length of CF.
View SolutionABCD is a rectangle and P, Q, R and S are the mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rhombus.
View Solution
EXAMPLE questions and complete solutions for chapter QUADRILATERALS of MODERN PUBLICATION of Class 9