Complete Solutions to EXERCISE (Subjective Problems) (Long Answer Type) of chapter QUADRILATERALS of Class 9 book with complete answers and questions
| Class 9 | MATHS |
| Chapter | QUADRILATERALS |
| MTG IIT JEE FOUNDATION | EXERCISE (Subjective Problems) (Long Answer Type) |
| Number Of Questions | 5 |
| Academic Year | 2023-24 |
In a parallelogram `A B C D ,` the bisector of `/_A` also bisects `B C` at `Xdot` prove that `A D=2A Bdot`
View Solution`A B C D` is a parallelogram. `A D` is a produced to `E` so that `D E=D C` and `E C` produced meets `A B` produced in `Fdot` Prove that `B F=B Cdot`
View SolutionABCD and APCR are the two parallelograms and AC is the common diagonal. Prove that PBRD is a parallelogram.
View SolutionIn the adjoining figure, ABCD is a paralogram. The side AB is produced to P such that AB=BP and the side AD is produced to Q such that AD = DQ. Prove that CP = CQ.
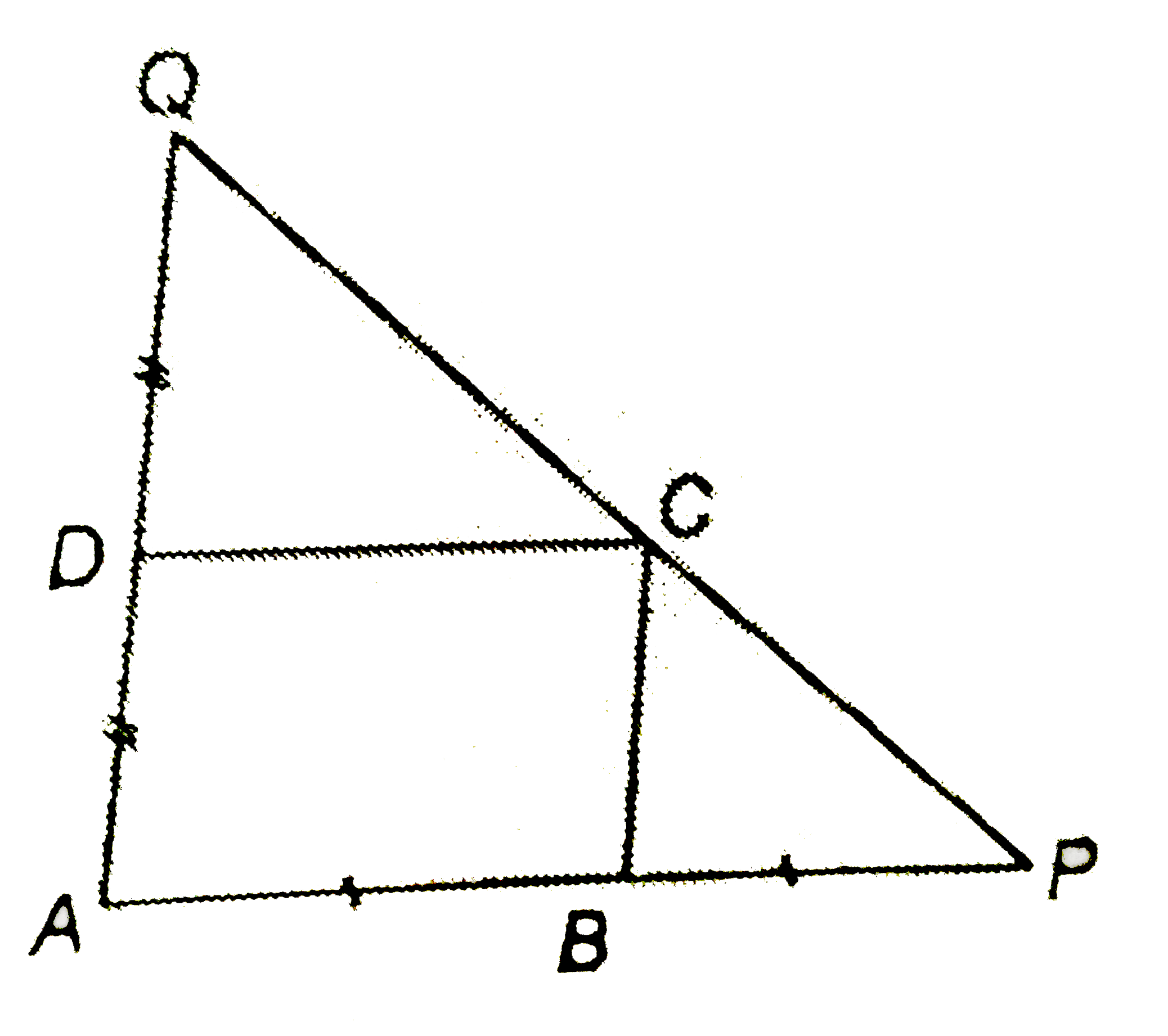
View SolutionIn the adjoining figure, ABCD is a parallelogram and E is the midpoint of AD. A line through D, drawn parallel to EB, meets AB produced at F and BC at L. Prove that (i) `AF=2DC`, (ii) `DF=2DL`
View Solution
EXERCISE (Subjective Problems) (Long Answer Type) questions and complete solutions for chapter QUADRILATERALS of MTG IIT JEE FOUNDATION of Class 9
All Exercises from MTG IIT JEE FOUNDATION Class 9 MATHS QUADRILATERALS
- EXERCISE (Assertion & Reason Type)
- EXERCISE (Comprehension Type PASSAGE- III )
- EXERCISE (Comprehension Type PASSAGE-I)
- EXERCISE (Comprehension Type PASSAGE-II )
- EXERCISE (Integer/Numerical Value Type)
- EXERCISE (Match the Following)
- EXERCISE (Multiple Choice Questions) (LEVEL-1 )
- EXERCISE (Multiple Choice Questions) (LEVEL-2 )
- EXERCISE (Subjective Problems) (Long Answer Type)
- EXERCISE (Subjective Problems) (Short Answer Type)
- EXERCISE (Subjective Problems) (Very Short Answer Type)
- ILLUSTRATIONS
- NCERT SECTION (Exercise 8.1 )
- NCERT SECTION (Exercise 8.2 )
- Olympiad/HOTS CORNER
- SOLVED EXAMPLES