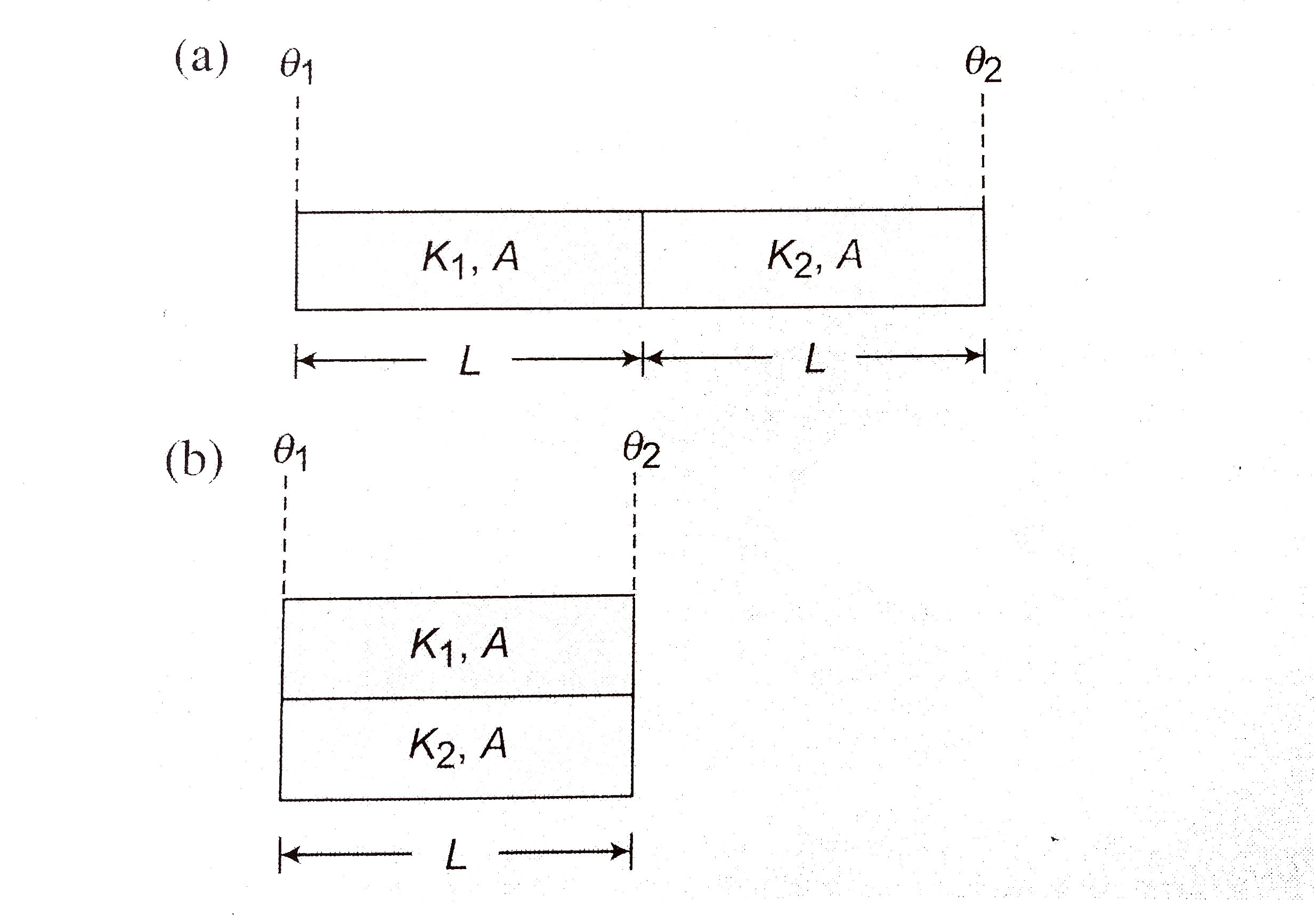Example from CP SINGH PHYSICS Chapter 7 HEAT TRANSFER for Class 11
| Class 11 | PHYSICS |
| Chapter | HEAT TRANSFER |
| CP SINGH PHYSICS | Example |
| Medium | English |
| Academic Year | 2023-2024 |
Question 2
(a) One end of a steel rod (K=46J/m−s−.∘C) length 1.0m is kept in ice at 0C and the end is kept in boling water at 100∘C. The area of cross-section of the rod is 0.4cm2. Assuming no heat loss to atmosphere, find the mass of of the ice melting per second. Latent heat of fusion of ice =3.36×105J/kg
(b) An icebox almost completely filled with ice at 0∘C is dipped into a large volume of water at 20∘C. The box has walls of surface area 2400cm2, thickness 2.0mm and thermal conductivity 0.6W/m−∘C. Calculate the rate at which the ice melts in the box. Latent heat of fusion if ice =3.4×105J/kg.View SolutionQuestion 3
Water is boiled in a container having a bottom of surface area 25cm2 , thickness 1.0mm and thermal conductivity 50Wm−1.∘C−1 , 100g of water is converted into steam per minute in the steady state after the boiling starts.Assuming that no heat is lost to the atmosphere, calculate the temperature of the lower surface of the bottom. Latent heat of vaporization of water =2.26×106Jkg−1 .View SolutionQuestion 9
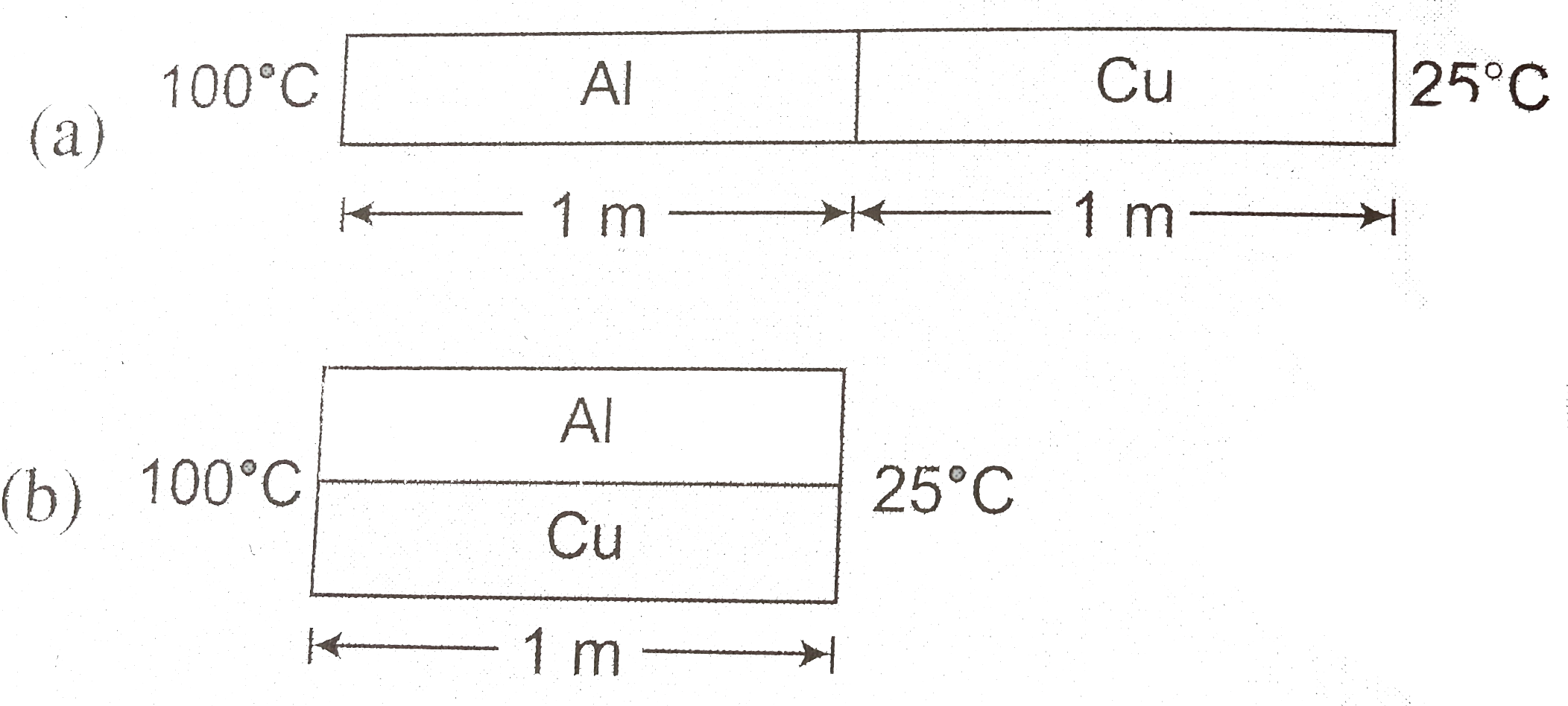
Figure shows an aluminium rod joined to a copper rod. Each of the rods has a length of 20cm and area of cross section 0.20cm2 . The junction is maintained at a constant temperature 40∘C and the two ends are maintained at 80∘C . Calculate the amount of heat taken out from the cold junction in one minute after the steady state is reached. The conductivities are KAl=200Wm−1.∘C−1 . and KCu=400Wm−1.∘C−1 .
View SolutionQuestion 10
(a) Three rod of lengths 20cm each and area of cross-section 1cm2 are joined to form a triangle ABC. The conductivities of the rods are KAB=50J/m−s−.∘C,KBC=200J/m−s−.∘C and KAC=400J/m−s−.∘C. The junctions A,B and C are maintained at 40∘C, 80∘C and 80∘C respectively. Find the rate of heat flowing through the rods AB,AC and BC.
(b) A semicircular rod is joineted as its end to a straight rod of the same material and the same cross-sectional area. The straight rod forms a diameter of the other rod. The junctions are maintained at different temperatures. Find the ratio of the heat transferred through a cross-section of the simicircular rod to the heat transferred through a cross-section of the straight rod in a given time.View SolutionQuestion 11
A room has a window fitted with a single 0.1m×2.0m glass of thickness 2mm. (a) Calculate the rate of heat flow through the closed window when the temperature inside the room is 32∘C and that outside is 40∘C . (b) The glass is now replaced by two glasspane, each having a thickness of 1mm and separated by a distance of 1mm. Calculate the rate of heat flow under the same condition of temperature. Thermal conductivity of window glass =1.0Js−1m−1.∘C−1 and that of air =0.025Js−1m−1.∘C−1 .View SolutionQuestion 12
Three identical rods are joined in series as shown. Their thermal conductivities are K,K/2 and K/3 respectively. At steady, if the free ends of rods are at 100∘C and 20∘C respectively. Determine the temperature at two junction points. Also find the equivalent thermal conductivity.
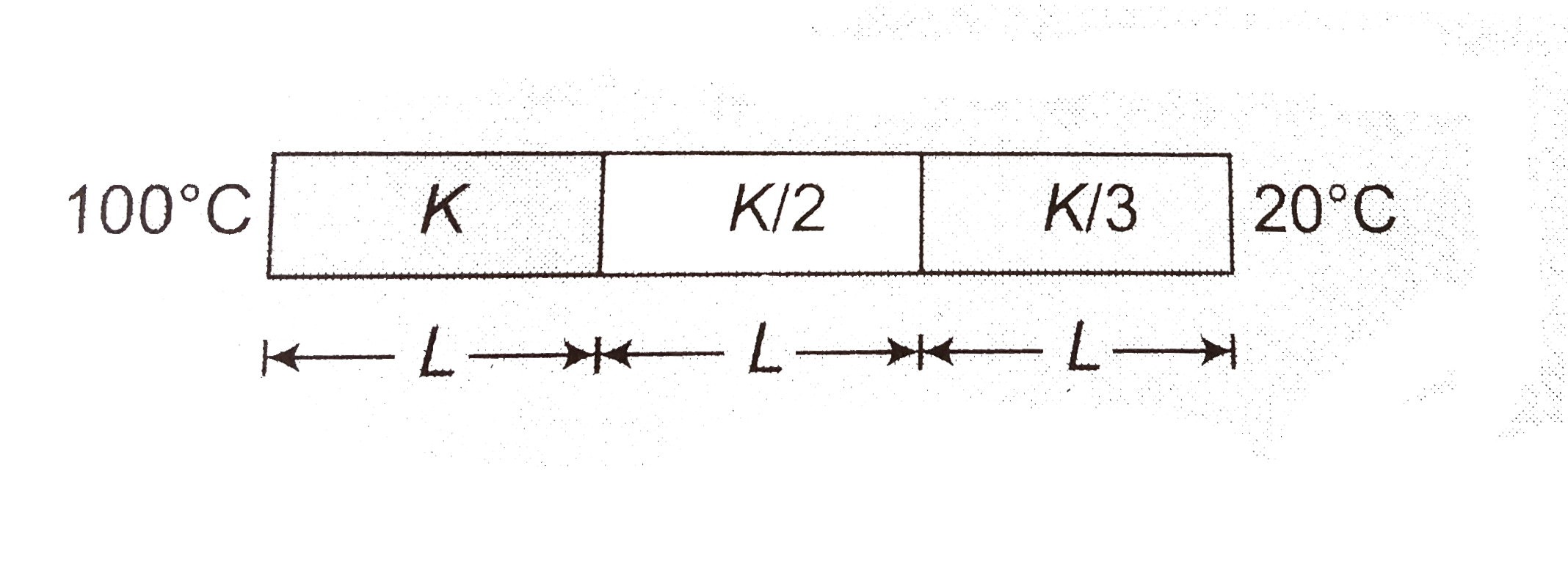View SolutionQuestion 14
A rod of negligible heat capacity has length L, area of cross-section A and thermal conductivity K. The temperature of one end is maintained at 0∘C and the other end is slowly linearly varied from 0∘C to θ∘0C in time t0 Assuming no loss of heat through the sides, find the total heat transimitted through the rod in time t0View SolutionQuestion 15
On a winter day when the atmospheric temperature drops to −10∘C, ice form on the surface of a lake.
(a) Calculate the rate of increases of thickness of the ice when 10cm of ice is already formed.
(b) Calculate the total time taken in forming 10cm of ice. Assume that the temperature of the entire water reaches 0∘C before the ice starts froming. Density of water =1000kg/m3, latent heat of fusion of ice =3.36×105J/kg and thermal conductivity of ice =1.7W/m−∘C. Neglect the expansion of water on freezingView SolutionQuestion 16
Consider the situation of the previous problem. Assume that the temperature of the water at the botton of the lake remains constant at 4∘C as the ice forms on the surface (the heat required to maintain the temperature of the bottom layer may come from the bed of the lake). The depth of the lake is 1.0m. Show that the thickness of the ice formed attains a steady state maximum value. Find this value. The thermal conductivity of water =0.50Wm−1.∘C−1 . Take other relevent data form the previous problelm.View SolutionQuestion 18
A spherical tungsten piece of radius 1√πcm is suspended in an evacuated chamber maintained at 200K. The piece is maintained at 400K by heating it electrically. Find the rate at which the electrial energy must be supplied. The emissivity of tungsten is 0.50, the Steafan constant σ is 6.0×10−8W/m2−K4View SolutionQuestion 19
A cubical block of mass 1.0kg and edge 5.0cm is heated to 227∘C . It is kept in an evacuated chamber maintained at 27∘C . Assming that the block emits radiation like a blackbody, find the rate at which the temperature of the block will decreases. Specific heat capacity of the material of the block is 400Jkg−1K−1 .View SolutionQuestion 20
A solid aluminium sphere and a solid copper sphere of twice the radius are heated to the same temperature and are allowed to cool under identical surrounding temperature. Assume that the emissivity of both the spheres is the same. Find the ratio of
(a) the rate of heat from the aluminium sphere to the rate of heat loss from the copper sphere and
(b) the rate of fall of temperature of the the aluminium sphere to the rate of fall temperature of teh copper sphere. The specific heat capacity of aluminium =900J/kg−∘C and that of copper =390J/kg−∘C. The density of copper is 3.4 times the density of aluminium.View SolutionQuestion 21
One end of a rod length 20cm is inserted in a furnace at 800K. The sides of the rod are covered with an insulating material and the other end emits radiation like a blackbody. The temperature of this end is 750K in the steady state. The temperature of the surrounding air is 300K. Assuming radiation to be the only important mode of energy transfer between the surrounding and the open end of the rod, find the thermal conductivity of the rod. Stefan constant σ=6.0×10−1Wm−2K−4 .View SolutionQuestion 26
A metal ball of mass 1kg is heated by means of a 20W heater in a room at 20∘C . The temperature of the ball becomes steady at 50∘C . (a) Find the rate of loss of heat to the surrounding when the ball is at 50∘C . (b) Assuming Newton's law of cooling, calculate the rate of loss of heat to the surrounding when the ball is at 30∘C . (c) Assume that the temperature of the ball rises uniformly from 20∘C to 30∘C in 5 minutes. Find the total loss of heat to the surrounding during this period. (d) Calculate the specific heat capacity of the metal.View SolutionQuestion 27
A hot body placed in a surrounding of temperature θ0 obeys Newtons's law of cooling dθ/dt=−k(θ−θ0). Its temperature at t=0 is θ1. The specific heat capacity of the body is s and its mass ism.
Find
(a) the maximum heat that the body can lose and
(b) the time starting from t=0 in which it will lose 90% of this maximum heat.View SolutionQuestion 28
Two bodies A and B have thermal emissivities of 0.01 and 0.081 respectively. The outer surface areas of the two bodies are same. The two bodies emit total radiant power at the same rate. The wavelength λB corresponding to maximum spectral radiancy in the radiation from B is shifted radiancy in the radiation from A by 1.00μm. If the temperature of A is 5802K, calculate
(a) the temperature of B and
(b) wavelength λBView Solution
