Two simple pendulums each mass m length l are connected by a spring spring constant k unstretched length b as shown in Fig.Derive the equations of motion using the Lagrange formalism.
The system has two degrees of freedom. We choose the generalized coordinates ϕ1 and ϕ2 as shown in the figure. With the kinetic and the potential energy respectively zero level: point 0 and unstressed spring the Lagrangian becomesL = T − VTo set up the Lagrange equationsthe following derivatives are needed:This leads to the equations of motion:lϕ1 + gsin ϕ1 cosϕ2 = 0 mlϕ2 + mg cosϕ1 sinϕ2 + k2lsin ϕ2 − b cosϕ2 = 0
Related Solutions
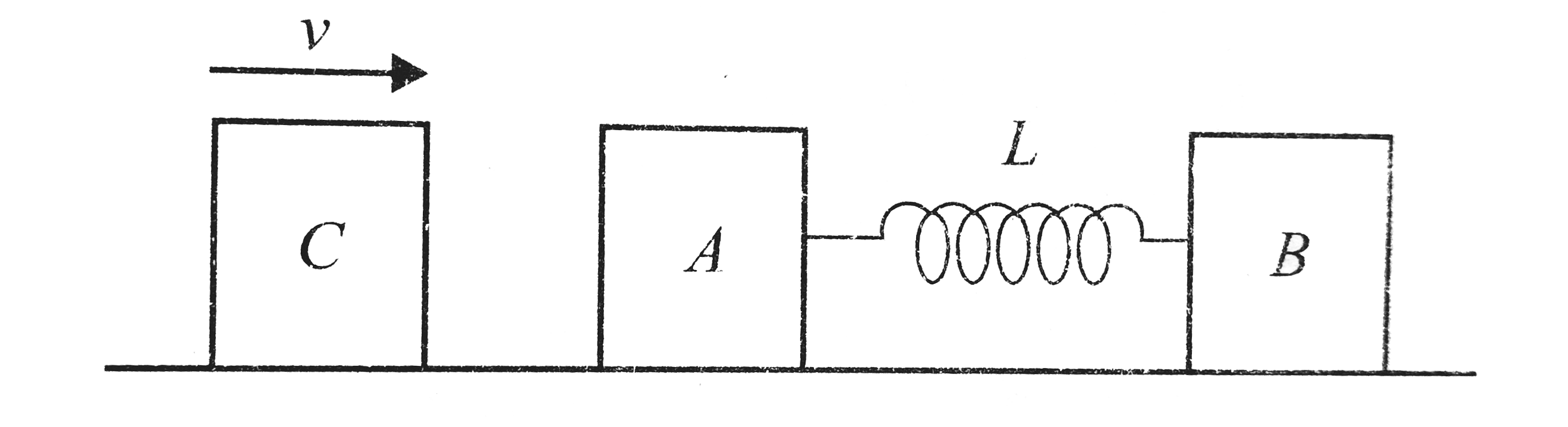
Two identical blocks A and B , each of mass m resting on smooth floor are connected by a light spring of natural length L and spring constant k , with the spring at its natural length. A third identical block C (mass m ) moving with a speed v along the line joining A and B collides with A . The maximum compression in the spring is
View SolutionTwo blocks and . each of mass , are connected by a massless spring of natural length . and spring constant . The blocks are initially resting in a smooth horizontal floor with the spring at its natural length, as shown in Fig. A third identical block , also of mass , moves on the floor with a speed along the line joining and . and collides elastically with . Then
 View Solution
View SolutionWhen a mass M hangs from a spring of length l, it stretches the spring by a distance x. Now the spring is cut in two parts of length l/3 and 2l/3, and the two springs thus formed are connected to a straight rod of mass M which is horizontal in the configuration shown in figure. Find the stretch in each of the spring.
View SolutionWhen a mass M hangs from a spring of length l, it stretches the spring by a distance x. Now the spring is cut in two parts of length l//3"and" 2l//3 , and two springs thus formed are connected to a straight rod of mass M which is horizontal in the configuration shown in figure . Find the stretch in each of the spring
View SolutionWhen a mass M hangs from a spring of length l, it stretches the spring by a distance x. Now the spring is cut in two parts of length l/3 and 2l/3, and the two springs thus formed are connected to a straight rod of mass M which is horizontal in the configuration shown in figure. Find the stretch in each of the spring.
View SolutionTwo identical springs are connected to mass m as shown (k= spring constant) If the period of the configuration in (a) is 2S , the period of the configuration (b) is
View SolutionBlocks A&B of mass m each are connected with spring of constant k both blocks lie on frictionless ground and are imparted horizontal velocity v as shown when spring is unstretched find the maximum stretch of spring.
View SolutionIn the equilibrium conditions shown in the figure all the springs have unstretched length l
View SolutionA horizontal metallic rod of mass m and length l is supported by two vertical identical springs of spring constant k each and natral length i_(0) A current l is flowing in the rod in the direction shown if the rod is in equilibrium then the length of each spring in this state is:
View SolutionA horizontal metallic rod of mass 'm' and length 'l' is supported by two vertical identical springs of spring constant 'k' each and natral length l_(0) A current 'l' is flowing in the rod in the direction shown if the rod is in equilibrium then the length of each spring in this state is:
View SolutionTwo identical blocks A and B, each of mass m resting on a smooth floor are connected by a light spring of natural length Land spring constant K, with the spring in its natural length. A third identical block of mass m moving with a speed v along the line joining Aand B collides with A. The maximum compression in the spring is
View SolutionTwo equal masses are attached to the two ends of a spring of spring constant k. The masses are pulled out symmetrically to stretch the spring by a length x over its natural length. The work done by the spring on each mass is
View SolutionTwo equal masses are attached to the two ends of a spring of spring constant . The masses are pulled a part symmetrically to stretch the spring by a length over its natural length. The work done by the spring on each mass is.
View SolutionTwo equal masses are attached to the two ends of a spring of spring constant . The masses are pulled a part symmetrically to stretch the spring by a length over its natural length. The work done by the spring on each mass is.
View SolutionTwo equal masses are attached to the two ends of a spring of spring constant k. The masses are pulled out symmetrically to stretch the spring by a length x over its natural length. The work done by the spring one each mass is
View SolutionTwo equal masses are attached to the two ends of a spring of spring constant k. The masses are pulled out symmetrically to stretch the spring by a length x over its natural length. The work done by the spring one each mass is
View SolutionA body of mass 20 g connected to a spring of spring constant k, executes simple harmonic motion with a frequency of Hz. The value of spring constant is
View SolutionA body of mass 20 g connected to a spring of spring constant k, executes simple harmonic motion with a frequency of (5//pi) Hz. The value of spring constant is
View SolutionA pendulum of mass m and length L is connected to a spring as shown in figure. If the bob is displaced slightly from its mean position and released, it performs simple harmonic motion. The angular frequency of the oscillation of bob is
View Solution
