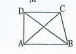
ABCD is quadrilateral. Is AB + BC + CD + DA < 2 AC + BD ?
Since the sum of lengths of any two sides in a triangle should be greater than the length of third sideTherefore In Δ AOB AB < OA + OB ……….i In Δ BOC BC < OB + OC ……….ii In Δ COD CD < OC + OD ……….iii In Δ AOD DA < OD + OA ……….iv ⇒ AB + BC + CD + DA < 2OA + 2OB + 2OC + 2OD ⇒ AB + BC + CD + DA < 2[AO + OC + DO + OB] ⇒ AB + BC + CD + DA < 2AC + BD Hence it is proved.
Related Solutions
ABCD एक चतुर्भुज हैं।क्या AB + BC + CD + DA > 2(AC+BD)?
View Solutionis quadrilateral. Is
View SolutionABCD ਇੱਕ ਚਤੁਰਭੁਜ ਹੈ। ਕੀ ?
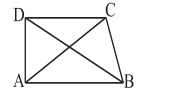
View Solutionएक चतुर्भुज ABCD में सिद्ध कीजिए कि
View SolutionABCD is a quadrilateral. Is AB+BC+CD+DA>AC+BD?
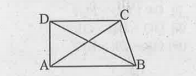
View Solutionदिखाइये कि एक चतुर्भुज ABCD में
View SolutionShow that in a quadrilateral ABCD
View Solutionis a quadrilateral. Is
View SolutionIn a quadrilateral ABCD, show that
.
View SolutionShow that in a quadrilateral ABCD
View SolutionIn a quadrilateral ABCD, show that
View SolutionABCD एक चतुर्भुज है। क्या AB + BC + CD + DA + > AC + BD ?

View SolutionABCD is a quadrilateral. Is ?
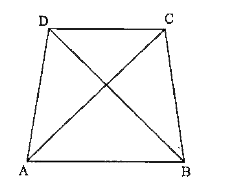
View SolutionABCD is a quadrilateral. Is ?

View SolutionABCD is a quadrilateral whose diagonals AC and BD intersect at O, prove that: (AB+BC+CD+DA)>2(AC+BD)
View Solutionयदि एक चतुर्भुर्ज है जिसके विकर्ण और पर परिच्छेद करते है तो सिद्ध कीजिए की :
(ii)
View SolutionIn the adjoining figure, ABCD is a quadrilateral. Its diagonals AC and BD intersect at point 'O'. Prove that :
(a)
(b)

View SolutionIn the adjoining figure, ABCD is a quadrilateral. Its diagonals AC and BD intersect at point 'O'. Prove that :
(a)
(b)

View SolutionABCD is a quadrilateral whose diagonals AC and BD intersect at O, prove that: (AB+BC+CD+DA)>(AC+BD)
View Solutionयदि ABCD एक चतुर्भुज हों, तो सिद्ध कीजिए कि-
(i) AB + BC + CD + DA > 2AC
(ii) AB + BC + CD + DA > AC + BD
View Solution