ABCD is a quadrilateral. Is AB+BC+CD+DA>AC+BD?
To determine whether AB+BC+CD+DA>AC+BD for quadrilateral ABCD, we can use the properties of triangles formed by the diagonals of the quadrilateral. Here’s a step-by-step solution:
Step 1: Identify the triangles
Consider the diagonals AC and BD of quadrilateral ABCD. We can form four triangles: △ABC, △ADC, △DBC, and △ADB.
Step 2: Apply the triangle inequality
For each triangle, we can apply the triangle inequality which states that the sum of the lengths of any two sides of a triangle must be greater than the length of the third side.
1. For triangle ABC:
AB+BC>AC(Equation 1)
2. For triangle ADC:
AD+DC>AC(Equation 2)
3. For triangle DBC:
DC+BC>BD(Equation 3)
4. For triangle ADB:
AD+AB>BD(Equation 4)
Step 3: Add the inequalities
Now, we will add all four inequalities together:
(AB+BC)+(AD+DC)+(DC+BC)+(AD+AB)>AC+AC+BD+BD
Step 4: Combine like terms
Combining the left side:
2AB+2BC+2AD+2DC>2AC+2BD
Step 5: Simplify the inequality
Dividing both sides by 2 gives:
AB+BC+AD+DC>AC+BD
Conclusion
Thus, we conclude that:
AB+BC+CD+DA>AC+BD
Consider the diagonals AC and BD of quadrilateral ABCD. We can form four triangles: △ABC, △ADC, △DBC, and △ADB.
For each triangle, we can apply the triangle inequality which states that the sum of the lengths of any two sides of a triangle must be greater than the length of the third side.
AB+BC>AC(Equation 1)
AD+DC>AC(Equation 2)
DC+BC>BD(Equation 3)
AD+AB>BD(Equation 4)
Now, we will add all four inequalities together:
(AB+BC)+(AD+DC)+(DC+BC)+(AD+AB)>AC+AC+BD+BD
Combining the left side:
2AB+2BC+2AD+2DC>2AC+2BD
Dividing both sides by 2 gives:
AB+BC+AD+DC>AC+BD
Thus, we conclude that:
AB+BC+CD+DA>AC+BD
Topper's Solved these Questions
- THE TRIANGLE AND ITS PROPERTIESBOOK - NCERT ENGLISHCHAPTER - THE TRIANGLE AND ITS PROPERTIESEXERCISE - EXERCISE 6.5
8 Videos
- THE TRIANGLE AND ITS PROPERTIESBOOK - NCERT ENGLISHCHAPTER - THE TRIANGLE AND ITS PROPERTIESEXERCISE - EXERCISE 6.1
1 Videos
- THE TRIANGLE AND ITS PROPERTIESBOOK - NCERT ENGLISHCHAPTER - THE TRIANGLE AND ITS PROPERTIESEXERCISE - SOLVED EXAMPLES
6 Videos
- SYMMETRYBOOK - NCERT ENGLISHCHAPTER - SYMMETRYEXERCISE - EXERCISE 14.2
2 Videos
Similar Questions
ABCD is quadrilateral. Is AB+BC+CD+DA<2(AC+BD)?
View SolutionABCD is a quadrilateral in which AB=BC and AD=CD ,Show that BD bisects both the ∠ABC and ∠ADC.
View SolutionIf ABCD is a quadrilateral such that AB = AD and CB = CD , then prove that AC is the perpendicular bisector of BD.
View SolutionIn the adjoining figure, ABCD is a quadrilateral. Its diagonals AC and BD intersect at point 'O'. Prove that :
(a) AB+BC+CD+DA<2(AC+BD)
(b) AB+BC+CD+DA>(AC+BD)

View SolutionShow that in a quadrilateral ABCD AB+BC+CD+DA>AC+BD
View SolutionABCD is a quadrilateral in which AD = BC. E, F, G and H are the mid-points of AB, BD, CD and AC respectively. Prove that EFGH is a rhombus.
View SolutionShow that in a quadrilateral ABCD AB+BC+CD+DA<2(BD+AC)
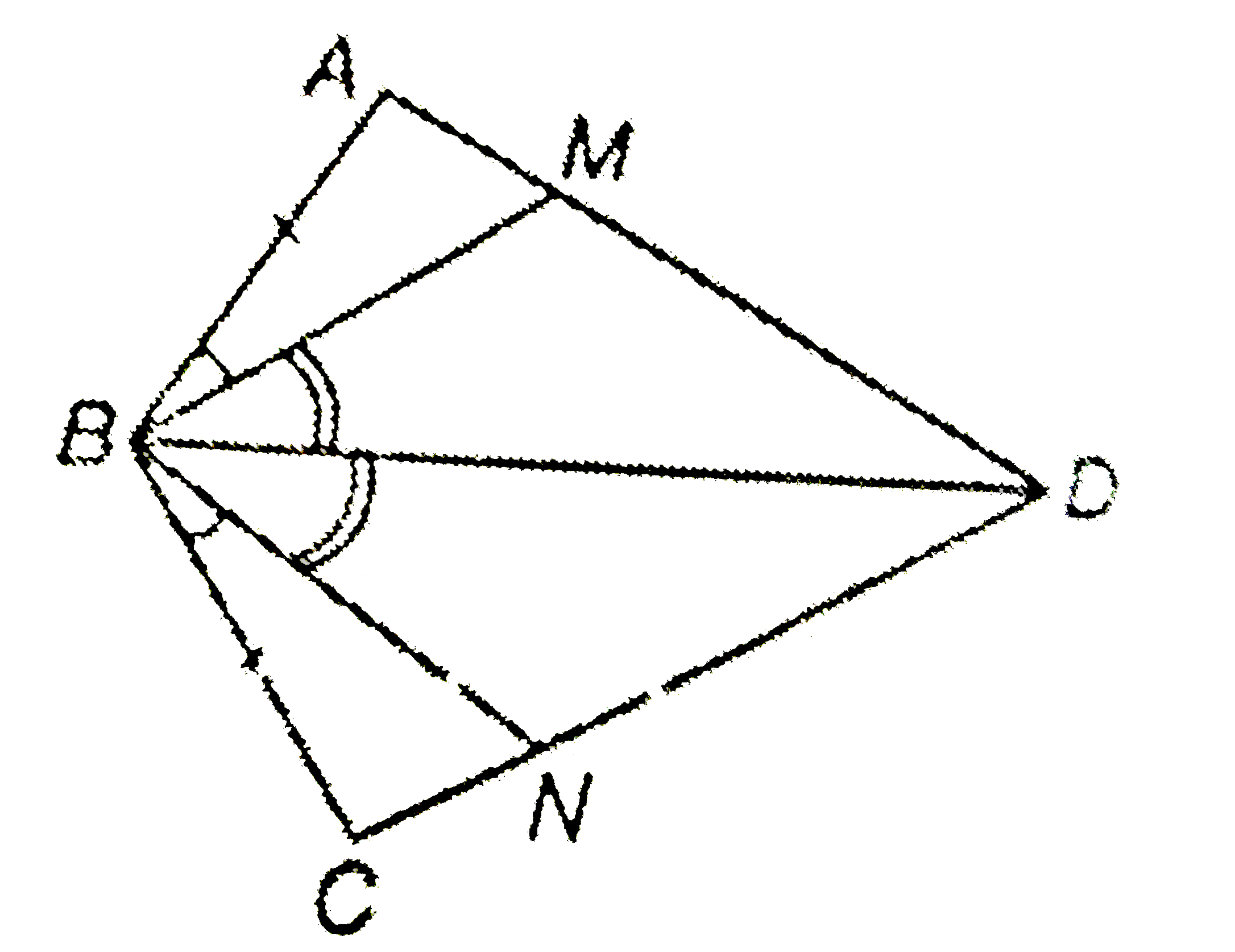
View SolutionIn the adjoining figure, ABCD is a quadrilateral. M and N are the points on AD and CD respectively such that AB=BC,∠ABM=∠CBN and ∠MBD=∠NBD. Prove that BM=BN.
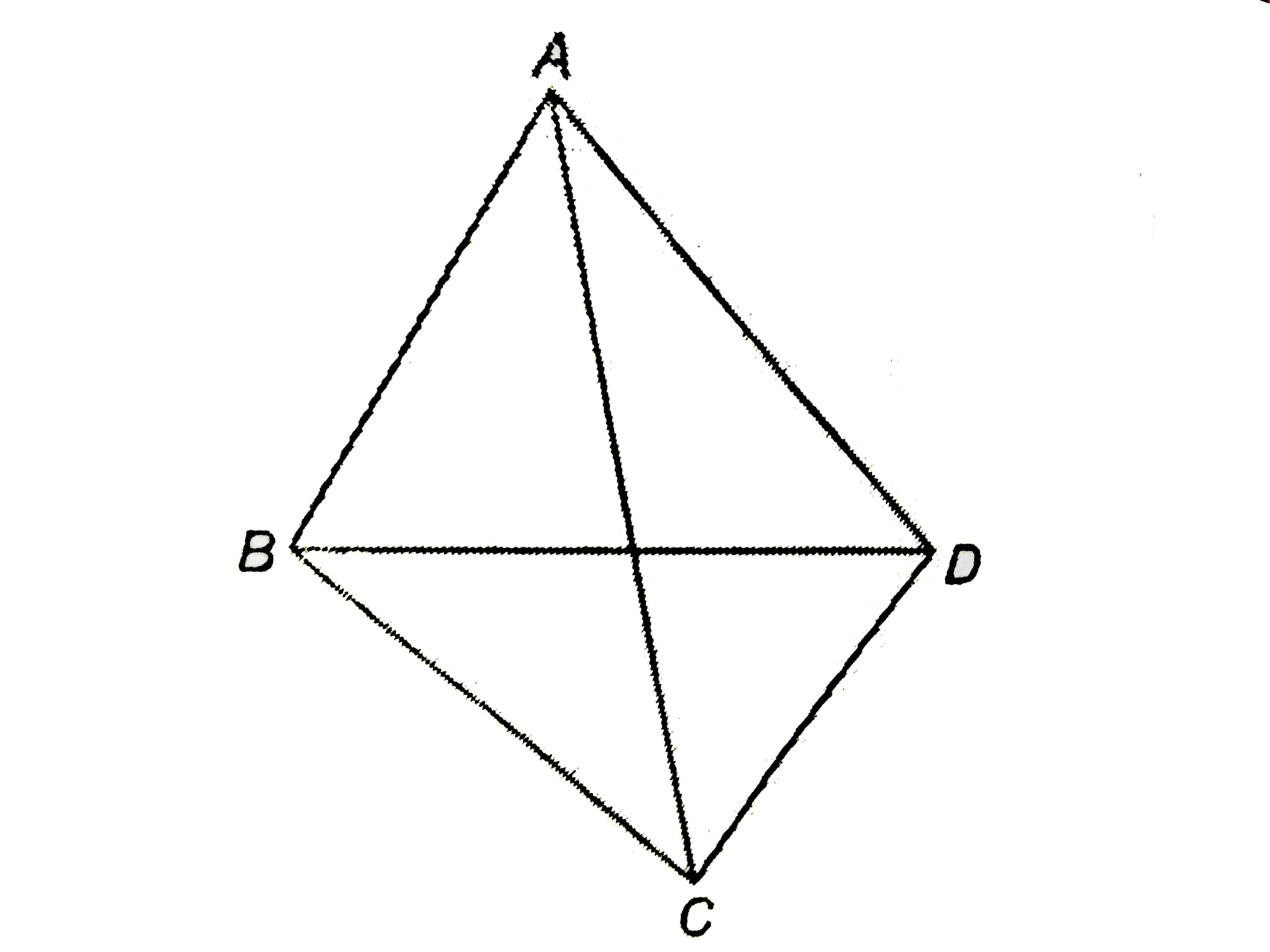
View SolutionThe given figure shows a quadrilateral ABCD. Prove that :
AB+BC+CD+DA>AC+BD
View SolutionABCD is a quadrilateral in which AB=AD . The bisector of BAC and CAD intersect the sides BC and CD at the points E and F respectively. Prove that EF||BD.
View Solution
