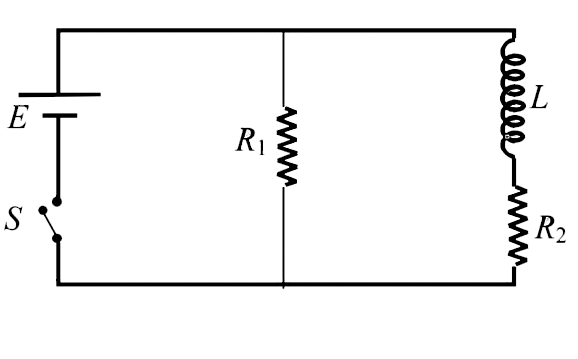
An inductor of inductance L=400 mH and resistor of resistance R1=2(Ω)andR2=2(Ω) are connected to a battery of emf E = 12 Vas shown in the figure. The internal resistance of the battery is negligible. The switch S is closed at time t =0. What is the potential drop across L as a function of time? After the steady state is reached, the switch is opened. What is the direction and the magnitude of current through R1 as a function of time?
The correct Answer is:A, B, C
This is a question on growth and rise of current.
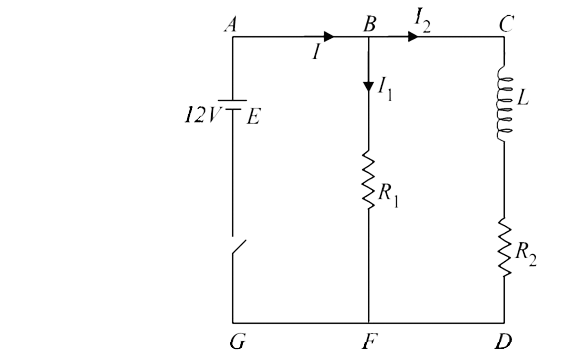
Growth of current: Let at any instant of time t the current be as shown in the figure.
Applying Kirochoff's law in the loop ABCDFGA
we get, starting from G moving clockwise
E−Ldl2dt−(I2)(R2)=0
or (I2)=ER2[1−e−(RL)t]
Also, we know that the emf (V) produced across hte inductor
V=−dϕdt=−ddt[LI2]=−LdI2dt
=-L(d)/(dt)[(E)/(R_2)( 1-e^((-R_(2))/(L)t)]<br>V=-E^((R_2)/(-L)t).Herethe¬ativesignshowstheopposite→thegrowthofcurrent.<br>:. V+12e(2)/(400xx10^(-3))t=12 e^(-5t)volt.<br>DECAYOFCURENT:Whentheswitchisope≠d,thebranchAGisoutofhte∘uitCBFDC(∈clockwisedirection).<br>Apply∈gKirchhoff'slaw<br>I(R_(1)+R_(2))-(-(LdI)/(dt)=0<br>:. (dI)/(I)=-(R_(1)+R_(2))/(L) int_(0)^(t) dt<br>:. I=(I_0)e^(-(R_(1)+R_(2)t)/(L)<br>here,R1+R2L=2+2400×10−3=10
and (I0)=ER1+R2=124=3A
∴I=3e−10tA,clockwise<br>A<ernatively,youmaydirectlyf∈dthetimeconstant<br>(tau)=(l)/(R_(1)+R_(2))andusetheequationi=(i_0)e^(-t//tau)where(I-0)=6A.
Topper's Solved these Questions
Similar Questions
An inductor of inductance L=400mH and resistors of resistances R1=2Ω and R2=2Ω are connected to a battery of emf E=12V as shown in the figure. The internal resistance of the battery is negligible. The switch S is closed at time t=0.

What is the potential dro across L s a function of time? After the steady state is reached, the switch is opened. What is the direction ad the magnitude of current throough R1 as a function of time?
View SolutionAn inductor of inductance L = 400 mH and resistors of resistance R1=2Ω and R2=2Ω are connected to a battery of emf 12 V as shown in the figure. The internal resistance of the battery is negligible. The switch S is closed at t=0. The potential drop across L as a function of time is

View Solution
Knowledge Check
An inductor of inductance L = 400 mH and resistors of resistance R1=2Ω and R2=2Ω are connected to a battery of emf 12V as shown in the figure.The internal resistance of the battery is negligible.The switch S is closed at t = 0.The potential drop across L as a function of time is
 A12te−3tVB6(1−e−t0.2)VC12e−5tVD6e−5tV
A12te−3tVB6(1−e−t0.2)VC12e−5tVD6e−5tVAn inductor of inductance L = 400 mH and resistors of resistance R1=2Ω and R2=2Ω are connected to a battery of emf 12 V as shown in the figure. The internal resistance of the battery is negligible. The switch S is closed at t=0. The potential drop across L as a function of time is
 A12te−3tVB6(1−e−t/0.2)VC12e−5tVD6e−5tV
A12te−3tVB6(1−e−t/0.2)VC12e−5tVD6e−5tVAn inductor of inductance L = 400 mH and resistors of resistance R1=2Ω and R2=2Ω are connected to a battery of emf 12 V as shown in the figure. The internal resistance of the battery is negligible. The switch S is closed at t=0. The potential drop across L as a function of time is
 A12te−31VB6(1−e−t/0.2)VC12e−5tVD5e−5tV
A12te−31VB6(1−e−t/0.2)VC12e−5tVD5e−5tV
An inductor of self-inductance L and resistor of resistance R are connected in series to a battery of emf E and negligible resistance.Calculate the maximum rate at which energy is stored in the inductor.
View SolutionCalculate the steady state current in the 2Ω resistor, shown in figure. The internal resistance of the battery is negligible and the capacitance of the capacitor C is 0.2μF.
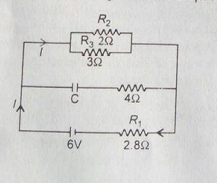
View SolutionCalculate the steady state current in the 2Ω resistor, shown in figure. The internal resistance of the battery is negligible and the capacitance of the capacitor C is 0.2μF.

View SolutionThe steady state current in a 2Ω resistor when the internal resistance of the battery is negligible and the capacitance of the condenser is 0.1μF is
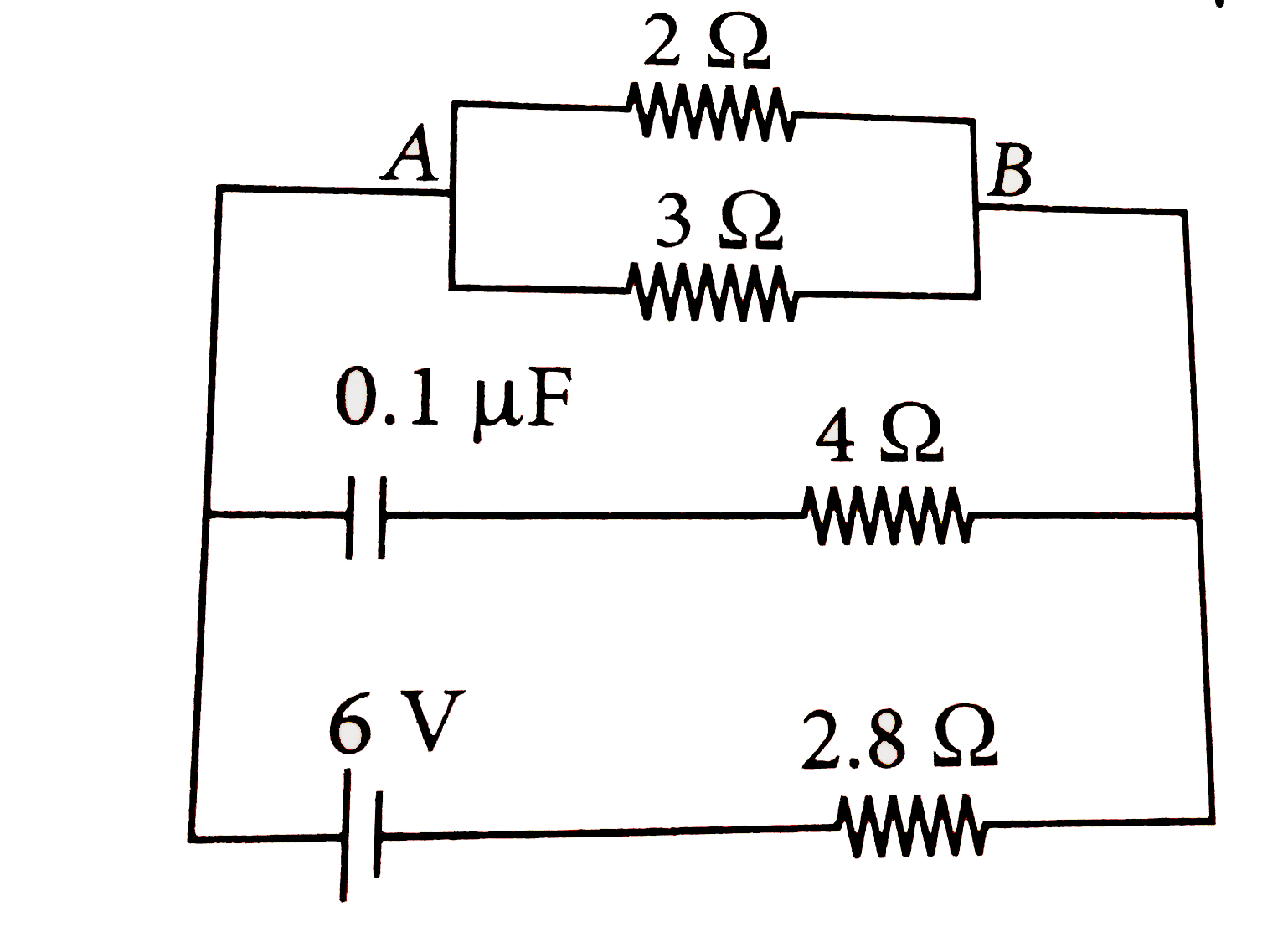
View SolutionA 4μF capacitor is connected to a battery of emf 24V. Through a resistance of 5MΩ and a switch which is kept open initially. Internal resistance of the battery is negligible. Switch is closed at t=0. Potential difference across capacitor and resistor at t=0 are respectively.
View Solution
