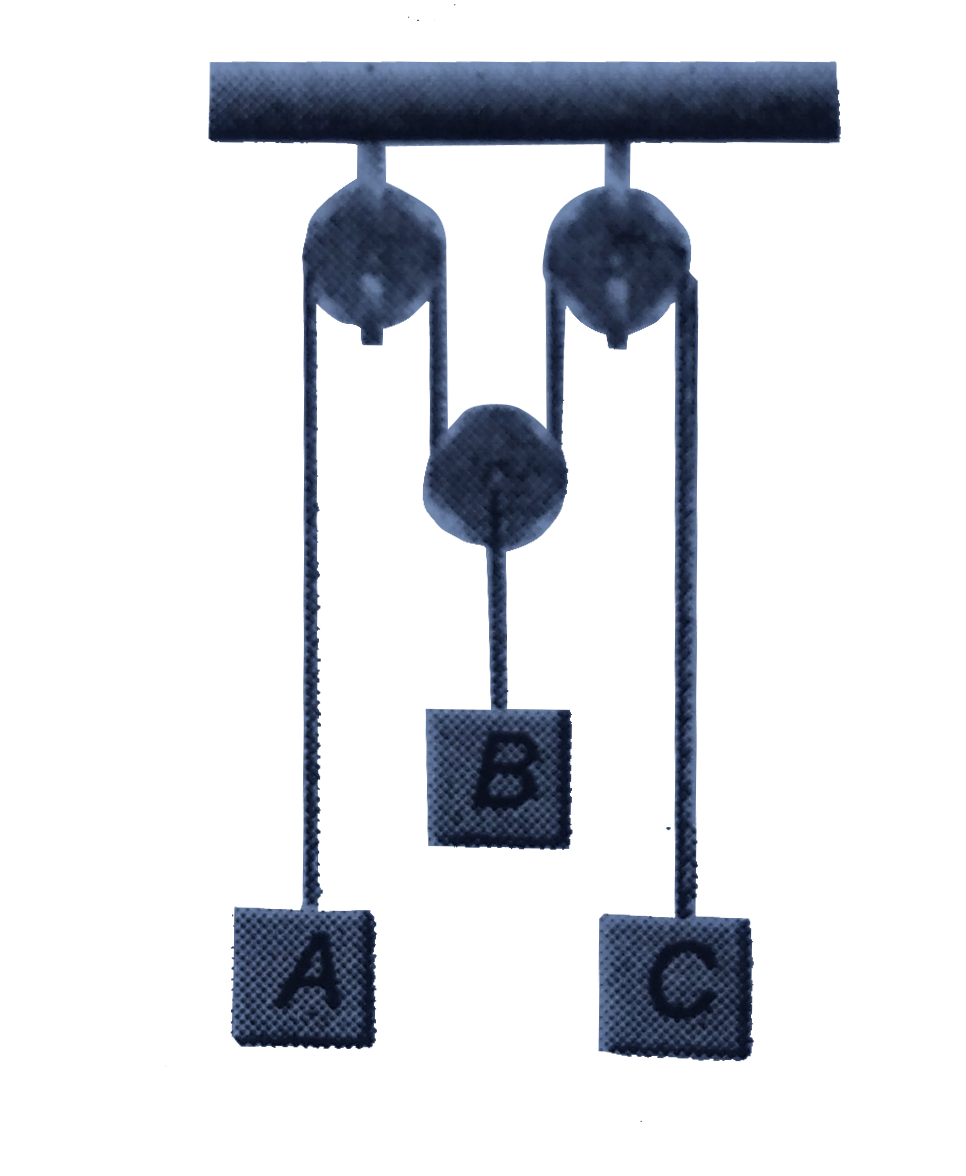
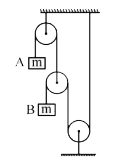
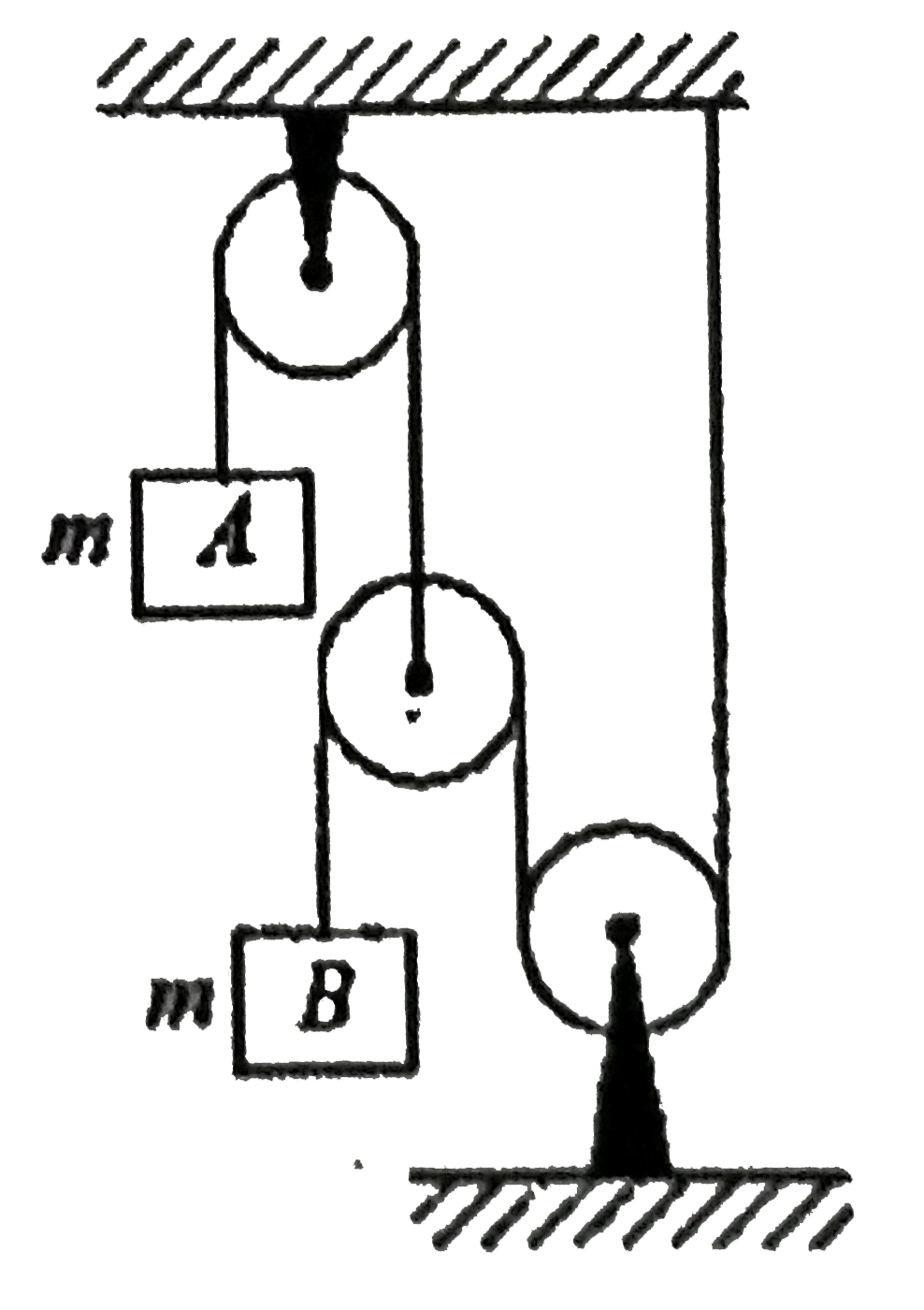
In the pulley-block arrangement shown in figure , find the relation between acceleration of blocks A and B

TA=3T,TB=T
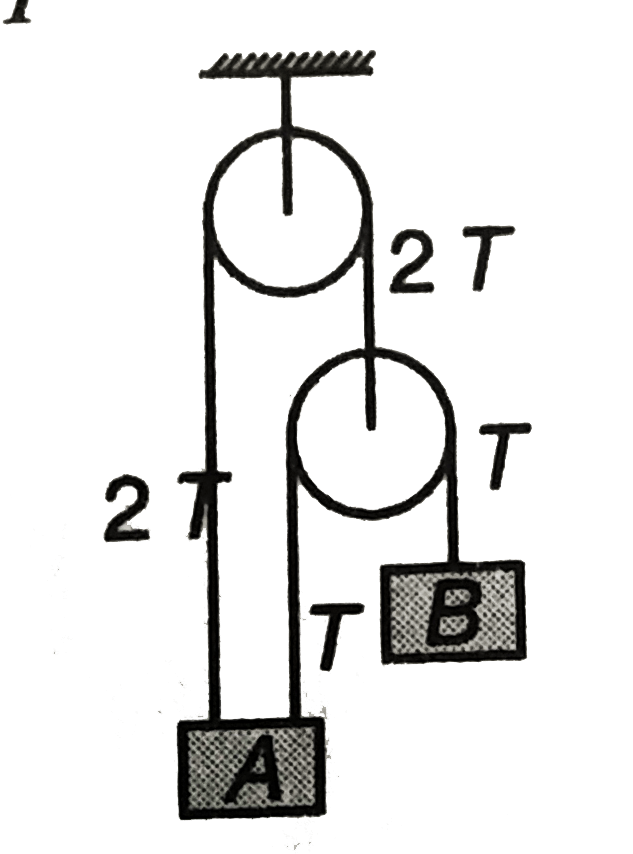
In such try of problem
a∝1T
∴|aB|=3|aA|
or aB=−3aA as aA and aB are in opposite direction.
Topper's Solved these Questions
- BOOK - DC PANDEYCHAPTER - LAWS OF MOTIONEXERCISE - Objective Question32videos
LAWS OF MOTION
- BOOK - DC PANDEYCHAPTER - LAWS OF MOTIONEXERCISE - Comprehension9videos
LAWS OF MOTION
- BOOK - DC PANDEYCHAPTER - LAWS OF MOTIONEXERCISE - Exercise 8.52videos
LAWS OF MOTION
- BOOK - DC PANDEYCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE15videos
KINEMATICS 1
- BOOK - DC PANDEYCHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Level 2 Subjective18videos
LAWS OF THERMODYNAMICS
Similar Questions
Knowledge Check
- Question 1 - Select One
In an arrangement shown in the figure, the acceleration of block A and B are given-
 Ag/3,g/6Bg/6,g/3Cg/2,g/2D0,0
Ag/3,g/6Bg/6,g/3Cg/2,g/2D0,0 - Question 2 - Select One
In the arrangement shown, the pulleys and the string are ideal. The acceleration of block B is.
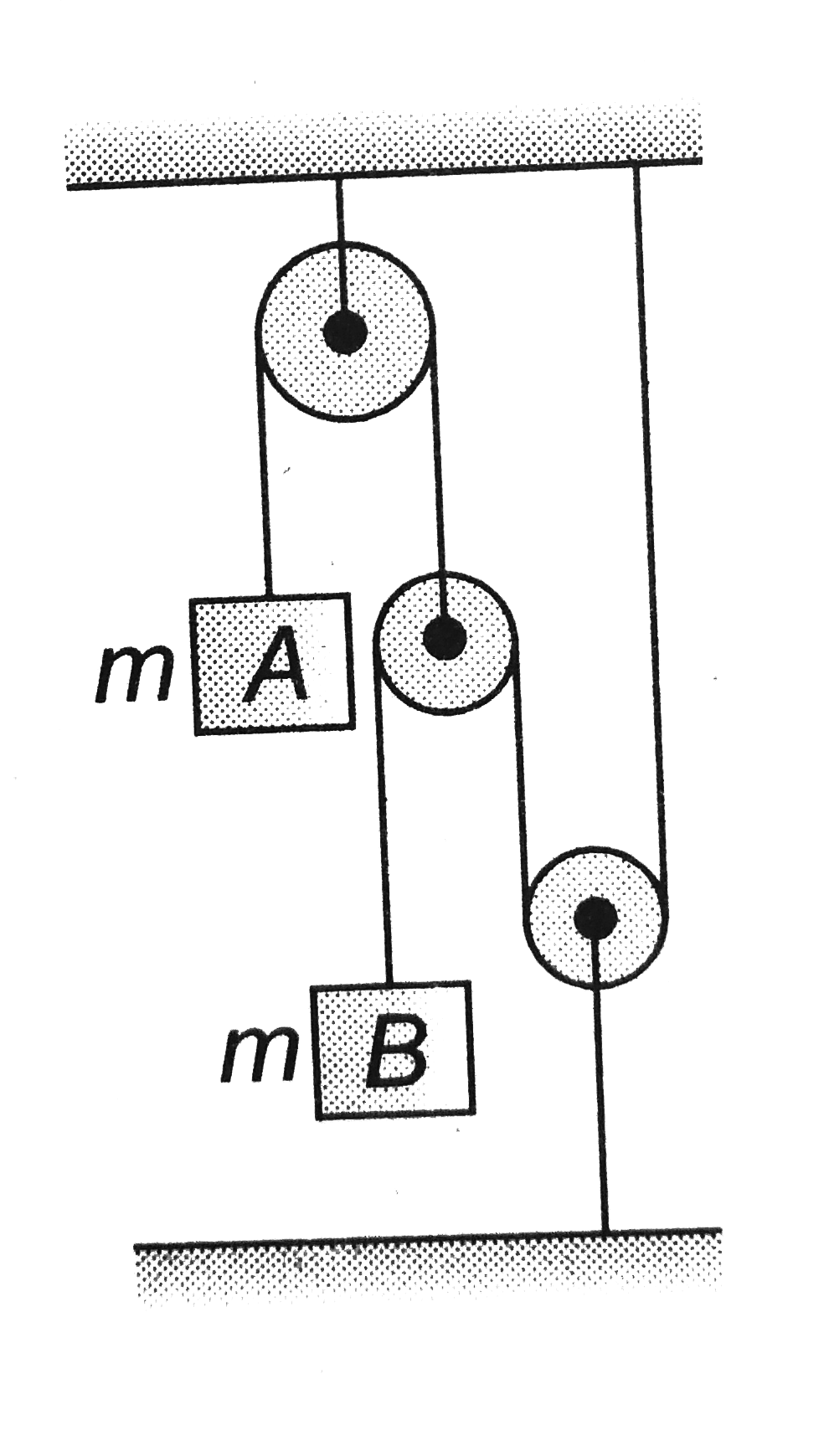 Ag/5Bg/2C2g/5D2g/3
Ag/5Bg/2C2g/5D2g/3 - Question 3 - Select One
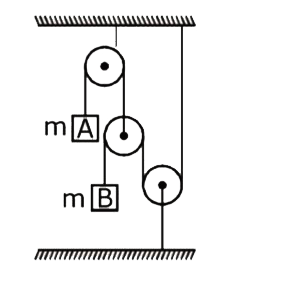
In the arrangement shown, the pulleys and strings are massless. The acceleration of block B is :
 Ag/2B5g/3C2g/3D2g/5
Ag/2B5g/3C2g/3D2g/5
In the arrangement shown, the pulleys and the strings are ideal.The acceleration of the block B is
 View Solution
View SolutionIn the arrangement shown in figure , find the retio of tension in the strings attached with 4kg block and the with 1kg block
 View Solution
View SolutionIn the system shown in figure, the block A is released from rest. Find
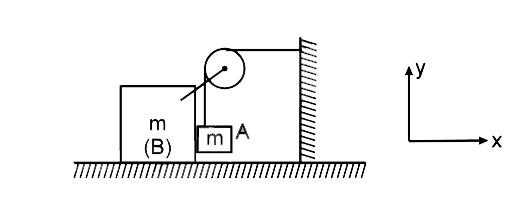
the acceleration of both blocks ‘A’ and ‘B’.View SolutionIn the arrangement shown in the figure the coefficient of friction between the blocks C and D is μ1=0.7 and that between block D and the horizontal table is μ2=0.2. The system is released from rest. [ Take g=10ms–2] Pulleys and threads are massless.
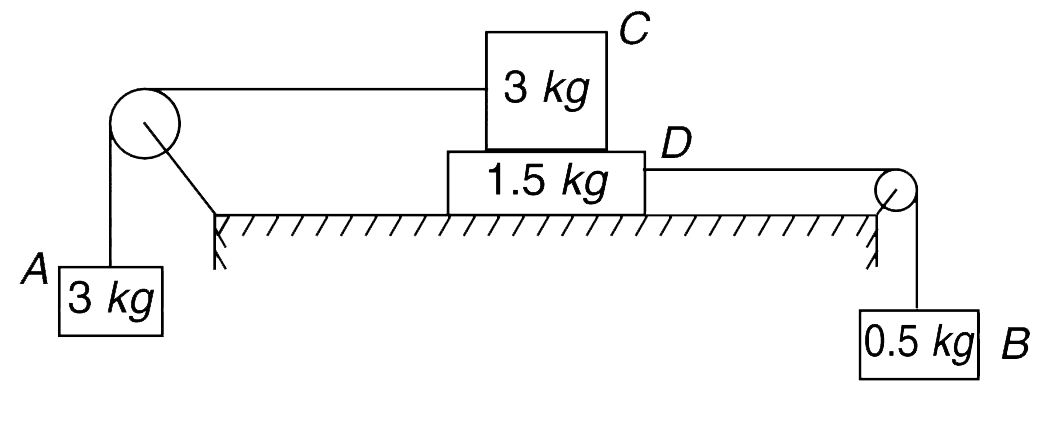
(a) Find the acceleration of the block C.
(b) Block B is replaced with a new block. What shall be the minimum mass of this new block so that block C and D accelerate in opposite direction?View SolutionIn the arrangement shown, the pulleys are smooth and the strings are inextensible. The acceleration of block B is :
 View Solution
View Solution