A, B be points with PV's $\to a, \to b$ . If the point C on OA is such that $2 \to A C = - - \to C O . - - \to C D$ is parallel to $\to O B$ and $\to C D = \to O B$ then $\to A D$

Video Solution

|

Answer

Step by step video & image solution for A, B be points with PV's vec a, vecb. If the point C on OA is such that 2vec AC =vec (CO).vec (CD) is parallel to vec OB and vec CD= vec OB then vec AD by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023

Class 12 MATHS QUESTION BANK

Similar Questions

Knowledge Check

Assertion A : If A, B, C, D are four points on a semi-circular arc with centre at 'O' such that
$∣ ∣ ∣ - - \to A B ∣ ∣ ∣ = ∣ ∣ ∣ - - \to B C ∣ ∣ ∣ = ∣ ∣ ∣ - - \to C D ∣ ∣ ∣$ , then
$- - \to A B + - - \to A C + - - \to A D = 4 - - \to A O + - - \to O B + - - \to O C$
Reason R : Polygon law of vector addition yields
$- - \to A B + - - \to B C + - - \to C D + - - \to A D = 2 - - \to A O$

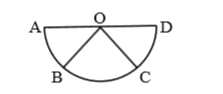
In the light of the above statements, choose the most appropriate answer from the options given below :
AA is correct but R is not correct.
BA is not correct but R is correct.
CBoth A and R are correct and R is the correct explanation of A.
DBoth A and R are correct but R is not the correct explanation of A.

Recommended Questions

Home
Ask Doubt
Profile