Torque acting on a rotating body

Video Solution

Text Solution

Verified by Experts

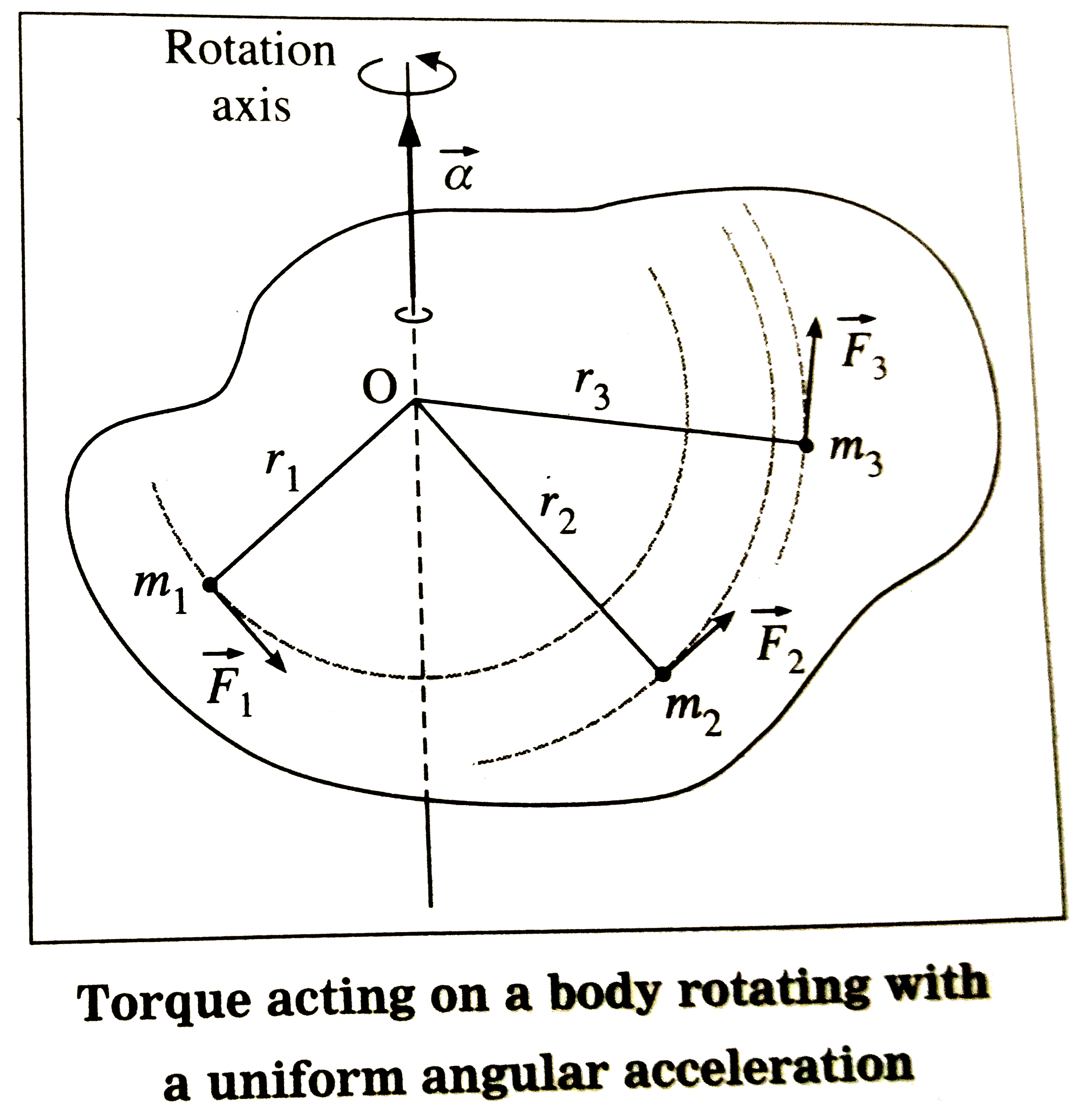
A torque on a body produces angular acceleration. Consider a rigid body rotatin about an axis passing through the point O and perpendicular to the plane of the figure. Suppose that a torque $\vec{τ}$ on the body produces uniform angular acceleration $\vec{α}$ along the axis of rotation.
The body can be considered as made of N particles with masses $m_{1}, m_{2}, ... ., r_{N}$ respectively from the axis of rotaion. $\vec{α}$ is the same for all the particles as the body is rigid. Let $\vec{F_{1}}, \vec{F_{2}}, ... ., {\vec{F}}_{N}$ be the external forces on the particles.
the torque $\vec{τ_{1}},$ on the particle of mass $m_{1},$ is $\vec{τ_{1}} = \vec{r_{1}} \times \vec{F_{1}}$ .
$∴ τ_{1} = r_{1} F_{1} \sin θ$ where $θ$ is the smaller of the two angles between $\vec{r_{1}}$
and $\vec{F_{1}} . ∴ τ_{1} = r_{1} F_{1}$ (since, in this case, $θ = 90^{\circ})$
Now, $F_{1} = m_{1} a_{1} = m_{1} r_{1} α (∵ a_{1} = r_{1} α) ... (1)$
$∴ τ_{1} = r_{1} (m_{1} r_{1} α) = m_{1} r_{1}^{2} α ... (2)$
Similarly , $τ_{2} = m_{2} r_{2}^{2} α, ... . τ_{N} = m_{N} r_{N}^{2} α$
The total torque on the body is
$τ = τ_{1} + τ_{2} + ... + τ_{N}$
$= m_{1} r_{1}^{2} α + m_{2} r_{2}^{2} α + ... m_{N} r_{N}^{2} α$
$= (m_{1} r_{1}^{2} + m_{2} r_{2}^{2} + ... . + M_{N} r_{N}^{2}) α ... . (3)$
$= (\sum_{i = 1}^{N} m_{i} r_{i}^{2}) α$
$∴ τ = I α ... (4)$
where $I = \sum_{i = 1}^{N} m_{i} r_{i}^{2}$ is hte moment of inertia of the body about the axis of roataion.
In vector form $τ = \vec{I α}$
This gives the requuired relation. Angular acceleration $\vec{α}$ has the same direction s the torque $\vec{a} τ$ adn both of them are along the rotation axis.

Updated on:21/07/2023

If the torque acting on a rigid body, under the application of a force, is zero then it's
Alinear momentum is conserved
Bangular momentum is not conserved
Cenergy is conserved
Dangular momentum is conserved
If the torque acting on a rigid body, under the application of a force, is zero then it's
Alinear momentum is conserved
Bangular momentum is not conserved
Cenergy is conserved
Dangular momentum is conserved
A torque of 50 Nm acts on a rotating body for 5 s. Its angular momentum is
Aincreases by 250 kg $m^{2} / s$
Bincreases by 10 kg $m^{2} / s$
Cincreases by 55 kg $m^{2} / s$
Ddecreases by 250 kg $m^{2} / s$