Establish a relation between angular momentum and moment of inertia of a rigid body.

Video Solution

Text Solution

Verified by Experts

Consider a rigid body rotating with a constant velocity $\to ω$ about an axis through the point O and perpendicular to the plane of the figure. As the body rotates, all the particles of the body perform uniform circular motion about the axis of rotation with the same angular velocity $\to ω$ .Suppose that the body consists of N particles of masses $m_{1}, m_{2}, ... . . m_{N}$ ,situated at distances $r_{1}, r_{2}, ... . . r_{N}$ , respectively from the axis of rotation.
As the body rotates, the particles of mass $m_{1}$ revolves along a circle of radius $r_{1}$ , with a linear velocity of magnitude $v_{1} = r_{1} ω$ .

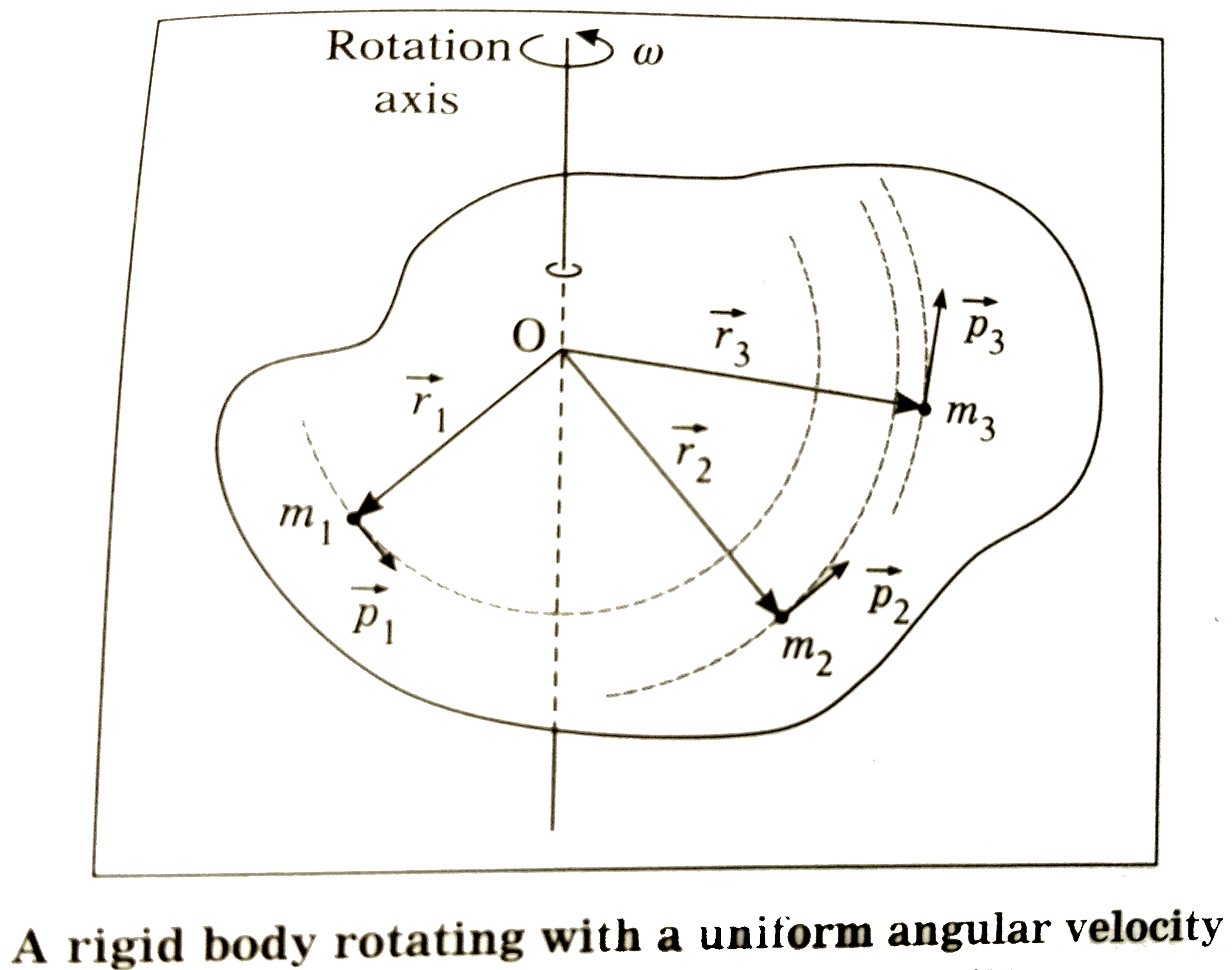
The magnitude of the linear momentum $(p_{1})$ of the particle is
$p_{1} = m_{1} v_{1} = m_{1} r_{1} ω$
The angular momentum of the particle about the axis of rotation is by definition,
${\to L}_{1} = {\to r}_{1} \times {\to p}_{1}$
$∴ L_{1} = r_{1} p_{1} sin θ,$ where $θ$ is the smaller angle between ${\to r}_{1}$ and ${\to p}_{1}$ .
In this case, $θ = 90^{\circ} ∴ sin θ = 1$
$∴ L_{1} = r_{1} p_{1} = r_{1} m_{1} r_{1} ω = m_{1} r_{1} ω = m_{1} r_{1}^{2} ω$
Similarly the angular momentum $(L_{2})$ of the particle of mass $m_{2}$ is $m_{2} r_{2}^{2} ω$ , that of the particle of mass $m_{3}$ is $m_{3} r_{3}^{2} ω$ , etc.The angular momentum of the body about the given axis is
$L = L_{1} + L_{2} + ... ... . . + L_{N}$
$= m_{1} r_{1}^{2} ω + m_{2} r_{2}^{2} ω + ... . . m_{N} r_{N}^{2} ω$
$= (m_{1} r_{1}^{2} + m_{2} R_{2}^{2} + ... . . + M_{N} r_{N}^{2}) ω$
$= (N Σ i = 1 m_{i} r_{i}^{2}) ω$
$∴ L = I ω$ , where $I = N Σ i = 1 m_{i} r_{i}^{2}$ =moment of inertia of the body about the given axis. In vector form,
$\to L = I \to ω$
Thus, angular momentum=moment of inertia $\times$ angular velocity.

Updated on:21/07/2023