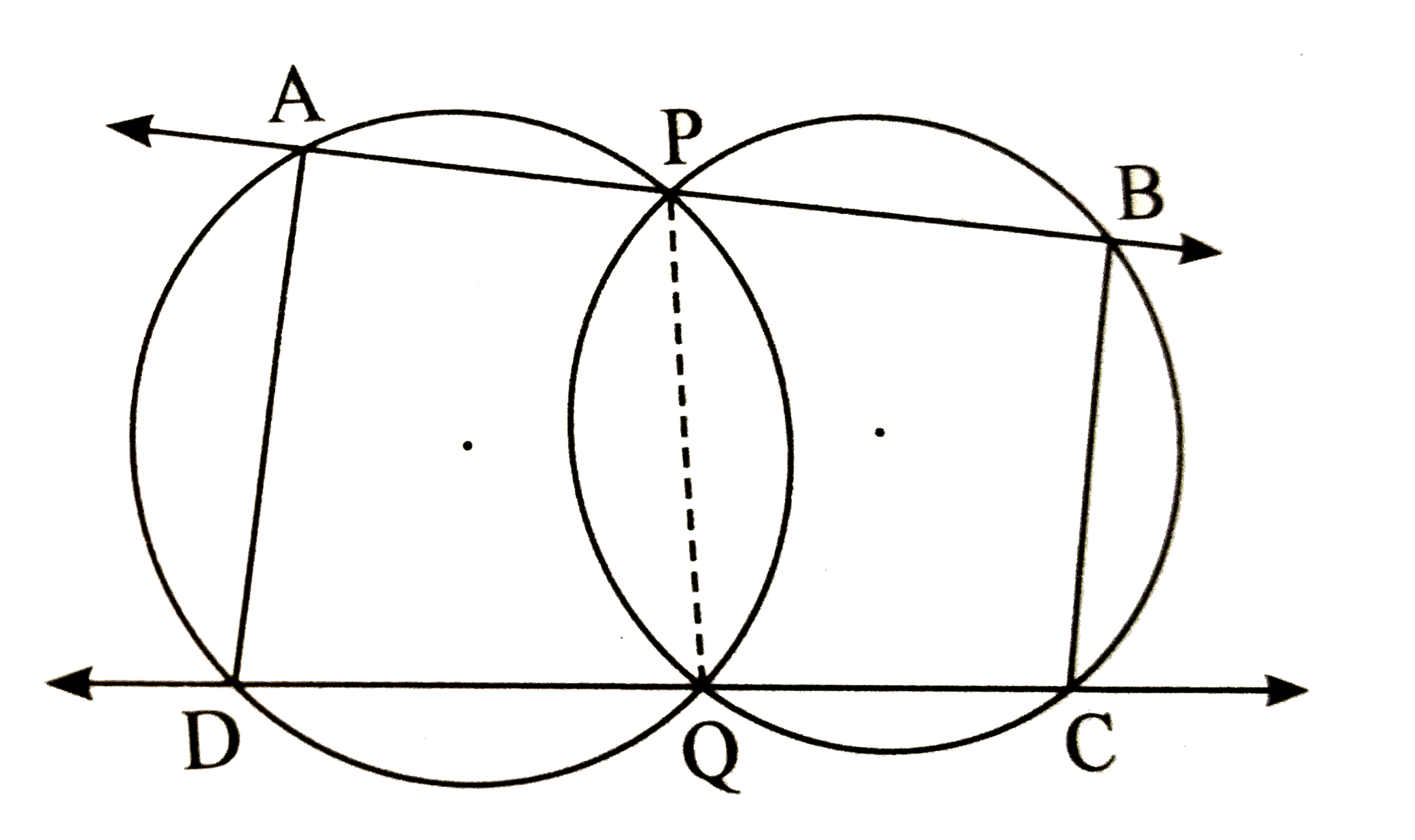
Parama drew to circles intersect each other at the points P and Q . If the diameter of the two ircels are PA and PB resepctively , then prove that A,Q,B are collinear. Solution in Bengali
Let two circles with centres O and O' intersects each other at P and Q.
PA is a diameter of the circle with centres at O and PB is alos a diameter of the circle at O,
To prove A,Q and B are collinear.
Construction : Let us join P,Q
Proof : In the with centre at O,∠AQP is a semi-circular angle ∴∠PQA=1 right angle.
Again, in the circle with centre at O,∠BQP is a semicircular angle
Now, ∠PQO+∠PQB=1 right angle +1 right angle.
or, ∠AQB=2 right angle or, ∠AQB=180∘or,∠AQB= straight angle.
∴∠AQB=1 straight angle.
Hence the point A,Q,B are colliear.

Topper's Solved these Questions
- BOOK - CALCUTTA BOOK HOUSECHAPTER - THEOREMS RELATED TO ANGLES IN A CIRCLEEXERCISE - EXAMPLES (True or False)4videos
THEOREMS RELATED TO ANGLES IN A CIRCLE
- BOOK - CALCUTTA BOOK HOUSECHAPTER - THEOREMS RELATED TO ANGLES IN A CIRCLEEXERCISE - EXAMPLES (Short-Answer Type Questions) MCQs5videos
THEOREMS RELATED TO ANGLES IN A CIRCLE
- BOOK - CALCUTTA BOOK HOUSECHAPTER - THEOREMS RELATED TO ANGLES IN A CIRCLEEXERCISE - EXAMPLES (Short Answer Type Questions)5videos
THEOREMS RELATED TO ANGLES IN A CIRCLE
- BOOK - CALCUTTA BOOK HOUSECHAPTER - THEOREMS RELATED TO CYCLIC QUADRILATERAL EXERCISE - Long -answer type questions (L.A.)12videos
THEOREMS RELATED TO CYCLIC QUADRILATERAL
- BOOK - CALCUTTA BOOK HOUSECHAPTER - THEOREMS RELATED TO TANGENT IN A CIRCLE EXERCISE - EXERCISE 4.223videos
THEOREMS RELATED TO TANGENT IN A CIRCLE
Similar Questions
In the adjoining figure AD and BE are perpendicular ot BC and AC respectively of the ΔABC. The four points A,B,D,E are concylic.
04:59View SolutionIn the adjoining figure, O is the centre of the circle AC is a diameter and chord DE is parallel to the diameter AC. If ∠CBD=60∘ , find the value of ∠CDE
03:00View SolutionIn the adjacent figure O is the centre of the circle. If ∠OAB=30∘,∠ABC=120∘,∠BCO=y∘and∠COA=x∘, then the find value sof x and y.
03:23View SolutionIn a cyclic quadrilateral, the ratio of three consecutive angle is 1 : 2 : 3 Find the value of first and third angles.
04:49View SolutionThe distance of the point from the centre of a circle with diameter of 16 cm is 17 cm. Then the length of the tangent from the point to the circle is
07:47View SolutionTwo circles intersect each other at the points P and Q. Two straight lines through P and Q intersect one circle at the points A and C and the other circle at B and D. Prove the AC|| BD
03:06View Solutionदो समान वृत्त एक-दूसरे को बिन्दुओं A और B पर काटते हैं | A से होकर जाने वाली एक वृत्तों को P और Q बिन्दुओं पर मिलती है | सिद्ध कीजिए कि BP = BQ.
03:05View SolutionTwo circles intersect each other at point P and Q. Secants drawn through p and Q intersect the circles at points A,B and D,C
Prove that :∠ADC+∠BCD=180∘
 03:15View Solution
03:15View SolutionAnkita drew two circles which intersect each other at the points P and Q. Through the point P two straight lines are draw so that they intersect one of the circle at the points. A and B and the ohte circle at the points C and D respectively. Prove that ∠AQC=∠BQD.
06:31View SolutionTwo circles intersect each other at the points P and Q. Two straight lines through P and Q intersect on circle at the points A and C and the other circle at B and D . Prove that AC||BD.
05:54View SolutionTwo circles intersect each other at P and Q. PA and PB are two diameters. Then ∠AQB is
03:51View SolutionTwo congruent circles intersect each other at points A and B. Through A any linesegment PAQ is drawn so that P, Q lie on the two circles. Prove that BP = BQ.
07:05View SolutionSahana draws two circles that intersect at points P and Q. If PA and PB are the diameters of two circles, respectively, prove that the points A, Q and B are equidistant.
01:07View SolutionTwo circles intersect each other at the points P and Q. Two straight lines through P and Q intersect one circle at the points A and C and
the other circle at B and D. Prove the AC|| BD04:39View SolutionTwo cirlces intersect at P and Q . Through P diameter PA and PB of the two circles are drawn. Show that the points A,Q and B are collinear.
03:11View Solution

