A stationary circular loop of radius a is located in a magnetic field which varies with time from t = 0 to t = T according to law $B = B_{0} t (T - t)$ . If plane of loop is normal to the direction of field and resistance of the loop is R, calculate
magnitude of charge flown through the loop from instant t = 0 to the instant when current reverses its direction.

A

$\frac{π^{2} a^{2} B_{0} T^{2}}{R}$

B

$\frac{π^{2} a^{2} B_{0} T^{2}}{4 R}$

C

$\frac{4 π^{2} a^{2} B_{0} T^{2}}{R}$

D

None of these

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

Since magnetic field strength B varies with time, therefore an emf is incuced in the loop ans a current starts flowing through the loop. Due to flow of current heat is generated.
At an instant t, flux linked with the loop.
$ϕ = π s^{2} B_{0} (t T - t^{2})$
Induced emf, $e = - \frac{d ϕ}{d t} = - π^{2} B_{0} (T - 2 t)$
Induced current , $i = \frac{e}{R} = \frac{π a^{2} B_{0}}{R} (2 t - T)$
Thermal power generated at this instant,
$P = \frac{π^{2} a^{4} B_{0}^{2}}{R} {(2 t - t)}^{2}$
During an elementary time interval dt, heat generated,
$p d t = \frac{π^{2} a^{4} B_{0}^{2}}{R} {(2 t - T)}^{2} \cdot d t$
Total heat generated from $t = 0 \to t = T$ .
$Q = \int P d t = \frac{π^{2} a^{4} B_{0}^{2}}{R} \int_{0}^{T} {(2 t - T)}^{2} d t$
$= \frac{π^{2} a^{4} B_{0}^{2} T^{3}}{3 R}$
The current reverses its sign when its magnitude reduces to zero. Let this happen at instant $t = t_{0}$ . Substituting t by $t_{0}$ in equation (i).
$\frac{π a^{2} B_{0}}{R} (2 t - t_{0}) = 0$
$t_{0} = \frac{T}{2}$
$∴$ Charge that flows from $t = 0$ to $t = T / 2$
$q = \frac{π a^{2} B_{0}}{R} \int_{0}^{T / 2} (2 t - T) d t = \frac{π a^{2} B_{0} T^{2}}{4 R}$ .

|

Updated on:21/07/2023

Class 12 PHYSICS MOVING CHARGES AND MAGNETISM

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A stationary circular loop of radius a is located in a magnetic field which varies with time from $t = 0 \to t = T$ according to law $B = B_{0} t (T - t)$ . If plane of loop is normal to the direction of field and resistance of the loop is R, calculate
amount of heat generated in the loop during this interval.
A $\frac{π^{2} a^{4} B_{0}^{2} T^{3}}{3 R}$
B $\frac{π^{2} a^{4} B_{0}^{2} T^{3}}{R}$
C $\frac{3 π^{2} a^{4} B_{0}^{2} T^{3}}{R}$
DNone of these
Question 2 - Select One
Magnetic field B in a cylindrical region of radius r varies according to the law $B = B_{0} t$ as shown in the figure. A fixed conducting loop ABCDA of resistance R is lying in the region as show . The current flowing through the loop is

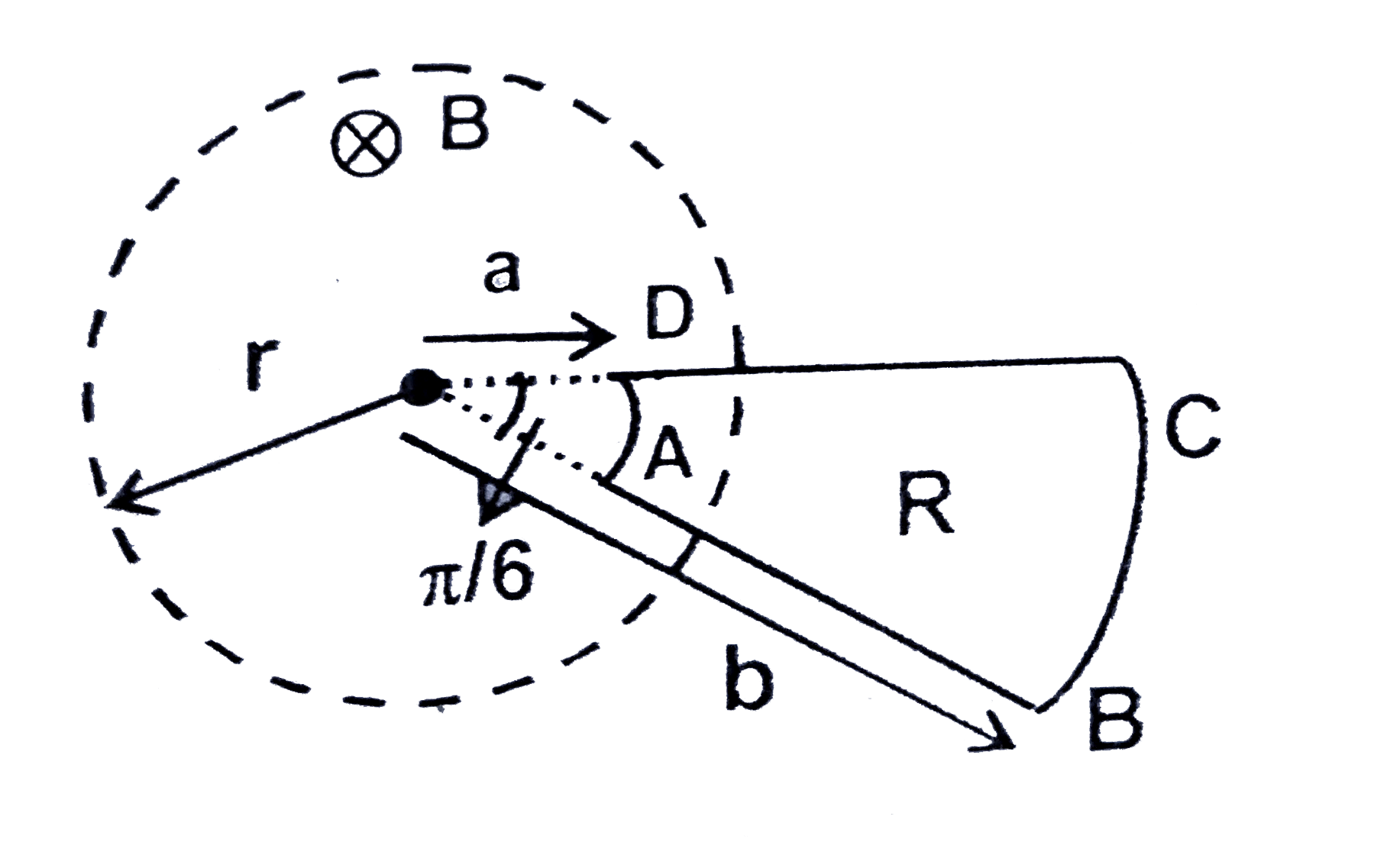A $\frac{π a^{2} B_{0}}{12 R}$
B $\frac{π (r^{2} - a^{2}) B_{0}}{12 R}$
C $\frac{π (b^{2} - a^{2}) B_{0}}{12 R}$
Dnone of the above
Question 3 - Select One
A circular wire loop of radius r lies in a uniform magnetic field B, with its plane perpendicular to magnetic field. If the loop is deformed to a square shape in the same plane in time t, the emf induced in the loop is
A $\frac{π B r^{2}}{t} (1 - \frac{π}{2})$
B $\frac{π B r^{2}}{t} (1 - \frac{π}{3})$
C $\frac{π B r^{2}}{t} (1 - \frac{π}{4})$
D $\frac{π B r^{2}}{t} (1 - \frac{π}{5})$

CENGAGE PHYSICS-MISCELLANEOUS VOLUME 5-Linked Comprehension

Home
Ask Doubt
Profile