Consider the circuit shown below. When switch S1 is closed, let I be the current at time t, then applying Kirchhoff's law
E−iR−Ldidt=0or∫i0diE−iR=1L∫10dt
i=ER(1−e−Rl⋅t)
LR = time constant of circuit
When current reaches its steady value (=i0, open S1 and close S2, the current does reach to zero finally but decays expnentially. The decay equation is given as i=i0e−RL⋅T).
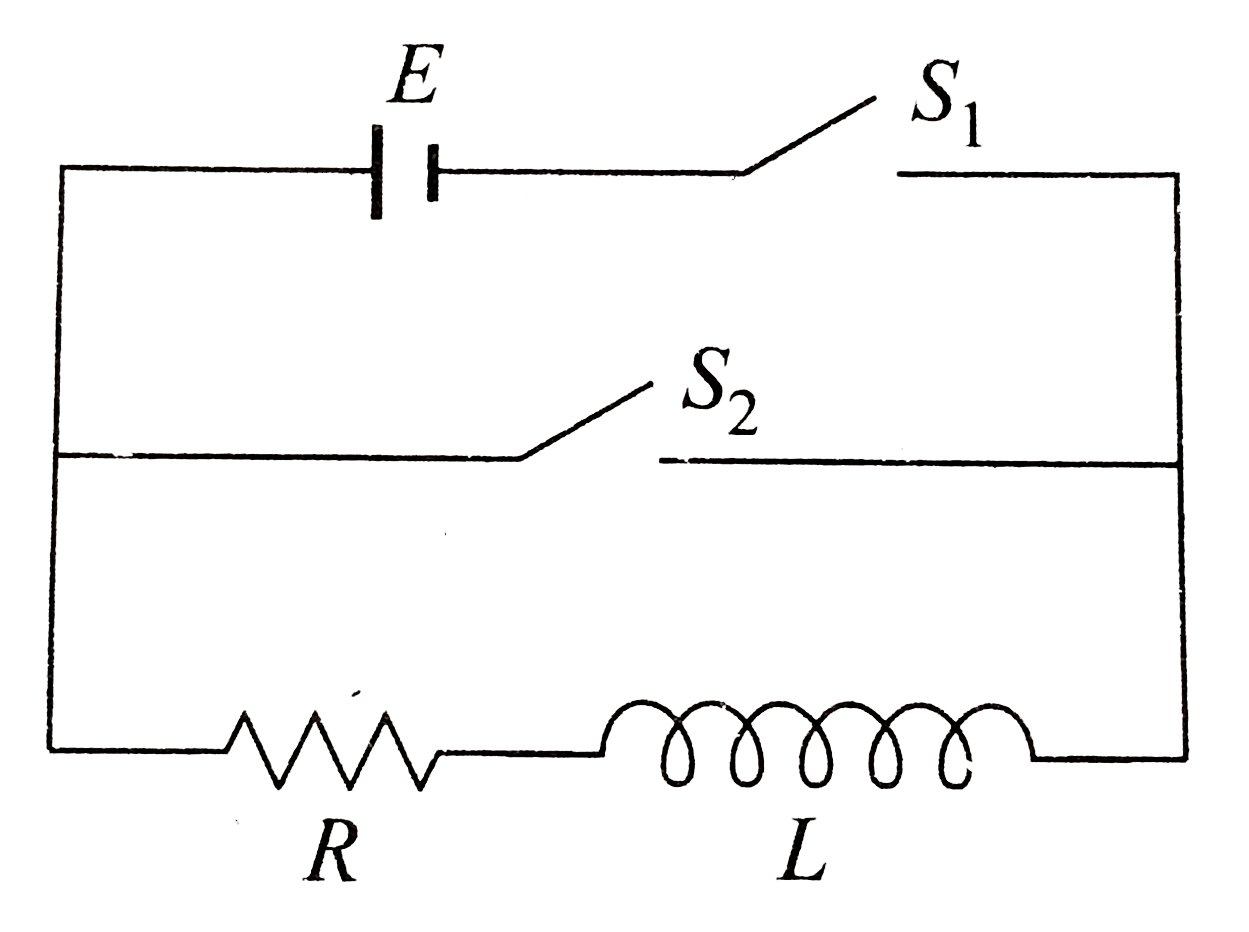
When a coil carrying a steady current is short circuited, the current decreases in it η times in time t0. The time constant of the circuit is
A
t01nη
B
t01nη
C
t0η
D
t0η−1
Video Solution
Text Solution
The correct Answer is:B
I=I0e−t/τ
Given , I−cI0att=t0
⇒η=e−t0τ
⇒1nη=−t0τ
⇒τ=t01nη.
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - MISCELLANEOUS VOLUME 5EXERCISE - Integer12videos
MISCELLANEOUS VOLUME 5
- BOOK - CENGAGE PHYSICSCHAPTER - MISCELLANEOUS VOLUME 5EXERCISE - Multiple Correct34videos
MISCELLANEOUS VOLUME 5
- BOOK - CENGAGE PHYSICSCHAPTER - MISCELLANEOUS VOLUME 3EXERCISE - True and False3videos
MISCELLANEOUS VOLUME 3
- BOOK - CENGAGE PHYSICSCHAPTER - Moving charges and magnetismEXERCISE - Question Bank20videos
Moving charges and magnetism
Similar Questions
Knowledge Check
- Question 1 - Select One
Consider the circuit shown below. When switch S1 is closed, let I be the current at time t, then applying Kirchhoff's law
E−iR−Ldidt=0or∫i0diE−iR=1L∫10dt
i=ER(1−e−Rl⋅t)
LR = time constant of circuit
When current reaches its steady value (=i0, open S1 and close S2, the current does reach to zero finally but decays expnentially. The decay equation is given as i=i0e−RL⋅T.
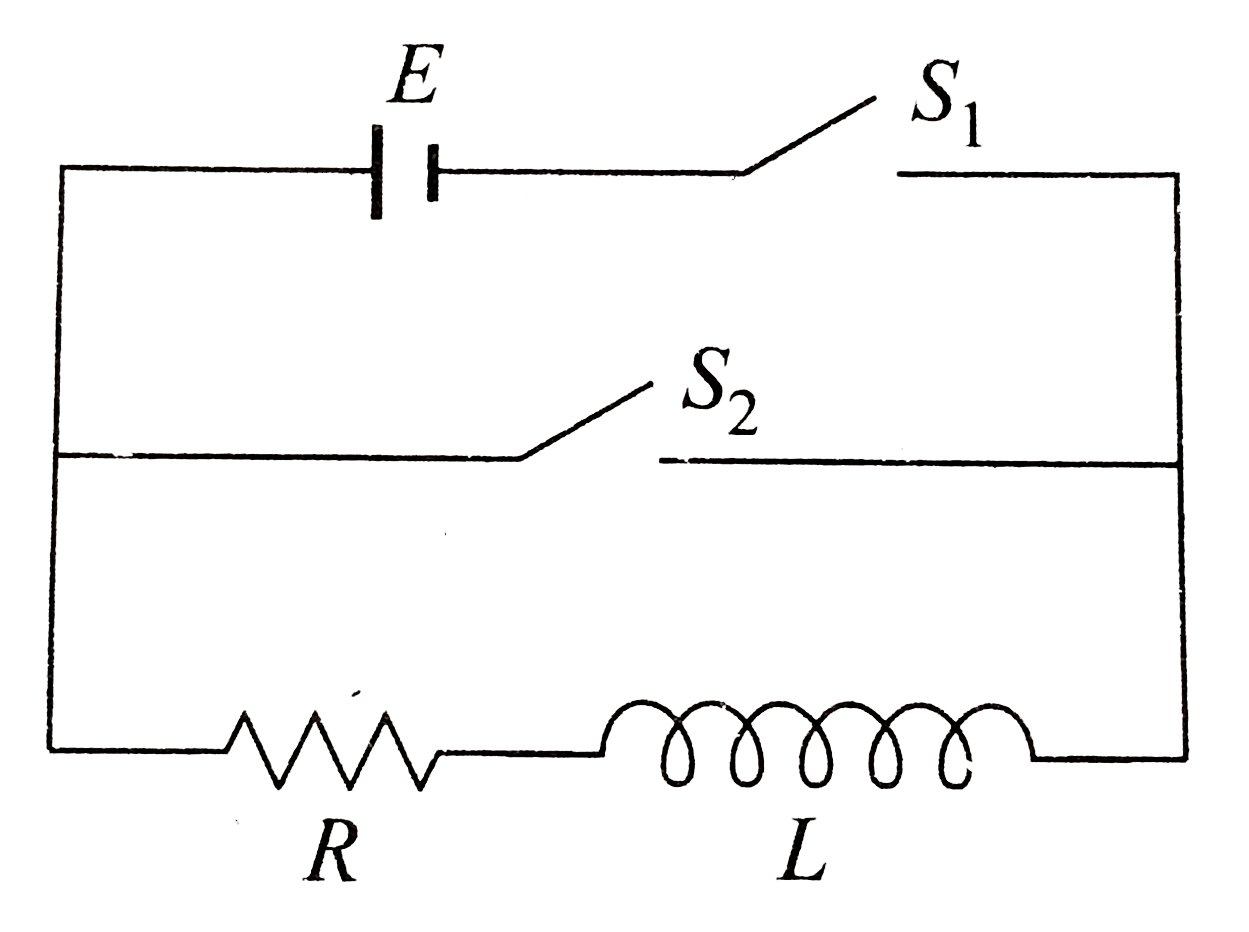
A wire is sliding as shown in fig . The angle between the acceleration and velocity of the wire is
 A30∘B40∘C120∘D90∘
A30∘B40∘C120∘D90∘ - Question 2 - Select One
Consider the circuit shown below. When switch S1 is closed, let I be the current at time t, then applying Kirchhoff's law
E−iR−Ldidt=0or∫i0diE−iR=1L∫10dt
i=ER(1−e−Rl⋅t)
LR = time constant of circuit
When current reaches its steady value (=i0, open S1 and close S2, the current does reach to zero finally but decays expnentially. The decay equation is given as i=i0e−RL⋅T.
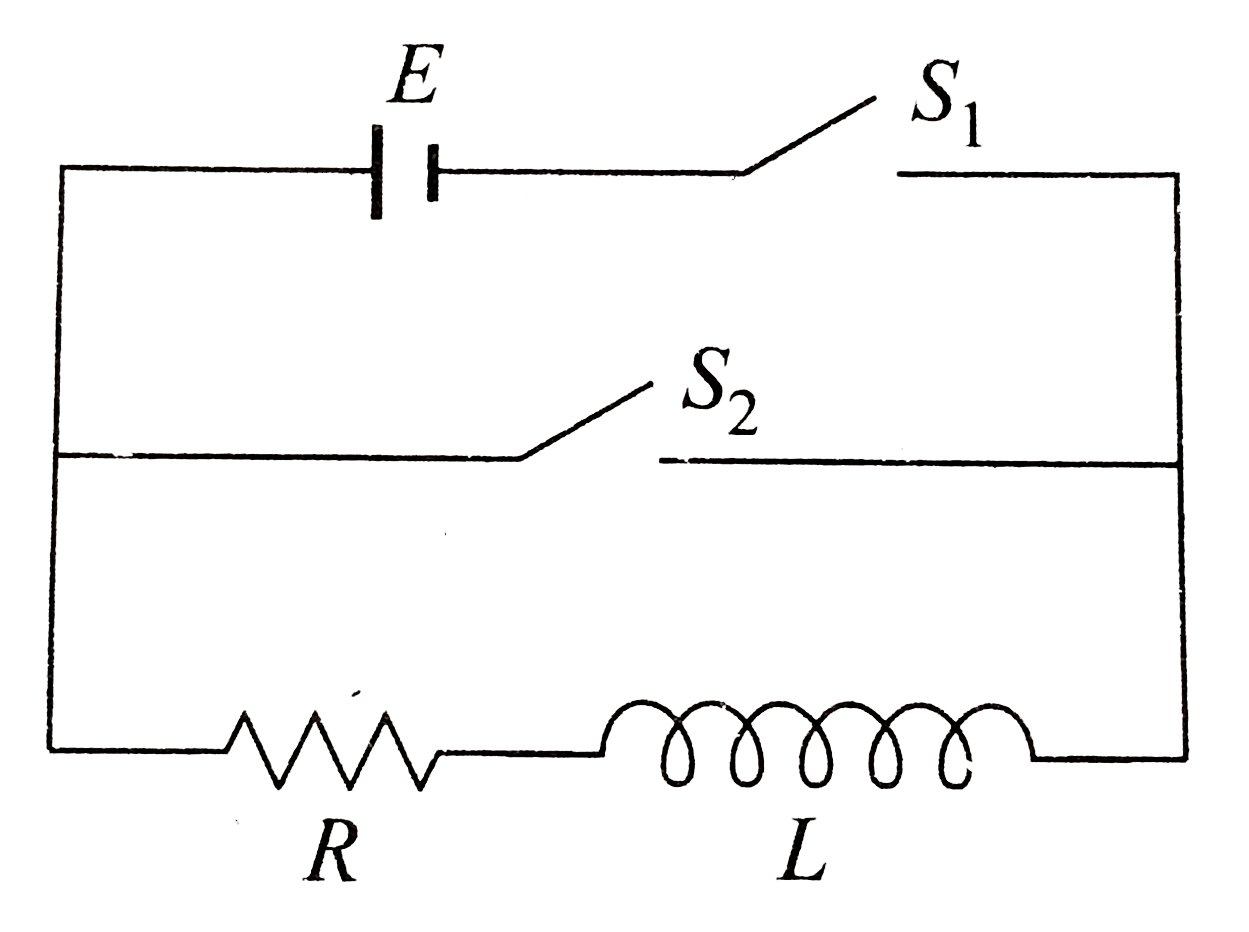
Three idenctical rings. The first (a). slips without rolling and the second (b) rolls without slipping and the third rolls with slipping .AThe same emf is induced in all three rings.BNo emf is induced in any of the rings.CIn each ring all points are at same potentialDB develops max. Induced emf and A, the least. - Question 3 - Select One
In the circuit shown, the switch is closed at t=0, the currents I1,I2 and I3 are
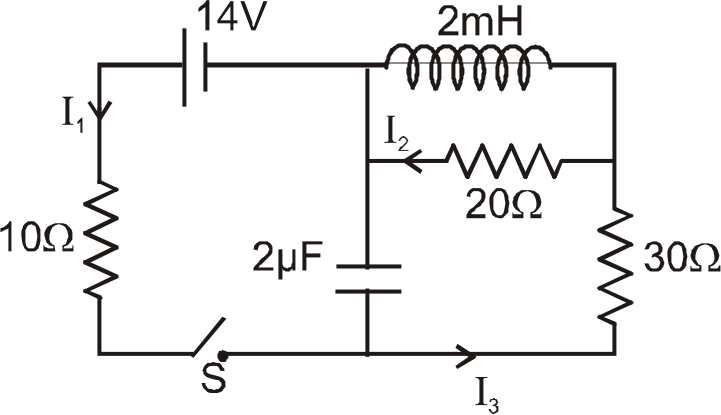 A1.4A,0,0B1.4 A,0.70 A,0.70 AC0,0,0D1.2A,0.6A,0.6A
A1.4A,0,0B1.4 A,0.70 A,0.70 AC0,0,0D1.2A,0.6A,0.6A
In the circuit shown switch S is closed at t=0. Let i1 and i2 be the current at any finite time t then the ratio i1i2
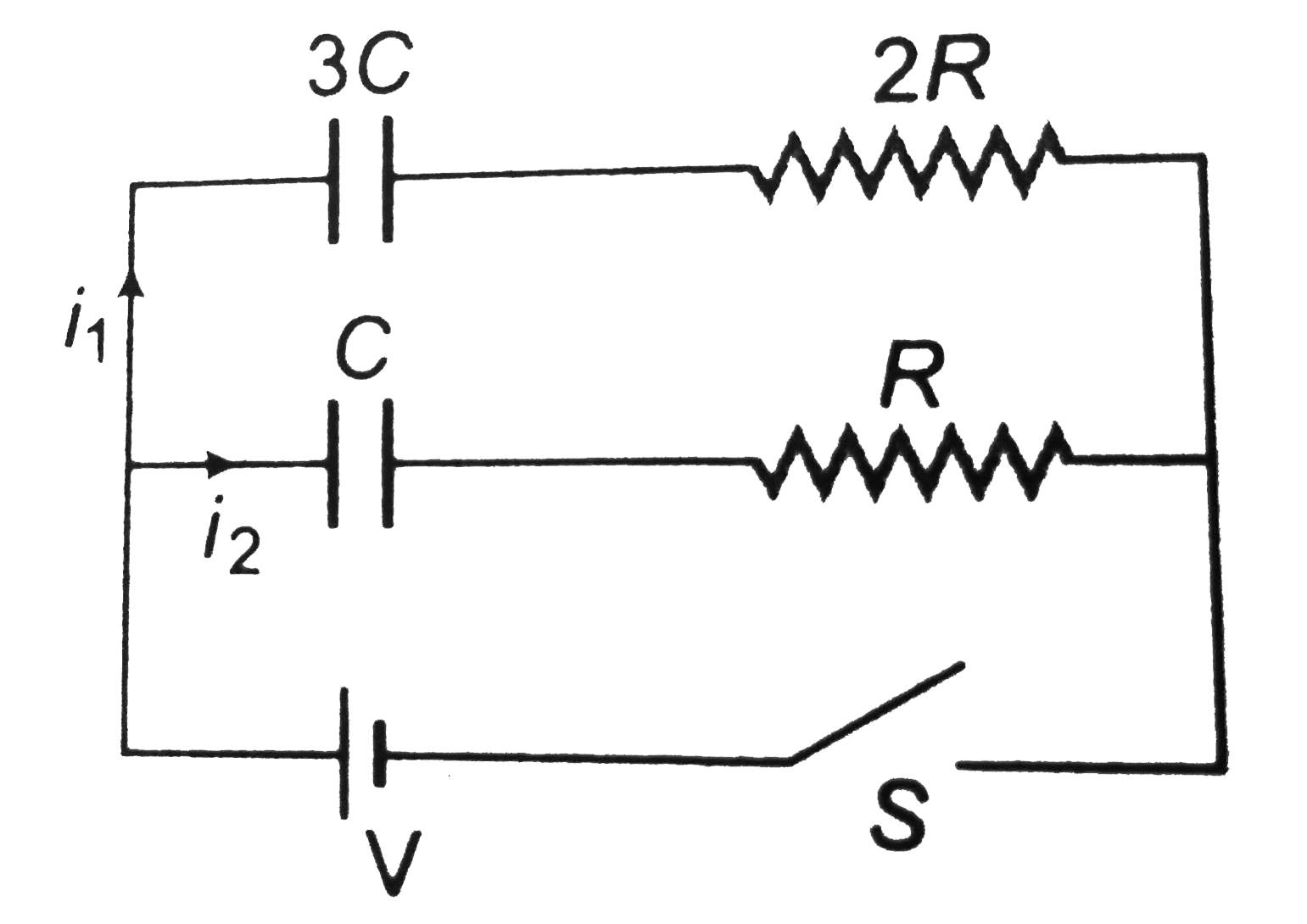 View Solution
View SolutionIn the circuit shown in figure switch S is closed at time t=0
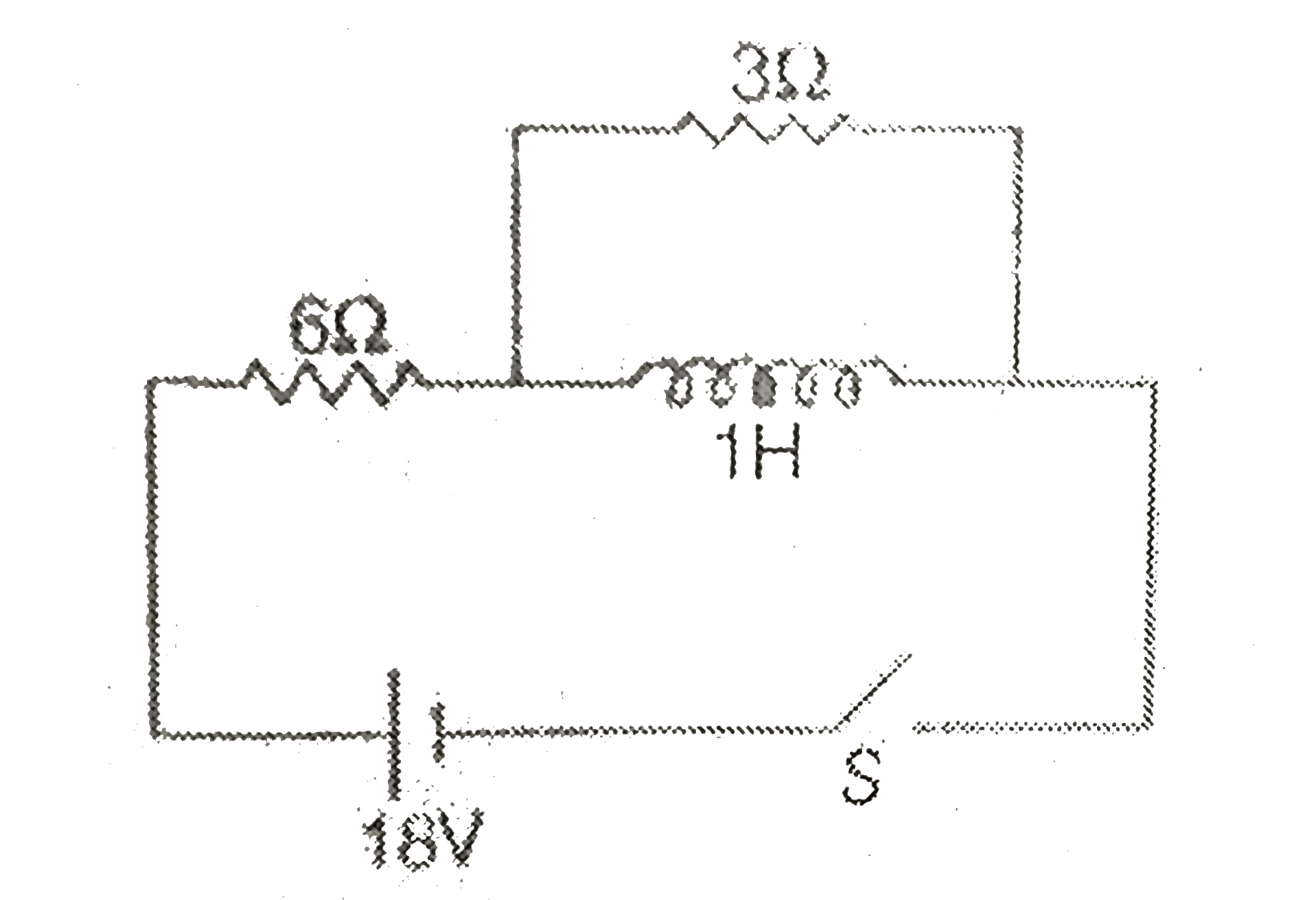
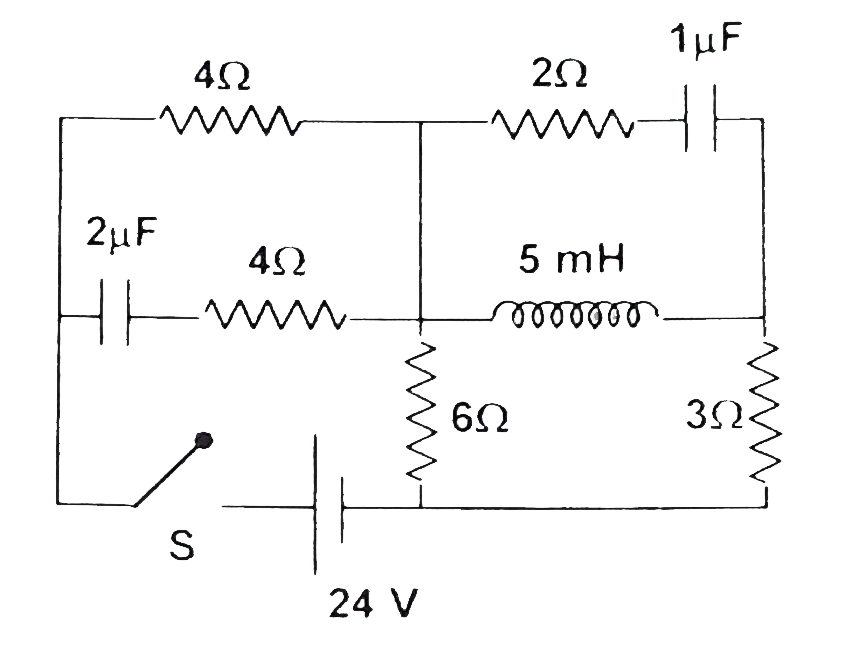
Current I from the battery at time t is given byView SolutionIn the circuit shown, the switch 'S' is closed at t=0. Then the current through the battery steady state reached is
 View Solution
View SolutionIn the circuit shwon in fig. switch S is closed at time t = 0. Let I1andI2 be the currents at any finite time t, then the ratio I1/I2
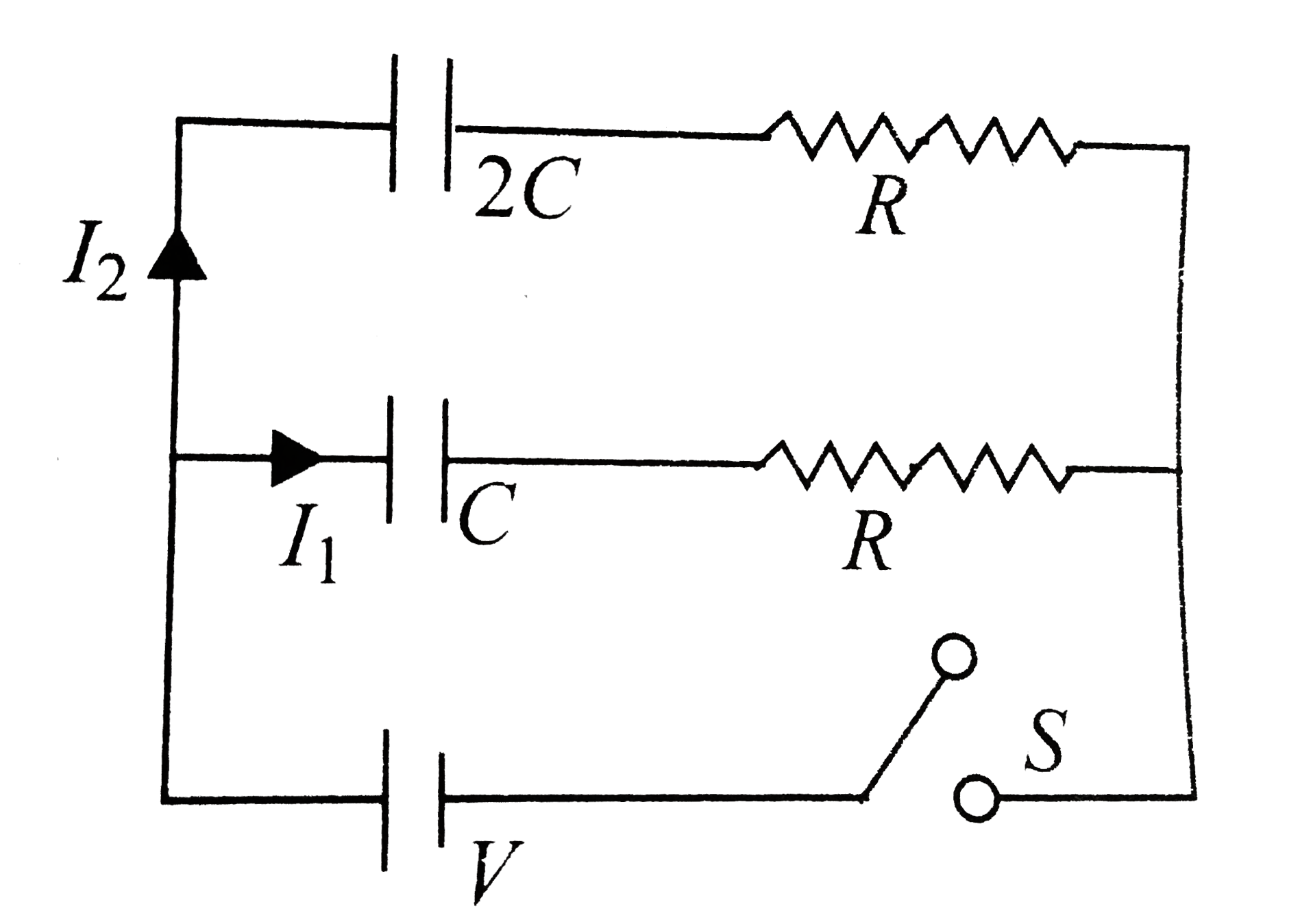 View Solution
View SolutionIn the circuit shown in figure L=10H,R=5Ω,E=15V. The switch S is closed at t=0. At t=2s the current in the circuit is
 View Solution
View Solution


_PHY_E06_157_Q01.png)
