There is a uniform sphere of mass M and radius R. Find the strength G and the potential varϕ of the gravitational field of this sphere as a function of the distance r from its centre (with r<R and r>R). Draw the approximate plots of the functions G(r) and φ(r).
We have obtained φ and G due to a uniform sphere, at a distance r from it's centre outside it.
φ=−γmMr and →G=−γMr3→r (A)
Accordance with the Eqs. (1) of the solution, potential due to a spherical shell of radius a, at any point, inside it becomes
φ=γMa=Const. and Gr=−δφδr=0 (B)
For a point (say P) which lies inside the uniform solid sphere, the potential φ at that point may be represented as a sum.
φinside=φ1+φ2
where φ1 is the potential of a solid sphere having radius r and φ2 is the potential of the layer of radii r and R. In accordance with equation (A)
φ1=−γr(M(4/3)πR343πr3)=−γMR3r2
The potential φ2 produced by the layer (thick shell) is the same at all points inside it. The potential φ2 is easiest to calculate, for the point positioned at the layer's centre. Using Eq. (B)
φ2=−γR∫rdMr=−32γMR3(R2−r2)
where dM=M(4/3)πR34πr2dr=(3MR3)r2dr
is the mass of a thin layer between the radii r and r+dr.
Thus φinside=φ1+φ2=(γM2R)(3−r2R2) (C)
From the Eq. Gr=−δφδr
Gr=γMrR3
or →G=−γMR3→r=−γ43πρ→r
(where ρ=M43πR3, is the density of the sphere) (D)
The plots φ(r) and G(r) for a uniform sphere of radius R are shown in figure of ansersheet.
Alternate: Like Gauss's theorem of electrostatics, one can derive Gauss's theorem for gravitation in the form ∮→G⋅d→S=−4πγminclosed. For calculation of →G at a point inside the sphere at a distance r from its centre, let us consider a Gaussian surface of radius r, Then,
Gr4πr2=−4πγ(MR3)r3 or, Gr=−γMR3r
Hence, →G=−γ⋅MR3→r=−γ43πρ→r (as ρ=M(4/3)πR3)
So, φ=∞∫rGrdr=R∫r−γMR3rdr+∞∫R−γMr2dr
Integrating and summing up, we get,
φ=−γM2R(3−r2R2)
And from Gauss's theorem for outside it:
Gr4πr2=−4πγM or Gr=−γMr2
Thus φ(r)=∞∫rGrdr=−γMr
Topper's Solved these Questions
- PHYSICAL FUNDAMENTALS OF MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Dynamics Of A Solid Body
56 Videos
- PHYSICAL FUNDAMENTALS OF MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Elastic Deformation Of Asolid Body
25 Videos
- PHYSICAL FUNDAMENTALS OF MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Laws Of Conservation Of Energy, Momentum And Angular Momentum
82 Videos
- OSCILLATIONS AND WAVESBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Electromagnetic Waves, Radiation
36 Videos
- THERMODYNAMICS AND MOLECULAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - THERMODYNAMICS AND MOLECULAR PHYSICSEXERCISE - Transport Phenomena
38 Videos
Similar Questions
A uniform sphere has a mass M and radius R. Find the pressure p inside the sphere, caused by gravitational compression, as a function of the distance r from its centre. Evaluate p at the centre of the Earth, assuming it to be a uniform sphere.
View SolutionA uniform sphere has a mass M and radius R. Find the pressure p inside the sphere, caused by gravitational compression, as a function of the distance r from its centre. Evaluate p at the centre of the Earth, assuming it to be a uniform sphere.
View Solution
Knowledge Check
Two spheres each of mass M and radius R are separated by a distance of r. The gravitational potential at the midpoint of the line joining the centres of the spheres is
A−GMrB−2GmrC−GM2rD−4GMrTwo spheres each of mass M and radius R are separated by a distance of r. The gravitational potential at the midpoint of the line joining the centres of the spheres is
A−GMrB−2GMrC−GM2rD−4GMr
From a solid sphere of mass M and radius R, a solid sphere of radius R/2 is removed as shown. Find gravitational force on mass m as shown
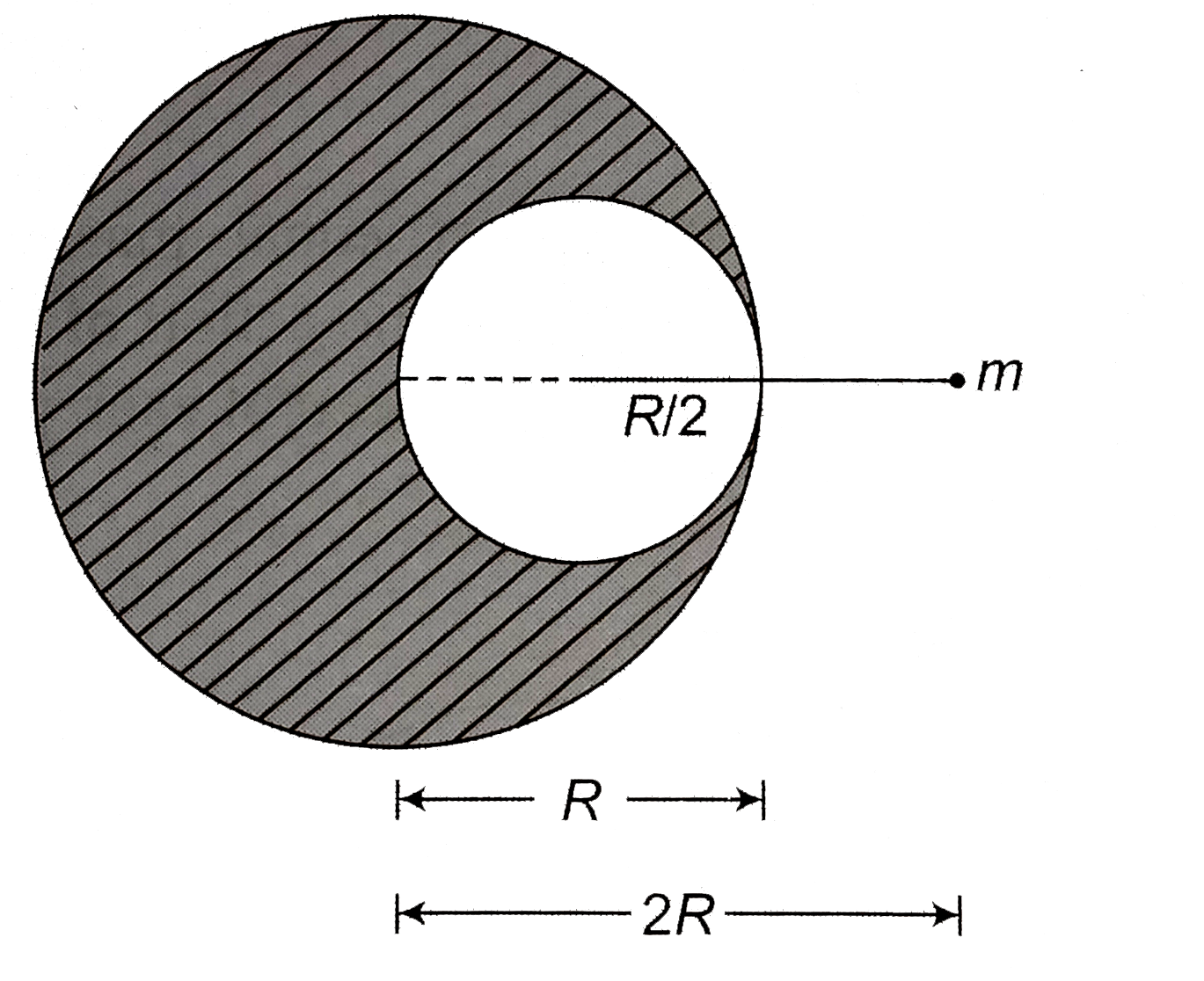
View SolutionTwo spheres each of mass M and radius R are separated by a distance of r. The gravitational potential at the midpoint of the line joining the centres of the spheres is
View SolutionA sphere of radius 2R and mas M has a spherical cavity of radius R as shown in the figure. Find the value of gravitational field at a point P at a distance of 6R from centre of the sphere.

View SolutionA sphere of radius 2R and mas M has a spherical cavity of radius R as shown in the figure. Find the value of gravitational field at a point P at a distance of 6R from centre of the sphere.

View SolutionFind the gravitational potential due to a spherical shell of mass M and radius R at r<R and r>R, where r is the distance from the centre of the shell.
View SolutionFind the gravitational potential due to a spherical shell of mass M and radius R at r<R and r>R, where r is the distance from the centre of the shell.
View Solution
