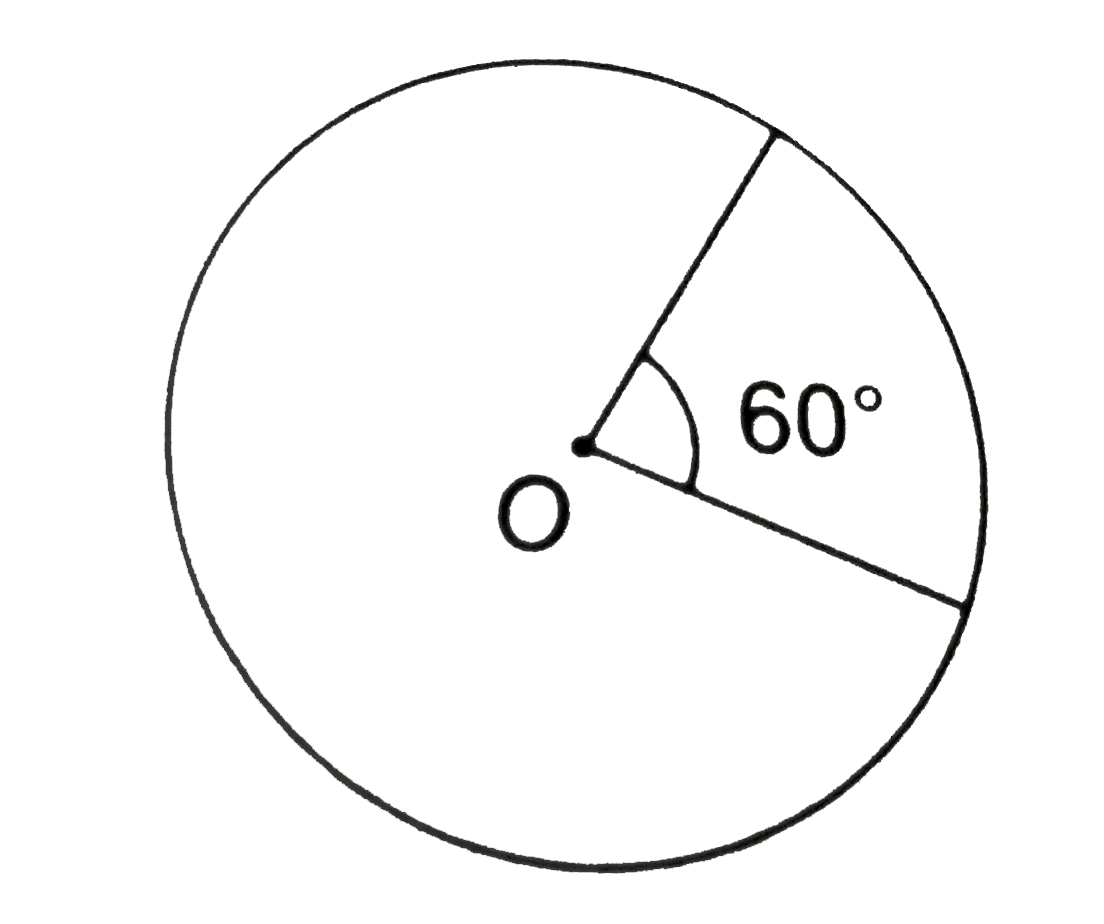
From a circular disc of mass M and radius R, a part of 60^(@) is remov

The moment of inertia of a circular disc about an axis passing through the circumstances perpendicular to the plane of the disc is
A $(M R^{2}$
B $\frac{3}{2} M R [^(2)$
C $\frac{M R^{2}}{2}$
D $\frac{5}{4} M R^{2}$
Radius of gyration of a uniform circular disc about an axis passing through its centre of gravity and perpendicular to its plane is
AR
B $\frac{R}{2}$
C $\sqrt{2} R$
D $\frac{R}{\sqrt{2}}$
The radius of of disc is 2 m the radius of gyration of disc about an axis passing through its diameter is
A2 m
B2 cm
C1 m
D0.2 m

From a circular disc of mass $M$ and radius $R$ , a part of $60^{\circ}$ is removed. The $M . I .$ of the remaining portion of disc about an axis passing through the center and perpendicular to plane of disc is