Block A and C starts from rest and move to the right with acceleration $a_{A} = 12 t m s^{- 2}$ and $a_{C} = 3 m s^{- 2}$ . Here t is in second. The time when block B again comes to rest is

A

$2 s$

B

$1 s$

C

$3 / 2 s$

D

$1 / 2 s$

Video Solution

Text Solution

Verified by Experts

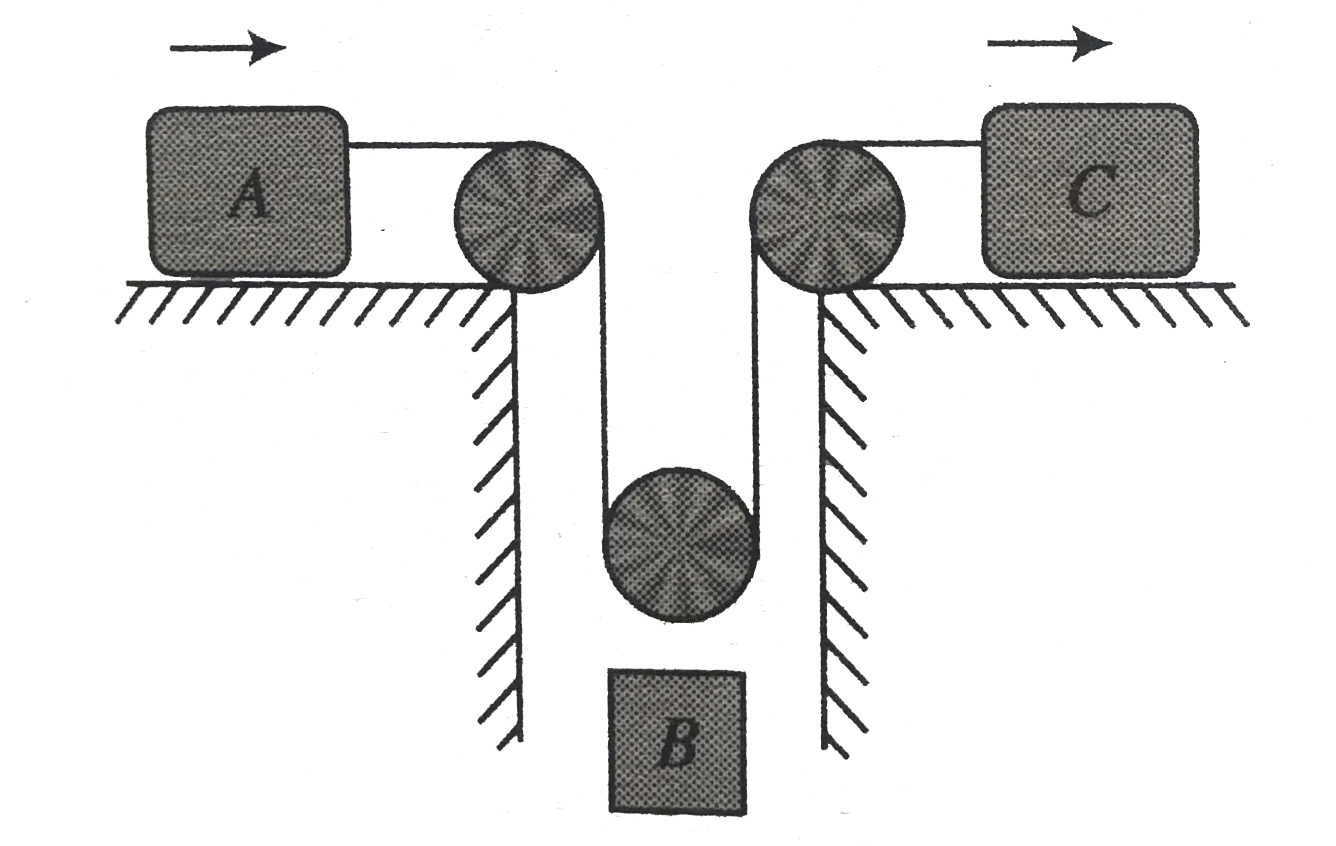
The correct Answer is:D

From constraint relation we can see that acceleration of block $B$ is $a_{B} = (\frac{a_{A} + a_{C}}{2})$ with proper signs. Hence $a_{B} = (\frac{3 - 12 t}{2}) = 1.5 - 6 t or$
$\frac{d v_{B}}{d t} = 1.5 - 6 or \underset{0}{\int^{v_{B}}} d v_{B} = \underset{0}{\int^{t}} (1.5 - 6 t) d t$ or
$v_{B} = 1.5 t - 3 t^{2}$ or $v_{B} = 0$ at $= \frac{1}{2} s$ .

Updated on:21/07/2023

|

Class 11 PHYSICS NEWTONS LAW OF MOTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Block A and C start from rest and move to the right with acceleration $a_{A} = 12 t m / s^{2}$ and $a_{C} = 3 m / s^{2}$ Here t is in seconds. The time when block B attain comes to rest is:

A2s
B1s
C $3 / 2 s$
D $1 / 2 s$
Blocks $m_{1}$ and $m_{2}$ starts from rest and move to right with accelerations as shown. If $t$ is in second then the time when block $m_{3}$ again come to rest from $t = 0$ is (pulley and strings are ideal) :
.
A $\frac{\sqrt{5}}{2} sec$
B $\frac{1 + \sqrt{5}}{2} sec$
C $\frac{1 + \sqrt{3}}{2} sec$
D $1 sec$
Two particles A and B start from rest at the origin $x = 0$ and move along a straight line such that $a_{A} = (6 t - 3) m s^{- 2}$ and $a_{B} = (12 t^{2} - 8) m s^{- 2}$ where t is in seconds based on the above facts answer the following questions,
Total distance travelled by B at $t = 4$ s is
A192 m
B184 m
C196 m
D200m

NARAYNA-NEWTONS LAWS OF MOTION-SINGLE ANSWER QUESTIONS

Home
Ask Doubt
Profile