Initially the blocks are at rest with $F = 0$ . $F$ is gradually increased.

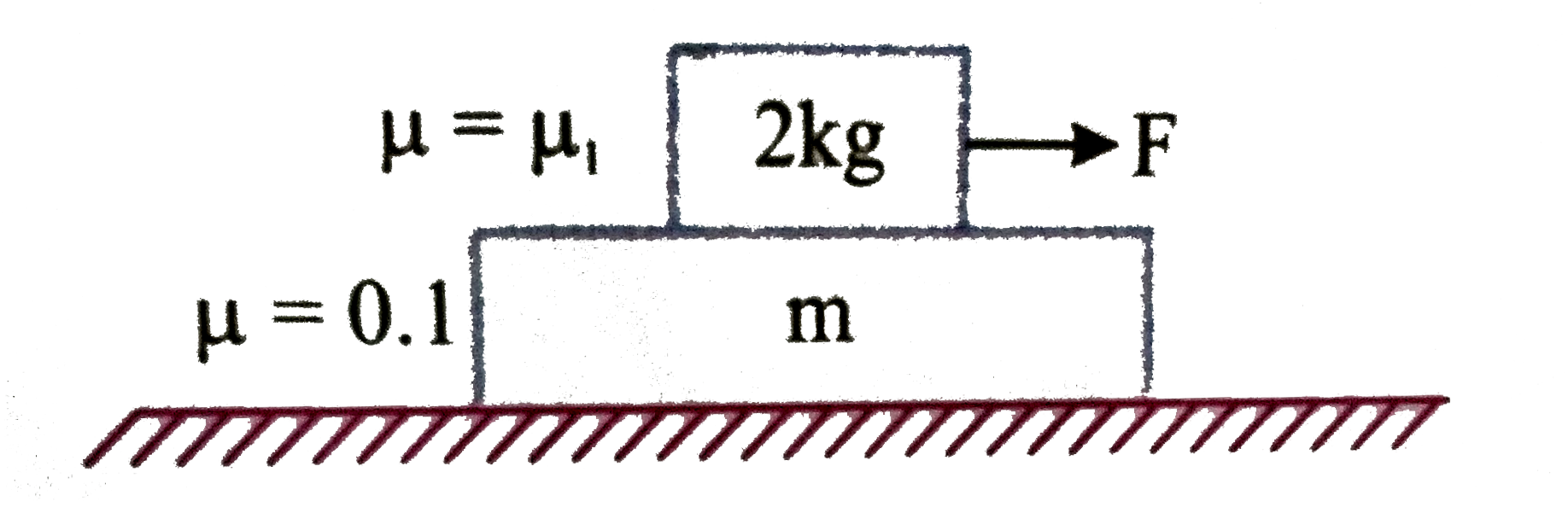
From $F = 0$ till $F = F_{1}$ , no motion
From $F > F_{1}$ till $F = 2 F_{1}$ , motion with relative acceleration $= 0$
From $F > 2 F_{1}$ , relative acceleration non-zero
At $F = 3 F_{1}$ , relative acceleration $= 2 m s^{- 2}$
Then,

A

$m = 2 K g$

B

$μ_{1} = 0.4$

C

$F_{1} = 6 N$

D

At $F = 4 F_{1}$ , relative acceleration is $4 m s^{- 2}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A, D

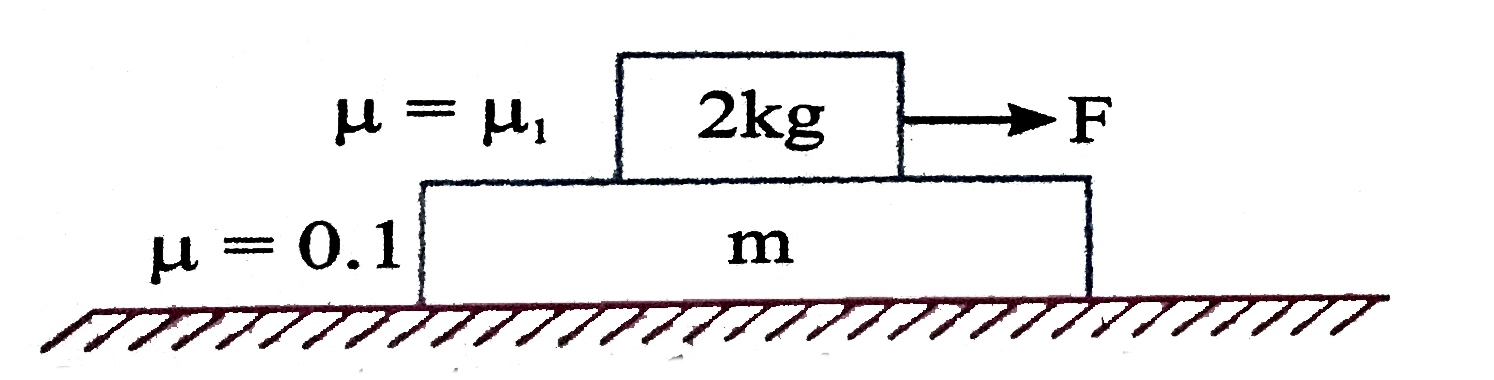
System starts accelerating when $F = F_{1}$
$F_{1} = 0.1 (m + 2) g$ …(i)
$F_{1} \leq F \leq 2 F_{1}$ no relative acceleration, a is common accceleration when $F = 2 F_{1}$
$2 F_{1} - μ_{1} 2 g = 2 a \dots . (i i) 2 F_{1} - μ_{1} 2 g = 2 a . . (i i i)$
$F = 3 F_{1}$ relative acceleration is $2 m / \sec^{2}$
$\frac{3 F_{1} - μ_{1} 2 g}{2} - a = 2$ ...(iv) solving equations
$F_{1} = 4, m = 2, a = 1, μ = 0.3$
$F = 4 F_{1}$ relative acceleration is $\frac{4 F_{1} - μ_{1} 2 g}{2} - a$
putting values relative acceleration is $4 m s^{- 2}$

|

Updated on:21/07/2023

Class 11 PHYSICS FRICTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A force $F_{1}$ acts on a particle so as to accelerate it from rest to a velocity v. The force $F_{1}$ is then replaced by $F_{2}$ which decelerates it to rest
A $F_{1}$ must be equal to $F_{2}$
B $F_{1}$ may be equal to $F_{2}$
C $F_{1}$ must be unequal to $F_{2}$
Dnone of these
Question 2 - Select One
For $r > 0, f (r)$ is the ratio of perimeter to area of a circle of radius r. Then f(1) + f(2) is equal to
A1
B2
C3
D4
Question 3 - Select One
When forces $F_{1}$ , $F_{2}$ , $F_{3}$ are acting on a particle of mass m such that $F_{2}$ and $F_{3}$ are mutually prependicular, then the particle remains stationary. If the force $F_{1}$ is now rejmoved then the acceleration of the particle is
A $F_{1} / m$
B $F_{2} F_{3} / m F_{1}$
C $(F_{2} - F_{3}) / m$
D $F_{2} / m$

NARAYNA-FRICTION-Multiple answer question

Home
Ask Doubt
Profile