The distance of centre of mass from O is
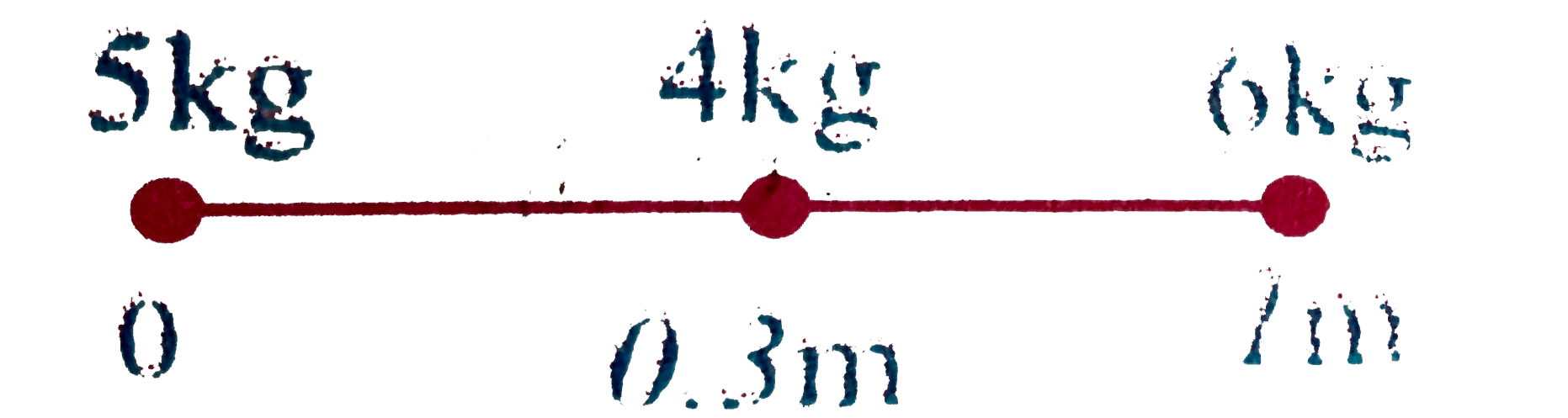
0.21m
0.35m
0.42m
0.48m
The correct Answer is:D
xcm=m1x1+m2x2m1+m2
Topper's Solved these Questions
- SYSTEM OF PARTICLESBook:NARAYNAChapter:SYSTEM OF PARTICLESExercise:Level -II(H.W)
Explore 47 Videos
- SYSTEM OF PARTICLESBook:NARAYNAChapter:SYSTEM OF PARTICLESExercise:Level-V
Explore 72 Videos
- SYSTEM OF PARTICLESBook:NARAYNAChapter:SYSTEM OF PARTICLESExercise:NCERT based questions
Explore 15 Videos
- PHYSICAL WORLDBook:NARAYNAChapter:PHYSICAL WORLDExercise:C.U.Q
Explore 10 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTION Book:NARAYNAChapter:SYSTEM OF PARTICLES AND ROTATIONAL MOTION Exercise:EXERCISE - IV
Explore 39 Videos
Similar Questions
The distance of centre of mass from point O of two square plates system, as shown,( if masses of plates are 2m and m and their edges are 'a' and '2a' respectively) is

View SolutionFor a group of particles of different masses,if all the masses are placed at different points at equal distance R from the origin, then the distance of the centre of mass from the origin will be
View Solution
Knowledge Check
A uniformly thick plate in the shape of an arrow head has dimensions as shown. Find the distance of centre of mass from point O (in cm)
 A1B2C3D4
A1B2C3D4A thin uniform rod of length L is bent at its mid point as shown in the figure. The distance of the centre of mass from the point O is
 AL2sin(θ2)BL2cos(θ2)CL4sin(θ2)DL4cos(θ2)
AL2sin(θ2)BL2cos(θ2)CL4sin(θ2)DL4cos(θ2)A uniform rod o length one meter is bent at its midpoint to make 90∘. The distance of centre of mass from the centre of rod is (in cm)
A20.2B13.4C15D17.6
In the figure one fourth part of a uniform disc of radius R is shown. The distance of the centre of mass of this object from centre 'O' is :
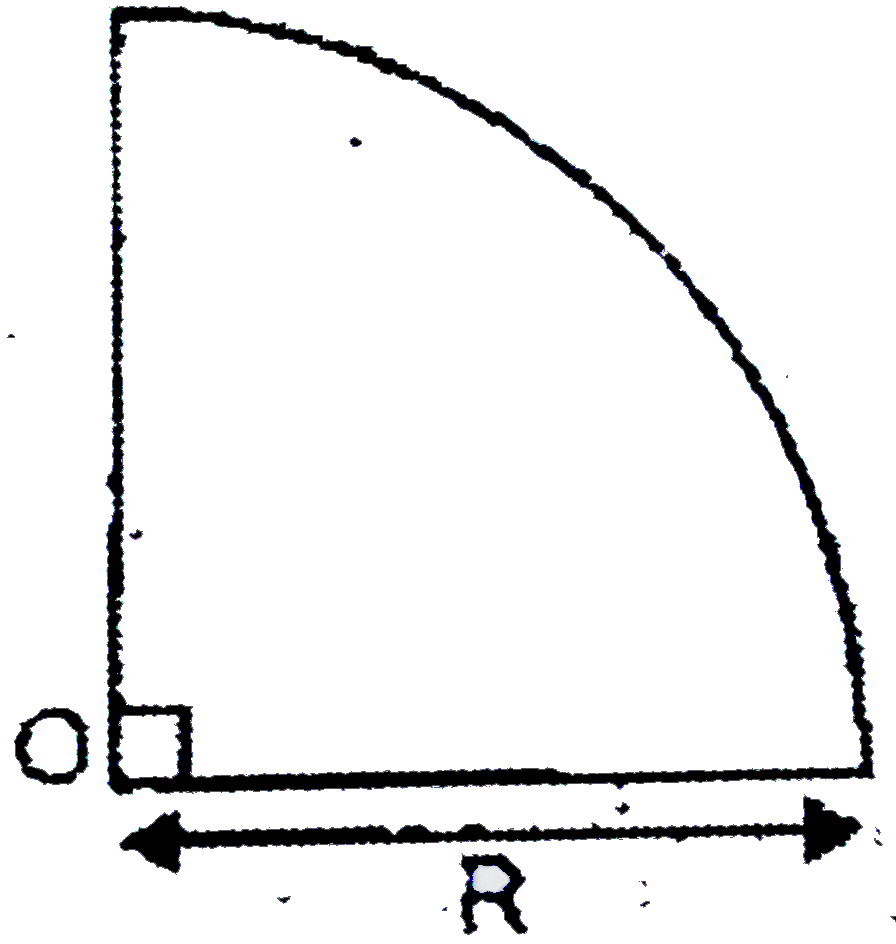
View SolutionThe distance of the centre of mass of a hemispherical shell of radius R from its centre is
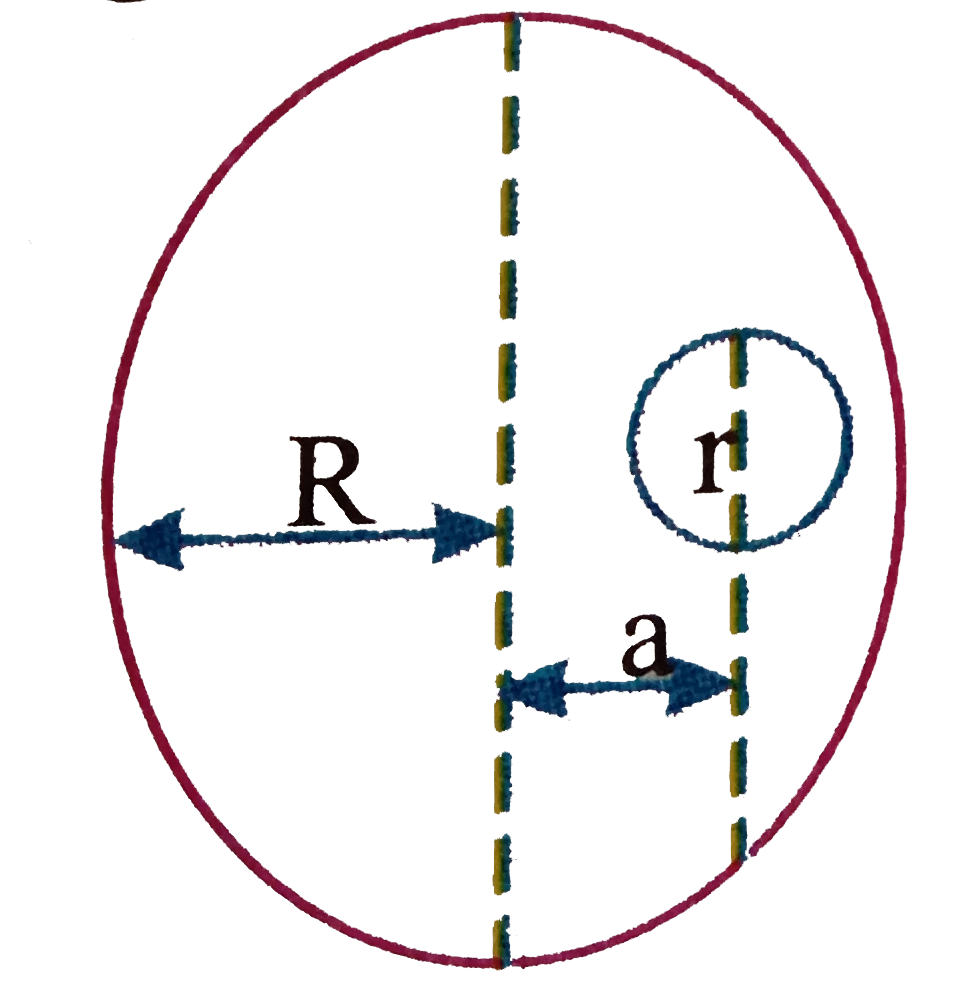
View SolutionA circular hole of radius r is made in a disk of radius R and of uniform thickness at a distance a from the centre of the disk. The distance of the new centre of mass from the original centre of mass is
View SolutionIf the distance between two point masses m1 and m2 is d, then distance of its centre of mass from m2 will be mod
View SolutionIn the figure shown a hole of radius 2 cm is made in a semicircular disc of radius 6π at a distance 8 cm from the centre C of the disc. The distance of the centre of mass of this system from point C is :
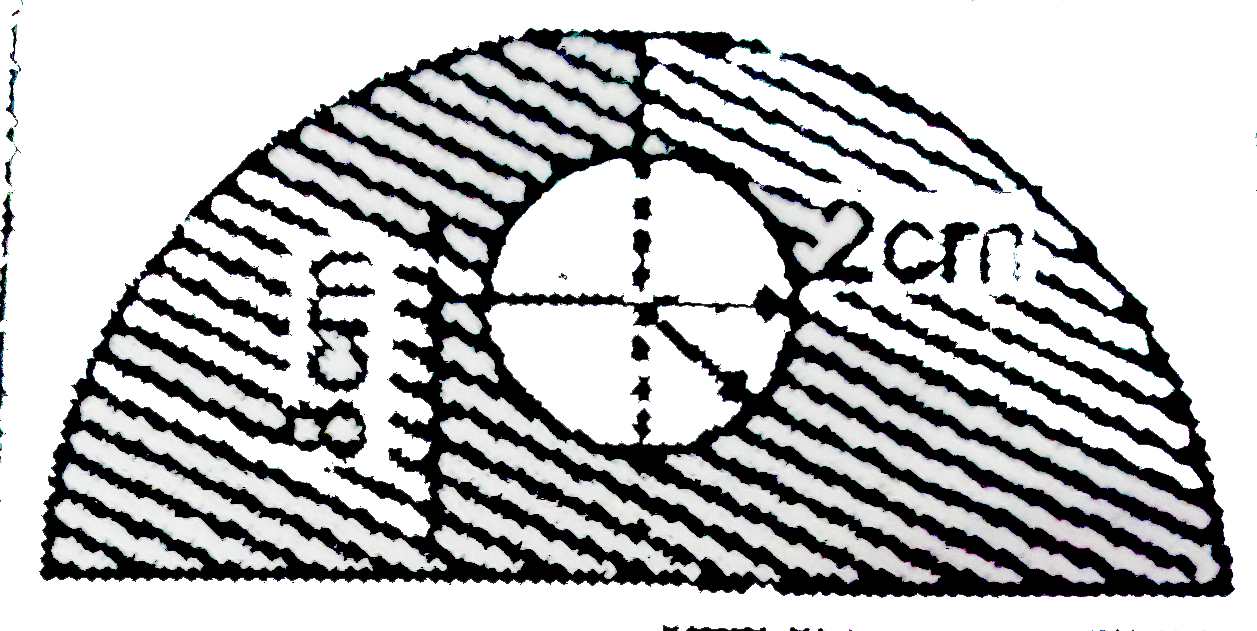
View Solution