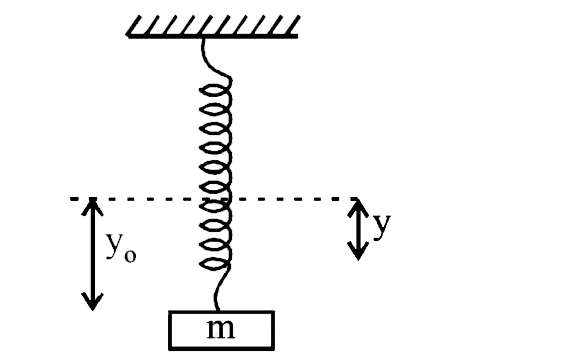
A small body attached to one end of a vertically hanging spring is performing (SHM) about is mean position with angular frequency (omega) and amplitude (a). If at a height (y) from the mean position, the body gets detached from the spring, calculate the value of (y) so that the height (H) attained by the mass is maximum. The body does noy interact with the spring during its subsquent motion after detachment. $(a ω^{2} > g)$ .
.

A

$\frac{g}{ω^{2}}$

B

$\frac{2 g}{ω^{2}}$

C

$\frac{3 g}{ω^{2}}$

D

$\frac{4 g}{ω^{2}}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

At distance $y$ above then mean position velocity of the block. $v = w \sqrt{A^{2} - y^{2}}$ . After detaching from the spring net downwrd acceleration of the block will be $g$ . Therefore, total height attained by the block above the mean position.
$h = y + \frac{v^{2}}{2 g} = y + \frac{ω^{2} (A^{2} - y^{2})}{2 g}$
For $h$ to be maximum $\frac{d h}{d y} = 0$
Putting $\frac{d h}{d y} = 0$ , we get $y = \frac{g}{ω^{2}}$

|

Updated on:21/07/2023

Class 11 PHYSICS SIMPLE HARMONIC MOTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A light body is hanging at the lower end of a vertical spring . On passing current in the spring, the body
Arises up
Bgoes down
Cno change
Doscillates up & down
Question 2 - Select One
A body executes SHM with an amplitude a. At what displacement from the mean positions, the potentail energy of the body is one-fourth of its total energy?
A $\frac{a}{4}$
B $\frac{a}{2}$
C $\frac{3 a}{4}$
DSome other fraction of $a$
Question 3 - Select One
A body performing SHM about its mean position with period 24 s, after 4 s its velocity is $π m / s$ , then its path will be
A48 m
B24 m
C52 m
D12 m

NARAYNA-OSCILLATIONS-SINGLE ANSWER QUESTIONS

Home
Ask Doubt
Profile