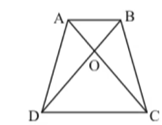
ABCD is a trapezium having ABDC . Prove that O , the point of intersection of diagonals, divides the two diagonals in the same ratio. Also prove that ar(OCD)ar(OAB)=19 , if AB=3 CD .
Step by step video & image solution for A B C D is a trapezium having A B D C . Prove that O , the point of intersection of diagonals, divides the two diagonals in the same ratio. Also prove that (a r( O C D))/(a r( O A B))=1/9 , if A B=3\ C D . by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
ABCD is a trapezium having ABDC . Prove that O , the point of intersection of diagonals, divides the two diagonals in the same ratio. Also prove that ar(OCD)ar(OAB)=19 , if AB=3 CD .
View SolutionO is any point on the diagonal BD of the parallelogram ABCD. Prove that ar(OAB)=ar(OBC)
View SolutionIn Figure, ABCD is a trapezium in which AB∣∣CD . Prove that: ar(AOD)=ar(BOC).
View SolutionD is the mid-point of side BC of ABC and E is the mid-point of BD. If O is the mid-point of AE , prove that ar(BOE)=18ar(ABC)
View SolutionIn figure ABC and DBC are two triangles on the same base BC. If AD intersects BC at O, show that ar(ABC)ar(DBC)=AODO.

View SolutionABCD is a kite having AB=AD and BC=CD . Prove that the figure formed by joining the mid-points of the sides, in order, is a rectangle.
View SolutionD is the mid-point of side BC of ABC and E is the mid-point of BD. If O is the mid-point of AE, prove that ar ( BOE)=18ar ( ABC)
View SolutionA point D is taken on the side BC of a ABC such that BD=2dC. Prove that ar(ABD)=2ar(ADC).
View SolutionABCD is a parallelogram whose diagonals intersect at O. If P is any point on BO, prove that: ar (ADO)=AR (CDO) ar ( ABP)= ar ( CBP)
View SolutionABCD is a trapezium with ABDC. A line parallel to AC intersects AB at X and BC at Y. Prove that ar(ADX)=ar(ACY).
View Solution
