A box and a solid sphere of equal mass are moving with the same velocity across a horizontal floor. The sphere rolls without slipping and the box without friction. They ecounter an upward slope in the floor and each move up the slope some distance before momentarily stopping and then moving downn again. The maximum vertical heights reached by the box and the sphere are HB and Hs respectively. What is the ratio 5HsHB ?

The correct Answer is:7
mgHB=12mv2
mgHs=12mv2+12Iω2=12mv2+12×12mr2ω2=12×75mv2
HsHB=75⇒5HsHB=7
Topper's Solved these Questions
- TEST PAPERSBOOK - FIITJEECHAPTER - TEST PAPERSEXERCISE - PHYSICS
747 Videos
- SIMPLE HARMONIC MOTIONBOOK - FIITJEECHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Assignment Problems (Objective) (Level-II)
20 Videos
- WORK, ENERGY AND POWER BOOK - FIITJEECHAPTER - WORK, ENERGY AND POWER EXERCISE - COMPREHENSIONS ( Comprehension-II)
5 Videos
Similar Questions
Two uniform solid spheres having unequal radii are released from rest from the same height on a rough incline. If the spheres roll without slipping
View SolutionTwo uniform solid spheres having unequal radii are released from rest from the same height on a rough incline. the spheres roll without slipping
View Solution
Knowledge Check
Two uniform solid spheres having unequal rdii are released from rest from the same height on a rough incline. Ilf the spheres roll without slipping
Athe heavier sphere reaches the bottom firstBthe bigger sphere reaches the bottom firstCthe two spheres reach the bottom togetherDthe information given is not sufficient to tell which sphere will reach the bottom firstA solid sphere is thrown up a rough incline. The sphere rolls up without slipping and eventually comes down rolling without slipping. The direction of friction during upward and downward motion respectively is :-
ADownward, upwardBUpward, downwardCDownward, downwardDUpward, upwardA solid sphere of mass m rolls without slipping on an inclined plane of inclination θ. The linear acceleration of the sphere is
A75gsinθB27gsinθC37gsinθD57gsinθ
A solid sphere is thrown up a rough incline. The sphere rolls up without slipping and eventually comes down rolling without slipping. The direction of friction during upward and downward motion respectively is :-
View Solution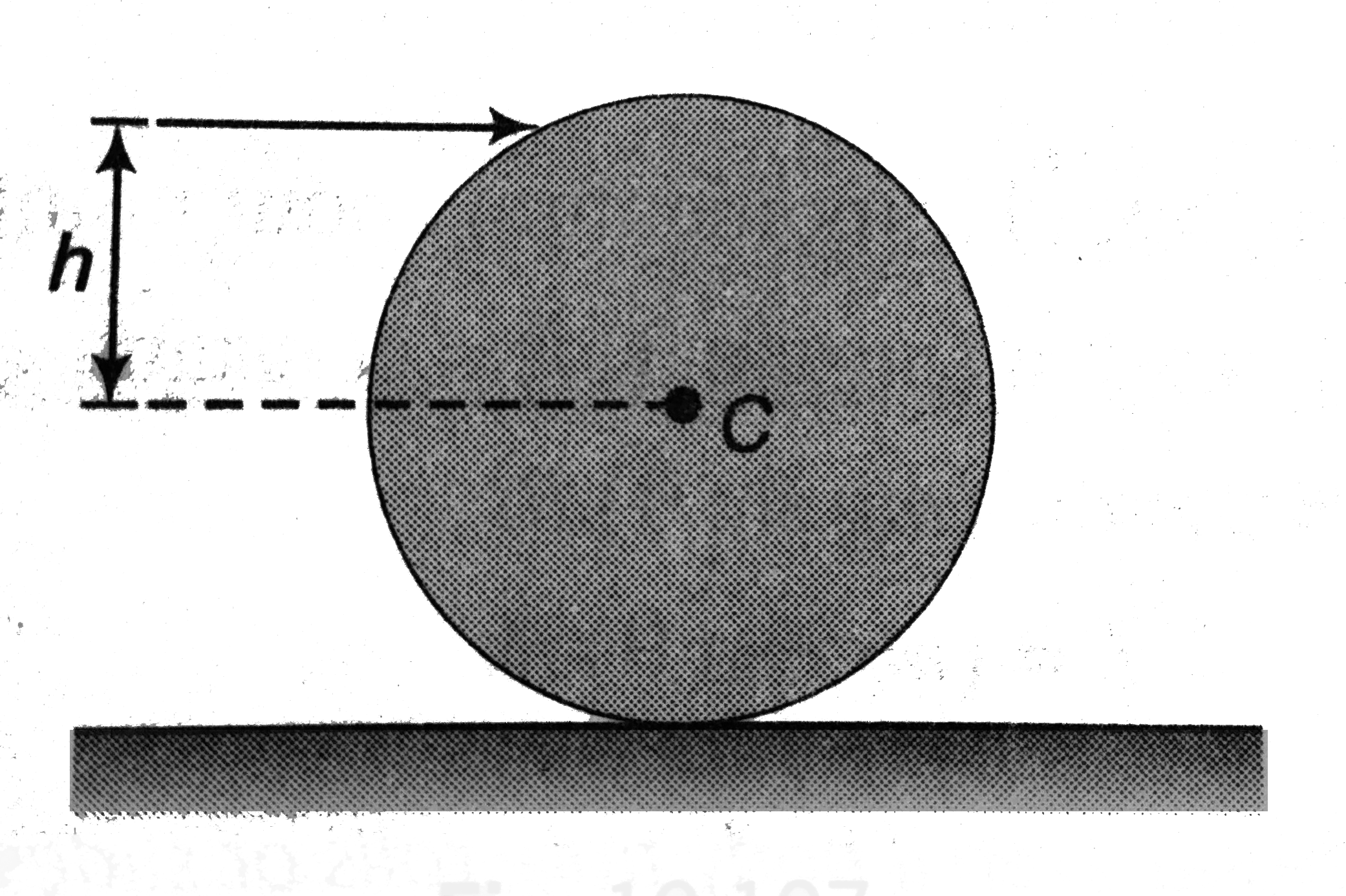
A solid sphere of mass M and radius R is hit by a cue at a height h above the centre C. for what value of h the sphere will rool without slipping ?
View Solution
A solid sphere of mass M and radius R is hit by a cue at a height h above the centre C. for what value of h the sphere will rool without slipping ?
View SolutionA solid sphere of mass m rolls without slipping on an inclined plane of inclination θ. The linear acceleration of the sphere is
View SolutionIf a solid sphere of mass 500 gram rolls without slipping with a velocity of 20 cm/s, then the rolling kinetic energy of the sphere will be
View Solution
