A small insect is climbing slowly along the inner wall of a hemispherical bowl of radius R. The insect is unable to climb beyond θ=45∘. Whenever it tries to climb beyond θ=45∘, it slips.
(a) Find the minimum angular speed ω with which the bowl shall be rotated about its vertical radius so that the insect can climb upto θ=60∘.
(b) Find minimum ω for which the insect can move out of the bowl.
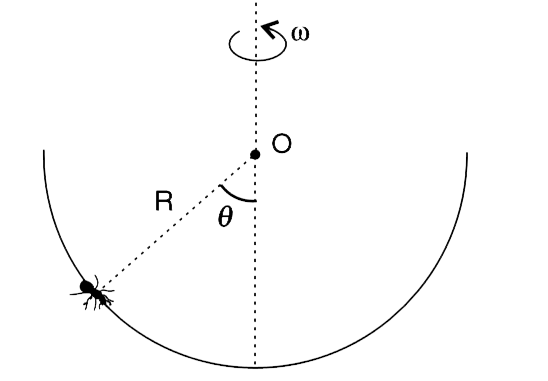
The correct Answer is:
⎷gR2(√3−1)√3(√3+1)
(b) √gR
Step by step video, text & image solution for A small insect is climbing slowly along the inner wall of a hemispherical bowl of radius R. The insect is unable to climb beyond theta = 45^(@). Whenever it tries to climb beyond theta = 45^(@), it slips. (a) Find the minimum angular speed omega with which the bowl shall be rotated about its vertical radius so that the insect can climb upto theta = 60^(@). (b) Find minimum omega for which the insect can move out of the bowl. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
Similar Questions
A particle is acted upon by a force for 1 second whose X component remains constant at Fx=30N but y and z components vary with time as shown in the graph. Calculate the magnitude of change in momentum of the particle in 1 s. What angle does the change in momentum (Δ→P) make with X axis ?
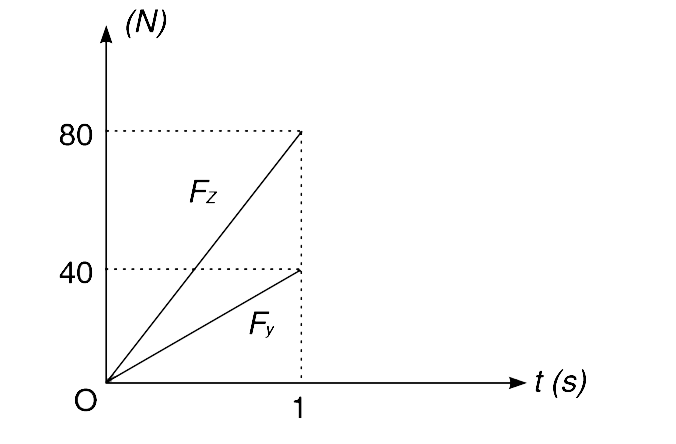 04:17View Solution
04:17View SolutionThe pulley of radius R can rotate freely about its axle as shown in the figure. A thread is tightly wrapped around the pulley and its free end carries a block of mass m. When the block is at a height h above the ground the system is released (i.e., the pulley is made free to rotate & the block is allowed to fall) and at the same instant the axle is moved up keeping it horizontal all the time. When the block hits the floor the axle has gone up by a distance 2h. Find the angle by which the pulley must have rotated by this time.
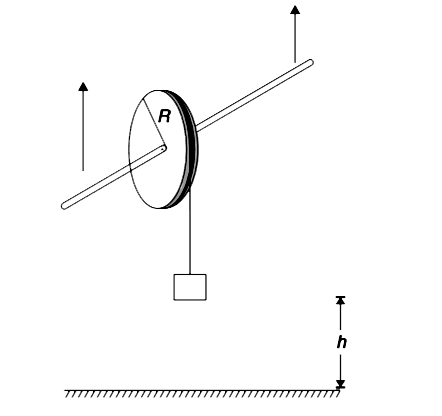 04:17View Solution
04:17View SolutionA hemispherical bowl of radius R is set rotating about its axis of symmetry which is kept vertical. A small block kept in the bowl rotates with the bowl without slipping on its surface. If the surfaces of the bowl is smooth, and the angle made by the radius through the block with the vertical is θ, find the angular speed at which the bowl is rotating.
02:34View SolutionA hemispherical bowl of radius R is set rotating about its axis of symmetry which is kept vertical A small block kept in the bowl rotates with the bowl without slipping on its surface If the surface of bowl is smooth and angle made by radius through the block with the vertical is 0 find the angular speed at which the bowl is rotating .
03:30View SolutionThe coefficient of friction between a hemispherical bowl and an insect is sqrt(0.44) and the radius of the bowl is 0.6m . The maximum height to which an insect can crawl in the bowl will be .
View SolutionIf the coefficient of friction between an insect and bowl is μ and the radius of the bowl is r, find the maximum height to which the insect can crawl in the bowl.
View SolutionThe coefficient of friction between an insect and a hemispherical bowl of radius r is mu . The maximum height to which the insect can crawl in the bowl is
View SolutionThe coefficient of friction between a hemispherical bowl and an insect is √0.44 and the radius of the bowl is 0.6m. The maximum height to which an insect can crawl in the bowl will be
View SolutionA hemispherical bowl of radius R is set rotating about its axis of symmetry which is kept vertical. A small block kept in the bowl rotates with the bowl without slipping on its surface. If the surface of bowl is smooth, and the angle made by the radius through the block with the vertical is θ, the angular speed at which the bowl is rotating is
05:04View SolutionA hemispherical bowl of radius R is set rotating about its axis of symmetry which is kept vertical. A small block kept in the bowl rotates with the bowl without slipping on its surface. If the surface of bowl is smooth, and the angle made by the radius through the block with the vertical is q, the angular speed at which the bowl is rotating is
03:23View SolutionA hemispherical bowl of radius (R) is set rotating about its axis of symmetry which is kept vertical. A small block kept in the bowl rotates with bowl without slipping on its surface. If the surface of bowl is smooth, the angle made by the radius through the block with the vertical is θ. the angular speed at which the bowl is rotating
View SolutionA hemispherical bowl of radius R si set rotating abouv its axis of symmetry whichis kept vertical. A small blcok kept in the bowl rotates with the bowl without slippingn on its surface. If the surfaces of the bowl is mooth, and the abgel made by the radius through the block with the vertical is θ, find the angular speed at which the bowl is rotating.
View SolutionA hemispherical bowl of radius R is set rotating about its axis of symmetry which is kept vertical. A small block kept in the bowl rotates with the bowl without slipping on its surface. If the surface of bowl is smooth, and the angle made by the radius through the block with the vertical is q, the angular speed at which the bowl is rotating is
03:30View SolutionA hemispherical bowl of radius R is set rotating about its axis of symmetry which is kept vertical. A small block kept in the bowl rotates with the bowl without slipping on its surface. If the surface of bowl is smooth, and the angle made by the radius through the block with the vertical is q, the angular speed at which the bowl is rotating is
05:02View SolutionIf the coefficient of friction between an insect and bowl is μ and the radius of the bowl is r, find the maximum height to which the insect can crawl in the bowl.
03:45View Solution

