Two balls of radii r and r2 are released inside a deep water tank. Their initial accelerations are found to be g2 and g4 respectively. Find the velocity of smaller ball relative to the larger ball, a long time after the two balls are released. Coefficient of viscosity is given to be η.
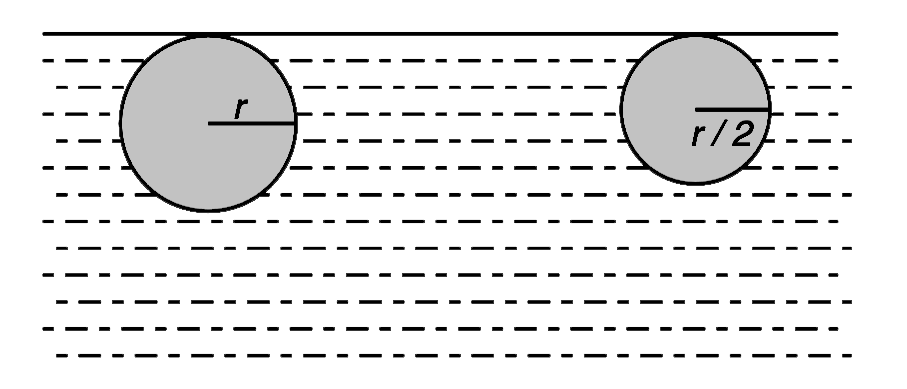
The correct Answer is:1154r2ρgeteupwards
Step by step video, text & image solution for Two balls of radii r and (r)/(2) are released inside a deep water tank. Their initial accelerations are found to be (g)/(2) and (g)/(4) respectively. Find the velocity of smaller ball relative to the larger ball, a long time after the two balls are released. Coefficient of viscosity is given to be eta. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
The focal length of a spherical mirror is given byf=R2 , where R is radius of curvature of the mirror. For a given spherical mirror made of steel the focal length is f = 24.0 cm. Find its new focal length if temperature increases by 50∘C. Given = αSteel=1.2×10−5.∘C−1
View SolutionA long taut string is plucked at its centre. The pulse travelling on it can be described as y(x,t)=e−(x+2t)2+e−(x−2t)2. Draw the shape of the string at time t=0, a short time after t=0 and a long time after t=0.
View SolutionBall 1 collides directly with another identical ball 2 at rest. Velocity of second ball becomes two times that of 1 after collison. Find the coefficient of restitution between the two balls?
View SolutionA ball is released from rest relative to the elevator at a distance h_1 above the floor. The speed of the elevator at the time of ball release is v_0 . Determine the bounce height h_2 relative to elevator of the ball (a) if v_0 is constant and (b) if an upward elevator accleration a=g/4 begins at the instant the ball is released. The coefficient of restitution for the impact is e .
View SolutionA small steel ball of mass m and radius r is falling under gravity through a viscous liquid of coefficient of viscosity eta . If g is the value of acceleration due to gravity. Then the terminal velocity of the ball is proportional to (ignore buoyancy)
View SolutionThe terminal velocity v of a small steel ball ofradius r fal ling under gravity through a column ofa viscous liquid of coefficient of viscosity η depends on mass of the ball m, acceleration due to gravity g, coefficient of viscosity η and radius r. Which of the following relations is dimensionally correct?
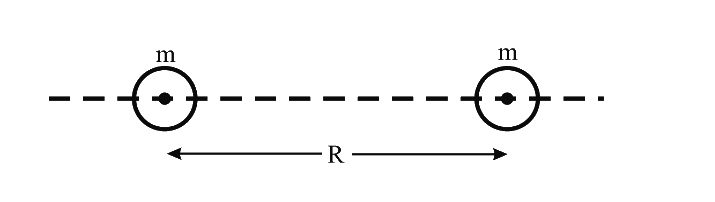
View SolutionTwo balls of radius r each are placed at a distance R and released . The speed of each ball when they collide
View SolutionA ball is thrown upward with an initial velocity of 100 ms^(-1) . After how much time will it return ? Draw velocity -time graph for the ball and find the maximum height attained by the ball. [Assume, g = - 10 ms ^ { - 2 } ]
View SolutionA ball is dropped from height h. If falls on liquid surface. Its velocity does not change when its enters in liquid. Find height h in terms of r = radius of ball, sigma = density of liquid, rho = density of ball eta = coefficient of viscosity and g = acceleration due to gravity :
View SolutionTwo balls are released from the same height at an interval of 2s. When will the separation between the balls be 20m after the first ball is released? Take g=10m//s^(2) .
View SolutionA ball is dropped from height h. If falls on liquid surface. Its velocity does not change when its enters in liquid. Find height h in terms of r = radius of ball, sigma = density of liquid, rho = density of ball eta = coefficient of viscosity and g = acceleration due to gravity :
View SolutionA small steel ball of mass m and radius r is falling under gravity through a viscous liquid of coefficient of viscosity eta . If g is the value of acceleration due to gravity. Then the terminal velocity of the ball is proportional to (ignore buoyancy)
View SolutionA ball is released from a height of 5m. How long after the release, the ball hitting the ground will stop. While the elasticity coefficient is 1/2
View SolutionBall 1 collides directly with another identical ball 2 at rest. Velocity of second ball becomes two times that of 1 after collison. Find the coefficient of restitution between the two balls?
View SolutionA ball is released from rest relative to the elevator at a distance h_1 above the floor. The speed of the elevator at the time of ball release is v_0 . Determine the bounce height h_2 relative to elevator of the ball (a) if v_0 is constant and (b) if an upward elevator accleration a=g/4 begins at the instant the ball is released. The coefficient of restitution for the impact is e .
View Solution
