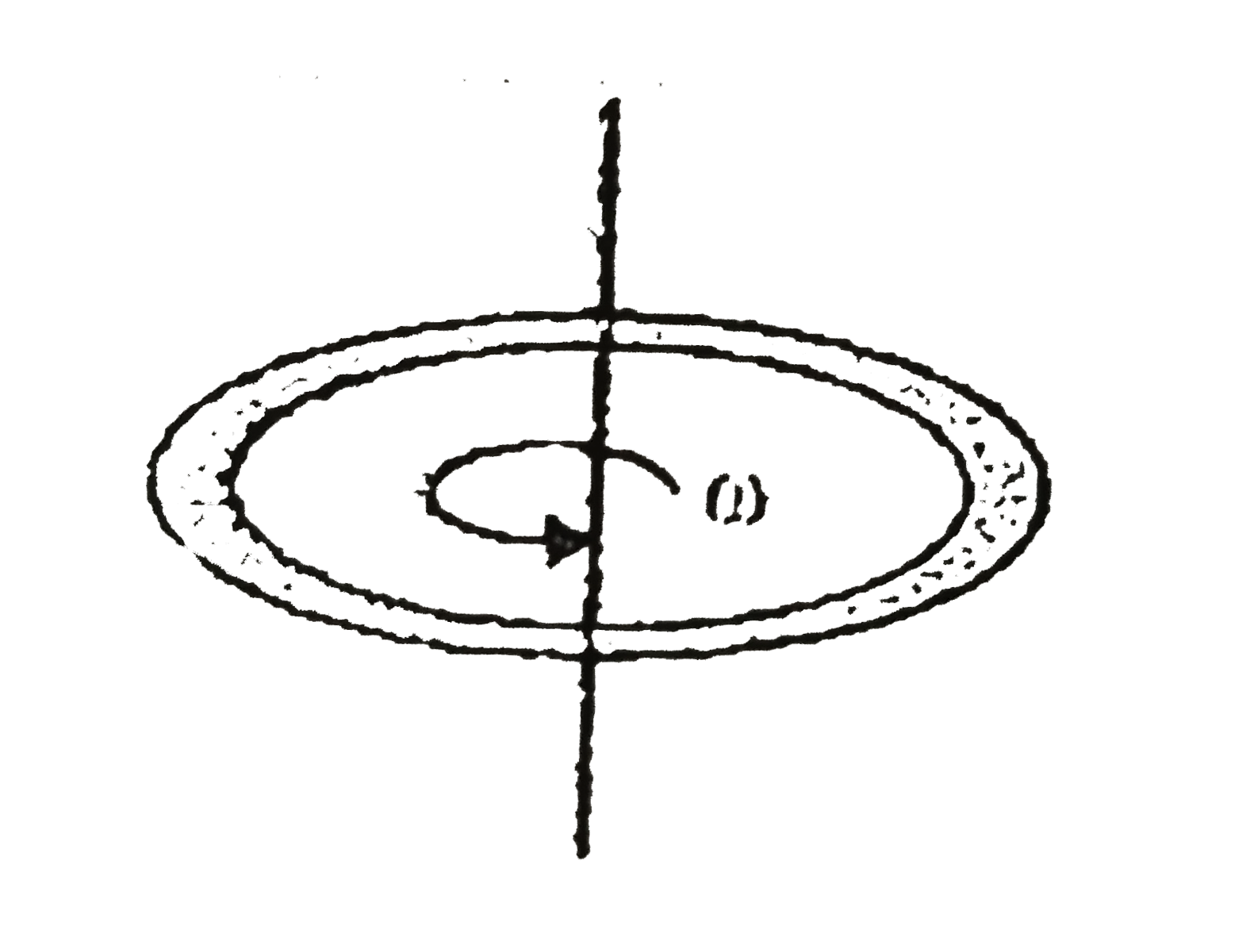
A ring is made to rotate about its diameter at a constant angular speed of ω0 . A small insect of mass m walks along the ring with a uniform angular speed ω relative to the ring (see figure). Radius of the ring is R.
(a) Find the external torque needed to keep the ring rotating at constant speed as the insect walks. Express your answer as a function of θ. For what value of q is this torque maximum? [given your answer for 0≤θ≤90∘ ]
(b) Find the component of force perpendicular to the plane of the ring, that is applied by the ring on the insect. For what value of θ is this force maximum? Argue quantitatively to show that indeed the force should be maximum for this value of θ. [Give your answer for 0∘≤θ≤90∘ ]
![A ring is made to rotate about its diameter at a constant angular speed of omega(0) . A small insect of mass m walks along the ring with a uniform angular speed omega relative to the ring (see figure). Radius of the ring is R. (a) Find the external torque needed to keep the ring rotating at constant speed as the insect walks. Express your answer as a function of theta. For what value of q is this torque maximum? [given your answer for 0 le theta le 90^(@) ] (b) Find the component of force perpendicular to the plane of the ring, that is applied by the ring on the insect. For what value of theta is this force maximum? Argue quantitatively to show that indeed the force should be maximum for this value of theta. [Give your answer for 0^(@) le theta le 90^(@) ]](https://d10lpgp6xz60nq.cloudfront.net/physics_images/IJA_PHY_V01_C06_E01_139_Q01.png)
The correct Answer is:τ=mR2ω0ωsin2θ; τ is maximum for θ=45∘
(b) F⊥=2mRω0ωcosθ;θ=0∘
Topper's Solved these Questions
Similar Questions
A ring of radius r is rotating about a vertical axis along its diameter with constant angular velocity ω.A read of mass m remains at rest w.r.t. ring at the position shown in figure. Then w2 is:
_2019_CBT8_E01_015_Q01.png)
View SolutionA ring of radius r is rotating about a vertical axis along its diameter with constant angular velocity ω.A read of mass m remains at rest w.r.t. ring at the position shown in figure. Then w2 is:
View Solution
Knowledge Check
- Question 1 - Select One
A ring of radius r is rotating about a vertical axis along its diameter with constant angular velocity ω.A read of mass m remains at rest w.r.t. ring at the position shown in figure. Then w2 is:
Ag√3B2g√3rC√3grDgr - Question 2 - Select One
A thin circular ring of mass per unit length p and radius r is rotating at an angular speed ω as shown in figure. The tension in the ring is
Apω2r2/2Bpω2r2Cpω2r2/3DNone - Question 3 - Select One
A thin circular ring of mass M and radius r is rotating about its axis with a constant angular velocity ω. Two objects each of mass m are attached gently to the opposite ends of a diameter of the ring. The ring will now rotate with an angular velocity of
Aω(M−2m)(M+2m)BωM(M+2m)CωM(M+m)Dω(M+2m)M
A ring of mass m and radius R is being rotated about its axis with constant angular velocity ω in the gravity free space. Find tension in the ring.
View SolutionA thin circular ring of mass M and radius R is rotating about its axis with an angular speed ω0 two particles each of mass m are now attached at diametrically opposite points. Find new angular speed of the ring.
View SolutionA ring of mass m and radius R is being rotated about its axis with angular velocity ω. If a increases then tension in ring
View SolutionA thin circular ring of mass M and radius R is rotating about its axis with a constant angular velocity omega. Four objects each of mass m, are kept gently to the opposite ends of two perpendicular diameters of the ring. The angular velocity of the ring will be
View SolutionA thin circular ring of mass M and radius R is rotating about its axis with a constant angular velocity omega. Four objects each of mass m, are kept gently to the opposite ends of two perpendicular diameters of the ring. The angular velocity of the ring will be
View Solution