A conducting rod of mass M and length L can oscillate like a pendulum in a vertical plane about point O. The lower end of the rod glides smoothly on a circular conducting arc of radius L. The circular arc is connected to point O of the rod through a capacitor of capacitance C. The entire device is kept in a uniform horizontal magnetic field B directed into the plane of the Figure. Disregard resistance of any component. The rod is deflected through a small angle θ0 from vertical position and released at time t = 0.
(a) Write the deflection angle (θ) of the rod as a function of time t.
(b) If the capacitor is replaced with a resistor what kind of motion do you expect? Give qualitative description only.
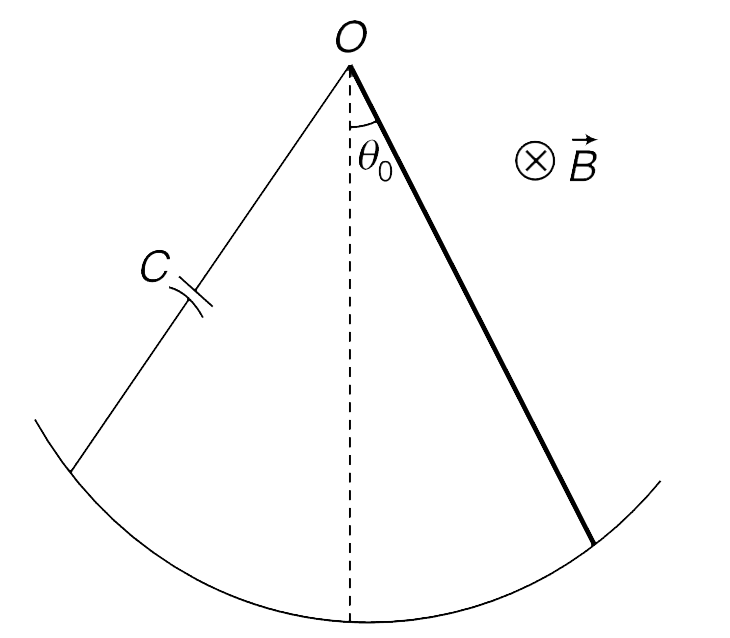
The correct Answer is:(a) θ=θ0cosωt where ω=[6Mg4ML+3B2L3C]1/2
(b) Oscillations die after some time.
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - ARIHANTCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Level 3
12 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - ARIHANTCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Level 3
12 Videos
- CURRENT ELECTRICITYBOOK - ARIHANTCHAPTER - CURRENT ELECTRICITYEXERCISE - Current Electricity
105 Videos
- ELECTROSTATICSBOOK - ARIHANTCHAPTER - ELECTROSTATICSEXERCISE - Level 3
59 Videos
Similar Questions
A uniform rod of mass m and length L is free to rotate in the vertical plane about a horizontal axis passing through its end. The rod initially in horizontal position is released. The initial angular acceleration of the rod is:

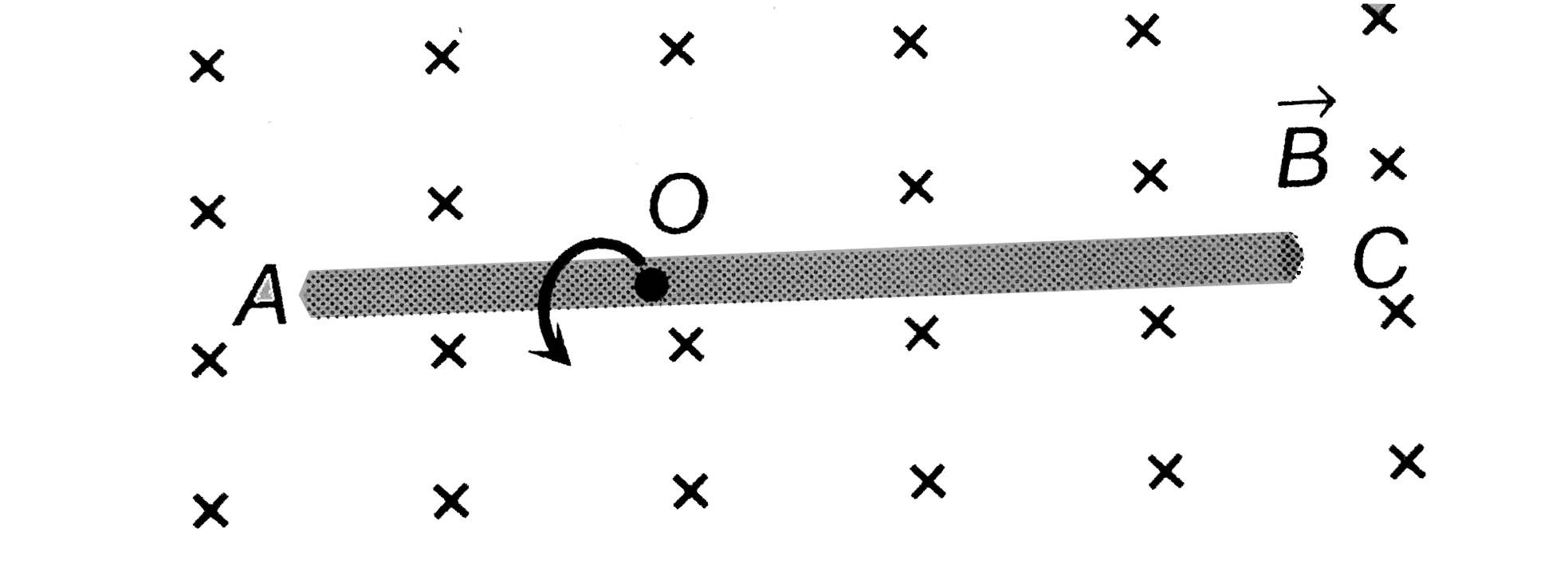
View SolutionA conducting rod AC of length 4l is rotate about a point O in a uniform magnetic field →B directed into the paper. AO=l and OC=3l. Then
View Solution
Knowledge Check
A conducting rod AC of length 4l is rotated about point O in a uniform magnetic field →B directed into the plane of the paper. AO=landOC=3l. Find VA−VC.
 AVA−Vo=Bωl22BVo−VC=72Bωl2CVA−Vc=4Bωl2DVc−Vo=92Bωl2
AVA−Vo=Bωl22BVo−VC=72Bωl2CVA−Vc=4Bωl2DVc−Vo=92Bωl2A conducting rod AB moves parallel to x-axis in a uniform magnetic field pointing perpendicular and into the plane of paper. The end A of the rod
 Agets positively chargedBgets negatively chargedCremains neutralDgets positively charged first and then negatively charged
Agets positively chargedBgets negatively chargedCremains neutralDgets positively charged first and then negatively chargedA conducting rod of length l is hinged at point O. It is a free to rotate in a verical plane. There exists a uniform magnetic field B in horizontal direction. The rod is released from the position shown. The potential difference between the two ends of the rod is proportional to
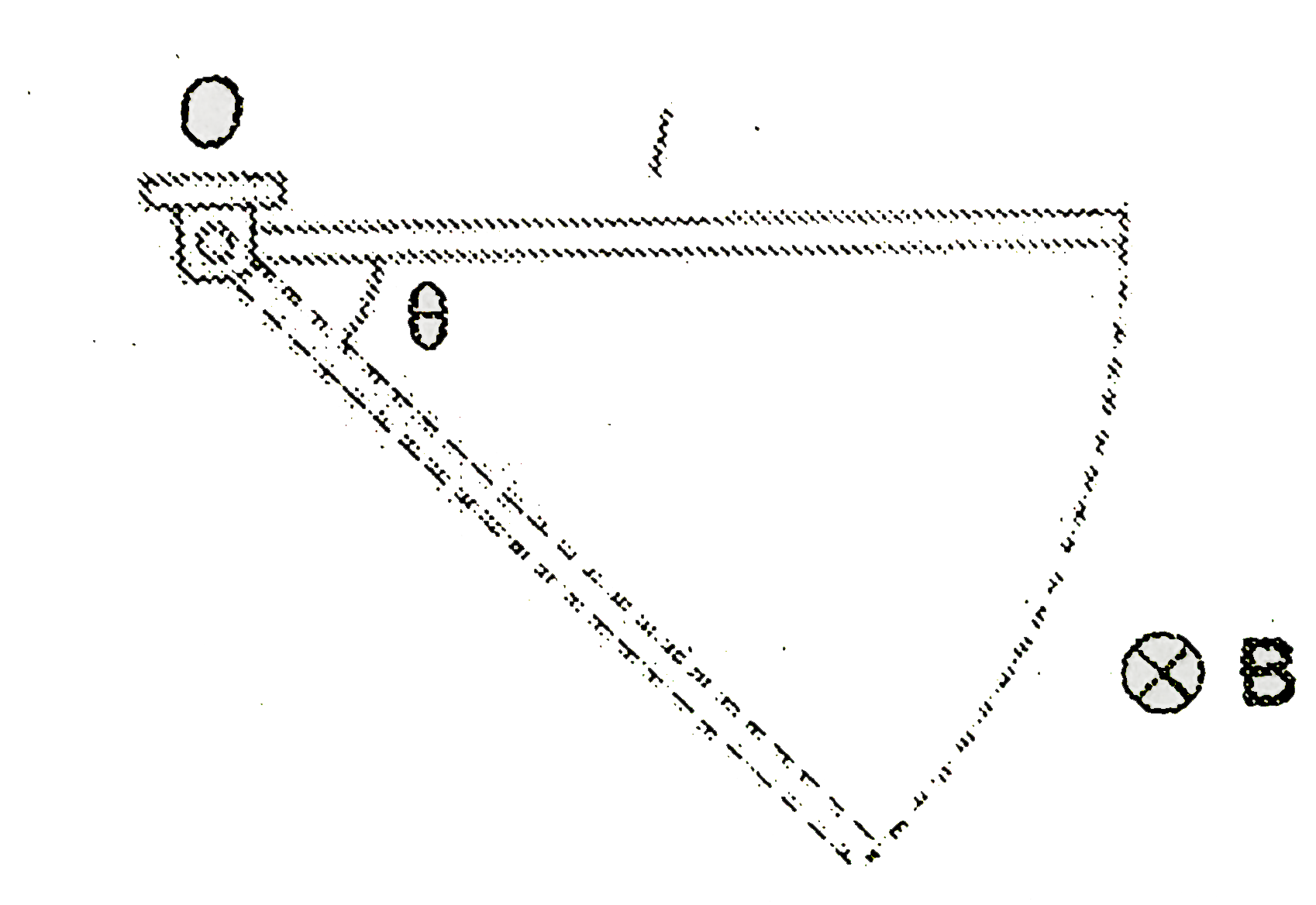 Al3/2Bl2Csinθ/2Dsinθ
Al3/2Bl2Csinθ/2Dsinθ
A conducting rod AC of length 4l is rotated about point O in a uniform magnetic field →B directed into the plane of the paper. AO=landOC=3l. Find VA−VC.

View SolutionA conducting rod AC of length 4l is rotated about point O in a uniform magnetic field →B directed into the plane of the paper. AO=landOC=3l. Find VA−VC.

View SolutionA conducting rod AB of length l is rotated about point O with an angular velocity velocity omega in a uniform magnetic field (B_0) directed perpendicular into the plane of rotation as shown in the figure. The value of VB−VA is
View SolutionIn the figure shown a uniform conducing rod of mass m and length l is suspended invertical plane by two conducing springs of spring constant k each. Upper end of springs are connected to each other by a capacitor of capacitance C. A uniform horizontal magnetic field (B0) perpendicular to plane of springs in space initially rod is in equillibrium. If the rod is pulled down and released, it performs SHM. (Assume resistance of springs and rod are negligible)
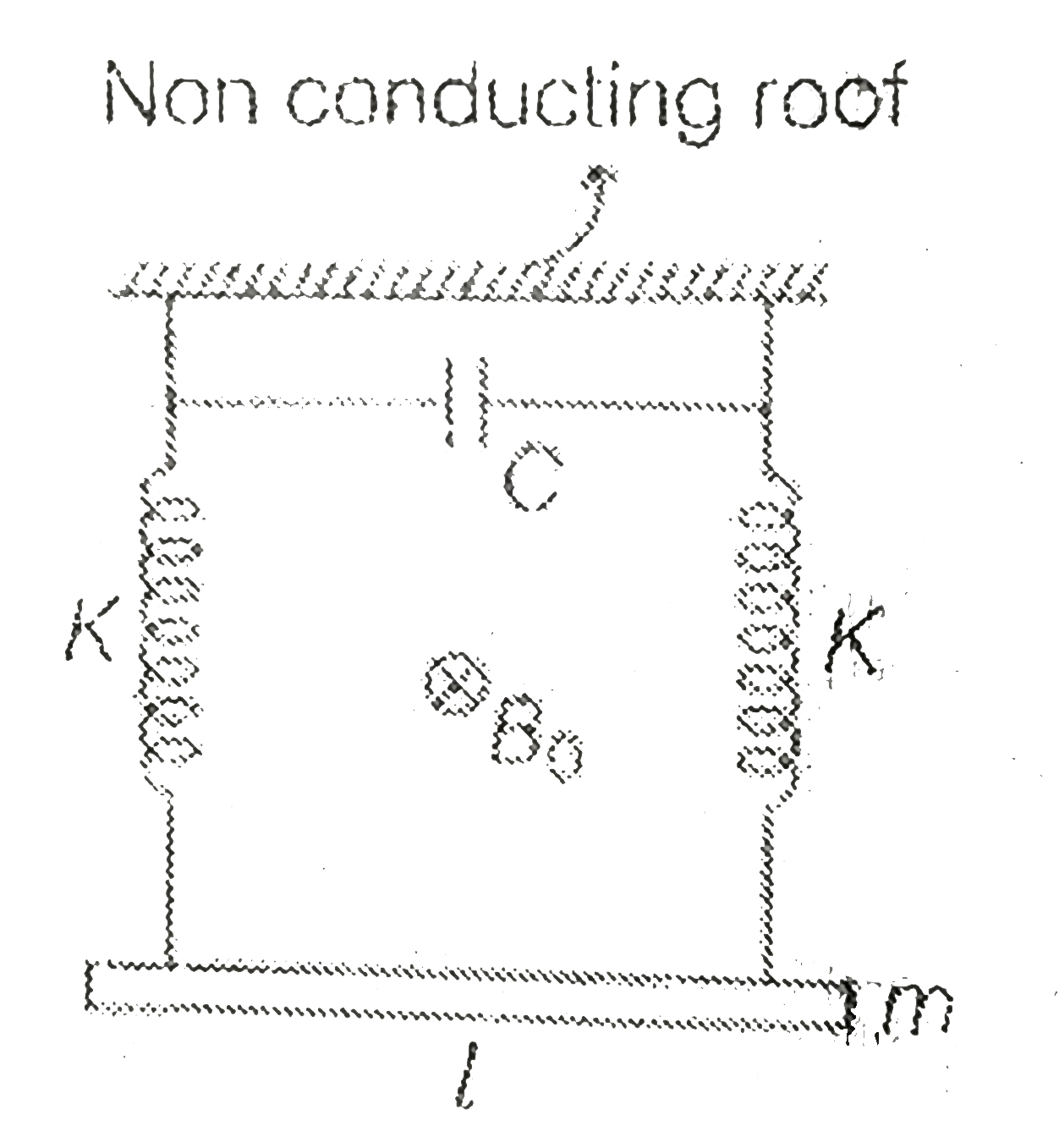
Find the period of oscillation of rod.
View SolutionIn the figure shown a uniform conducing rod of mass m and length l is suspended invertical plane by two conducing springs of spring constant k each. Upper end of springs are connected to each other by a capacitor of capacitance C. A uniform horizontal magnetic field (B0) perpendicular to plane of springs in space initially rod is in equillibrium. If the rod is pulled down and released, it performs SHM. (Assume resistance of springs and rod are negligible)

Find the period of oscillation of rod.
View Solution
