There are two co-axial non conducting cylinders of radii a and b(>a). Length of each cylinder is L(>>b) and their curved surfaces have uniform surface charge densities of–σ (on cylinder of radius a) and +σ (on cylinder of radius b). The two cylinders are made to rotate with same angular velocity ω as shown in the figure. The charge distribution does not change due to rotation. Find the electric field (E) and magnetic field (B) at a point (P) which is at a distance r from the axis such that (a) 0<r<a (b) a<r<b (c) r>b. Assume that point P is close to perpendicular bisector of the length of the cylinders

The correct Answer is:E=0forr<a
=aσ∈0r radially inward for a<r<b
=((b−a))σ)∈0r radially outward for r<b
B=σω(b−a) up along the axis for r<a
=bσω up along the axis for a<r<b=0forr<b
Topper's Solved these Questions
Similar Questions
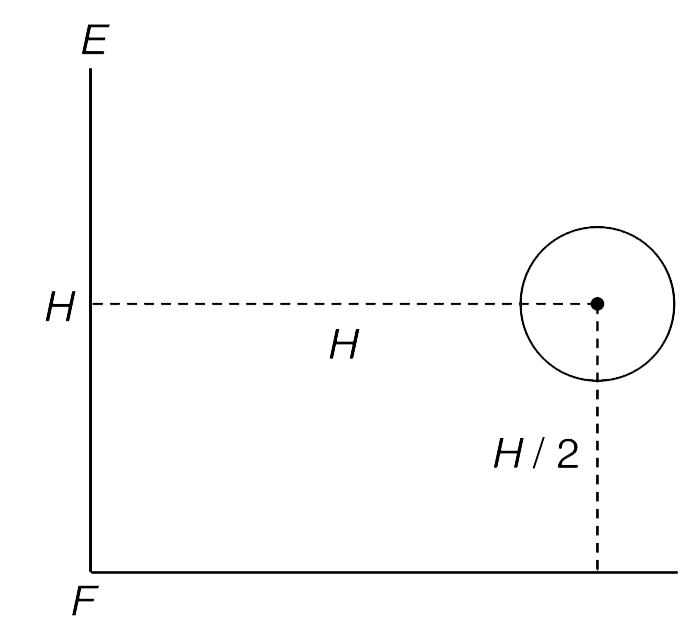
In the Figure FE is a man of height H standing on a floor. E is eye of the man and F is his foot. The distance between eye and the head is negligible. A steel ball of radius r is suspended in front of him. The distance of the ball from the man is H and height of the centre of the ball from the floor is H2. It is given that r<<H. The surface of the ball acts like a mirror and the man sees his image in it. Calculate the angle subtended by the image at the eye of the man.
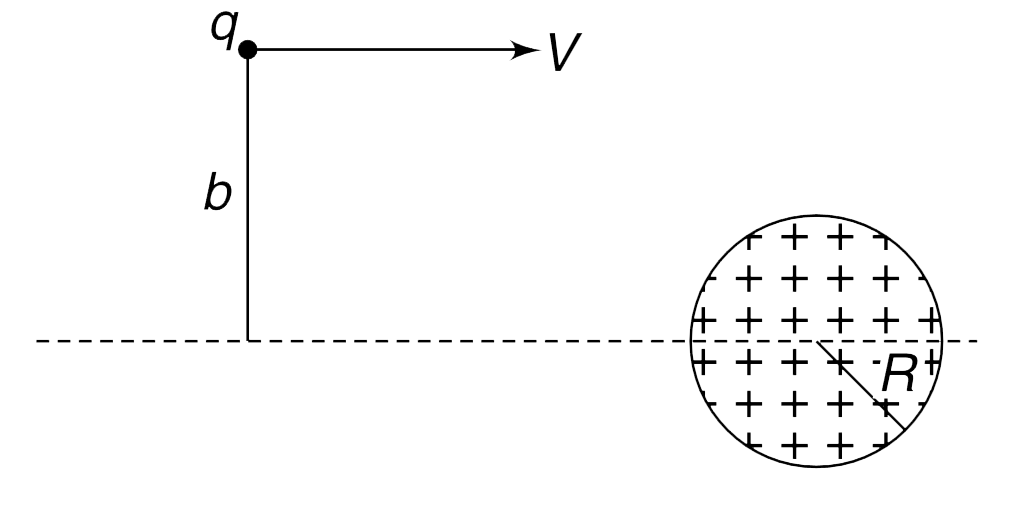
View SolutionThere is a fixed sphere of radius R having positive charge Q uniformly spread in its volume. A small particle having mass m and negative charge (–q) moves with speed V when it is far away from the sphere. The impact parameter (i.e., distance between the centre of the sphere and line of initial velocity of the particle) is b. As the particle passes by the sphere, its path gets deflected due to electrostatics interaction with the sphere.
(a) Assuming that the charge on the particle does not cause any effect on distribution of charge on the sphere, calculate the minimum impact parameter b0 that allows the particle to miss the sphere. Write the value of b0 in term of R for the case
12mV2=100.(kQqR)
(b) Now assume that the positively charged sphere moves with speed V through a space which is filled with small particles of mass m and charge – q. The small particles are at rest and their number density is n [i.e., number of particles per unit volume of space is n].
The particles hit the sphere and stick to it. Calculate the rate at which the sphere starts losing its positive charge dQdt. Express your answer in terms of b0
View SolutionA cylinder of radius R and length l is placed in a uniform electric field E parallel to the axis of the cylinder. The total flux over the curved surface of the cylinder is
View Solutiona point charge q is placed at the centre of a cylinder of length L and radius R the electric flux through the curved surface of the cylinder is
View SolutionA long charged cylinder of linear charged density λ is surrounded by a hollow co-axial conducting cylinder. What is the electric field in the space between the two cylinders ?
View SolutionA charge q is placed at the centre of a cylinder of radius R and length 2R. Then electric flux through the curved surface of the cylinder is
View SolutionThe space between two coaxial cylinders, whose radii are a and b (where a ltb as in (figure shown) is filled with a conducting medium . The specific conductivity of the medium is sigma . (a) Compute the resistance along the lenght of cylinder . (b) Compute the resistance between the cylinders are very long as compared to their radii , i.e ., Lgtgt b, where L is the lenght of the cylinders.
View SolutionA long, hollow insulating cylinder of radius R and negligible thickness has a uniform surface charge density sigma . The cylinder rotates about its central axis at a constant angular speed omega . Choose the correct option(s):
View SolutionA long charged cylinder of linear charge density lambda surrounded by a hollow co-axial conducting cylinder. What is the electric field in the space between the two cylinders?
View SolutionA long charged cylinder of linear charge density λ is surrounded by a hollow co-axial conducting cylinder. What is electric field in the space between the two cylinders?
View SolutionA long charged cylinder of linear charged density lambda is surrounded by a hollow co-axial conducting cylinder. What is the electric field in the space between the two cylinders?
View SolutionA long charged cylinder of linear charged density lambda is surrounded by a hollow co-axial conducting cylinder. What is the electric field in the space between the two cylinders?
View SolutionA long charged cylinder of linear charged density lambda is surrounded by a hollow co-axial conducting cylinder. What is the electric field in the space between the two cylinders?
View SolutionA long charged cylinder of linear charge density λ is surrounded by a hollow co-axial conducting cylinder. What is the electric field in the space between the two cylinders ?
View SolutionA long charged cylinder of linear charged density à is surrounded by a hollow co-axial conducting cylinder. What is the electric field in the space between the two cylinders ?
View Solution
