In a two dimensional x–y plane, the magnetic field lines are circular, centred at the origin. The magnitude of the field is inversely proportional to distance from the origin and field at any point P has magnitude given by B=kr , where k is a positive constant. A wire carrying current I is laid in xy plane with its ends at point A (x1,y1 and point B (x2),y2). Find force on the wire.

The correct Answer is:→F=kl2ln(x22+y22x21+y21)ˆk
Topper's Solved these Questions
Similar Questions
Knowledge Check
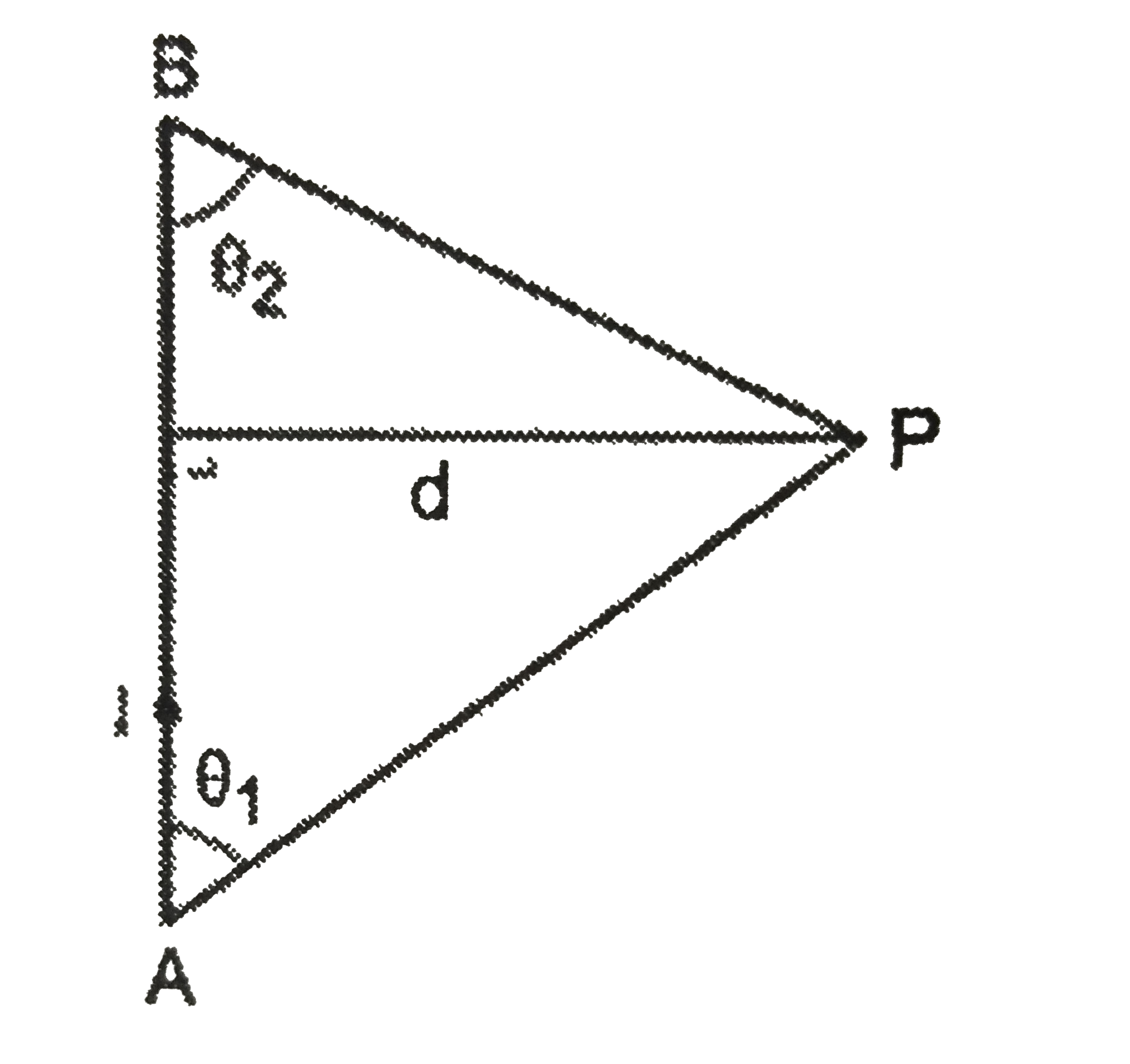
AbB is a section of a straight wire carrying a current I. P is a point at a distance d from AB. The magnetic field at P due to AB has magnitudeAμ0I4πd(cosθ1+cosθ2)Bμ0I4πd(cosθ1−cosθ2)Cμ0I4πd(sinθ1+sinθ2)Dμ0I4πd(sinθ1−sinθ2)A semicircule wire of radius R and carrying a current I si placed in the XY plane where the uniform magnetic field is B=B0K. The force on the wire is
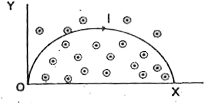 AπIRBˆjB−πIRBˆjC2IRBˆjD−2IRBˆj
AπIRBˆjB−πIRBˆjC2IRBˆjD−2IRBˆj
The magnitude of magnetic field at a distance 'r' from a long straight wire carrying a current I is given by B = …………………… .
View SolutionA conducting wire carrying current I is shown in the figure. The magnitude of magnetic field at the point P will be

View SolutionA conducting wire carrying current I is shown in the figure. The magnitude of magnetic field at the point P will be

View SolutionA sphere of radius R carries charge density p proportional to the square of the distance from the centre such that p=Cr2, where C is a positive constant. At a distance R/2 from the centre, the magnitude of the electric field is :
View SolutionMagnitude of the magnetic field produced at a given point is directly proportional to the magnitude of the ___________.
View SolutionThere are two parallel current carrying wires X and Y as shown in figure. Find the magnitude and direction of the magnetic field at points, P, Q and R.
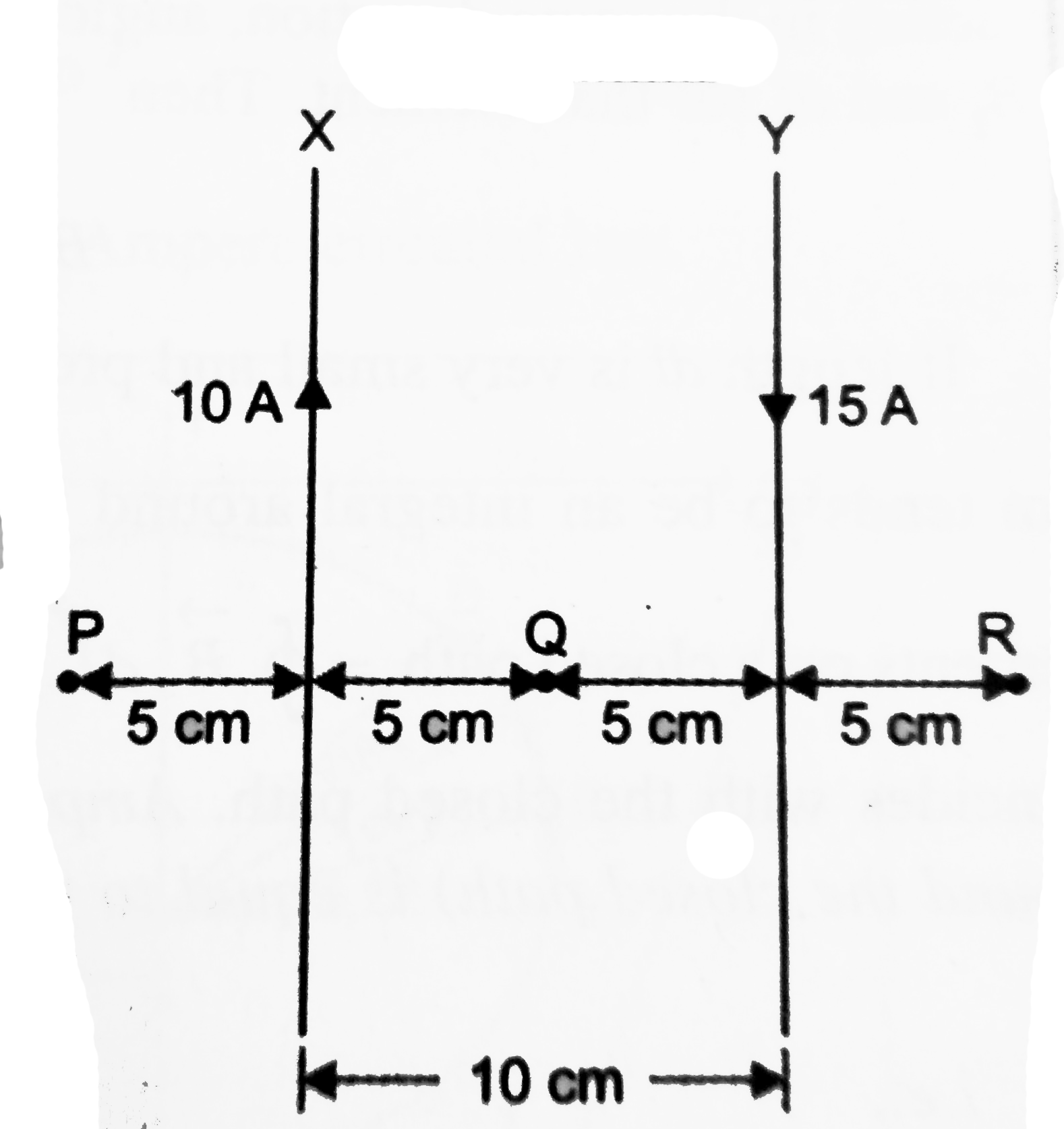
View Solution
