A uniform ring of mass M and radius R carries a current I (see figure). The ring is suspended using two identical strings OA and OB. There exists a uniform horizontal magnetic field B0 parallel to the diameter AB of the ring. Calculate tension in the two strings. [Given θ=60∘]
![A uniform ring of mass M and radius R carries a current I (see figure). The ring is suspended using two identical strings OA and OB. There exists a uniform horizontal magnetic field B(0) parallel to the diameter AB of the ring. Calculate tension in the two strings. [Given theta = 60^(@)]](https://d10lpgp6xz60nq.cloudfront.net/physics_images/IJA_PHY_V02_C10_E01_041_Q01.png)
The correct Answer is:TAO=Mg+πIRB0
TBO=Mg−πIRB0
Topper's Solved these Questions
Similar Questions
A uniform conducting rectangular loop of sides l, b and mass m carrying current i is hanging horizontally with the help of two vertical strings. There exists a uniform horizontal magnetic field B which is parallel to the longer side of loop. The value of tension which is least is
View SolutionA conducting ring of mass 2kg, radius 0.5m carries a current of 4A. It is placed on a smooth horizontal surface. When a horizontal magnetic field of 10 T parallel to the diameter of the ring is applied, the initial acceleration is (in rad/sec2)
View Solution
Knowledge Check
A uniform conducting rectangular loop of sides l, b and mass m carrying current i is hanging horizontally with the help of two vertical strings. There exists a uniform horizontal magnetic field B which is parallel to the longer side of loop. The value of tension which is least is
Amg−Bb2Bmg+Bb2Cmg−2iBb2Dmg+2Bb2A conducting ring of radius 2R rolls on a smooth horizontal conducting surface as shown in figure-5.295. A uniform horizontal magnetic field B is perpendicular to the plane of the ring. The potential of A with respect to O is:
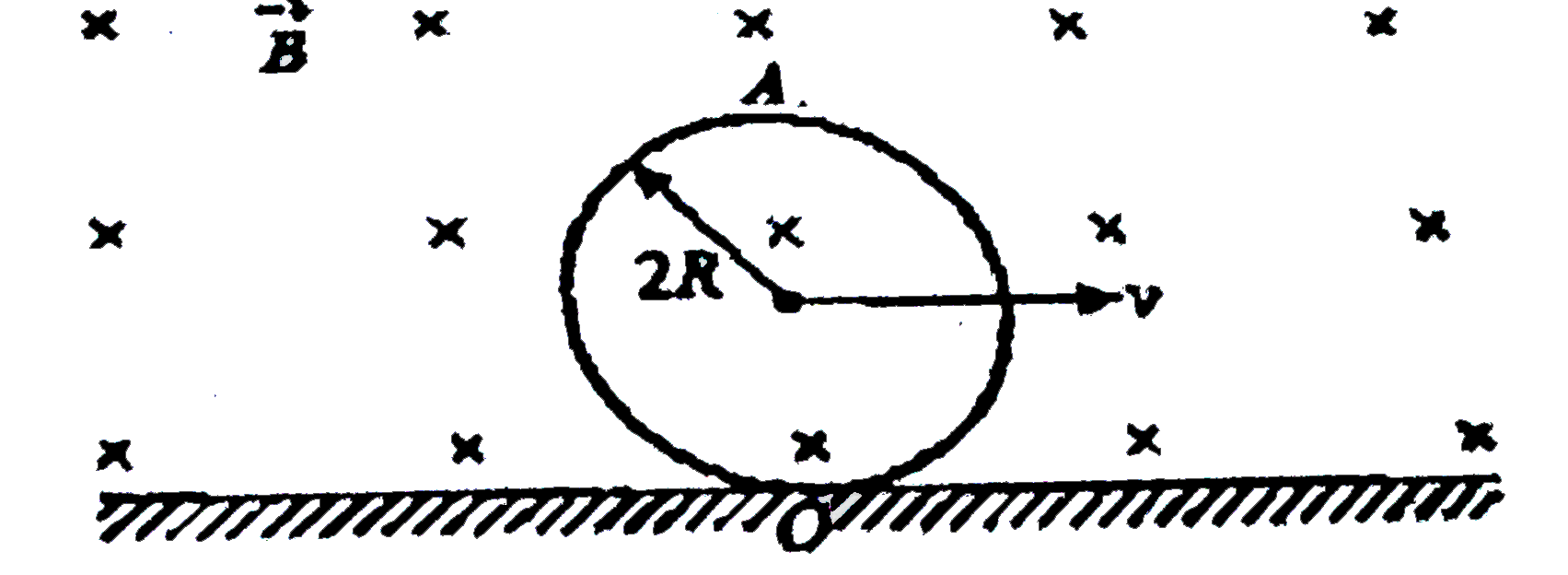A2 BvRB12BvRC8BvRD4BvRA conducting ring of mass 2kg and radius 0.5m is placed on a smooth plane. The ring carries a current of i=4A. A horizontal magnetic field B=10T is switched on at time t=0 as shown in fig The initial angular acceleration of the ring will be
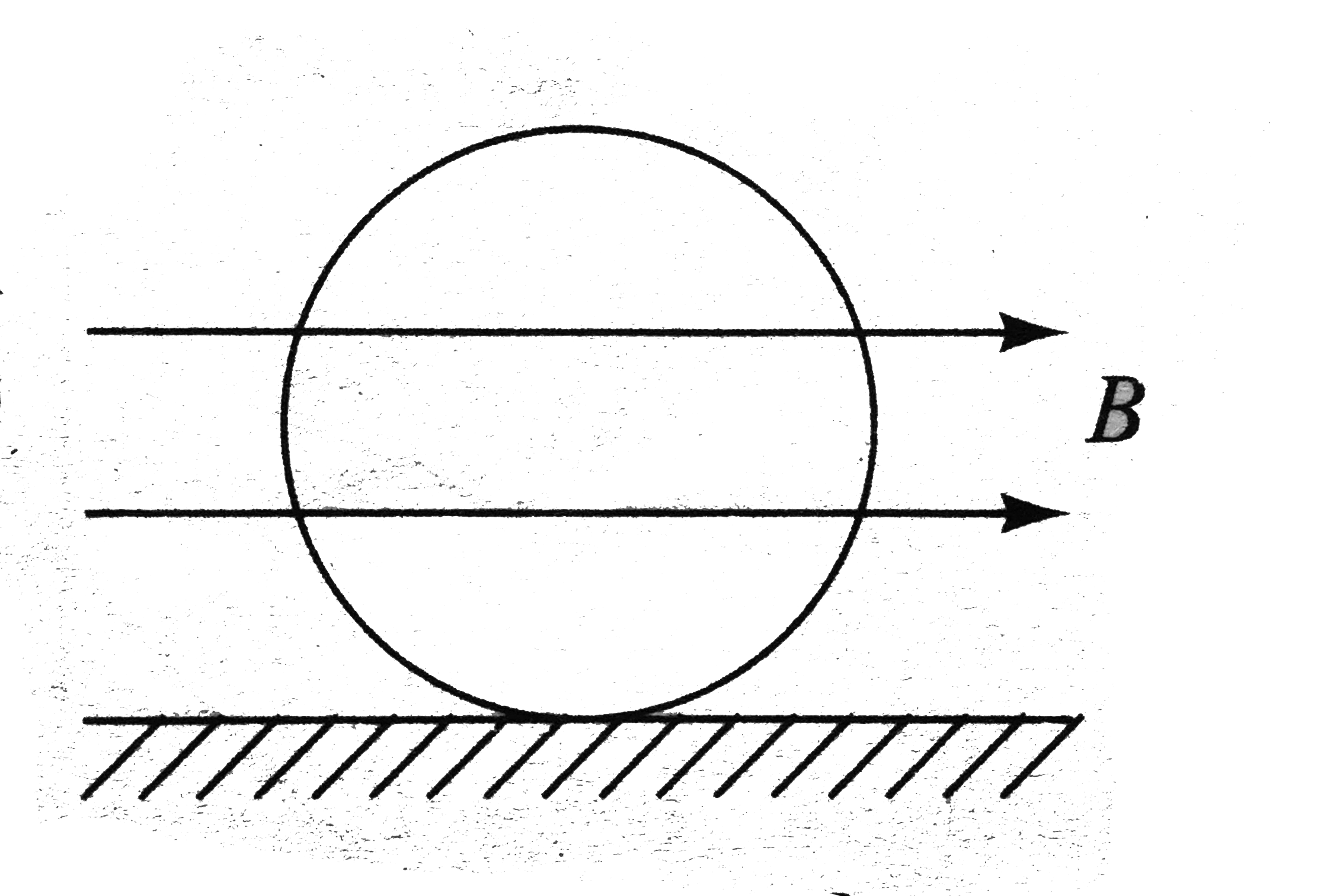.A40πrads−2B20πrads−2C5πrads−2D15πrads−2
A uniformly conducting wire is bent to form a ring of mass 'm' and radius 'r', and the ring is placed on a rough horizontal surface with its plane horizontal. There exists a uniform and constant horizontal magnetic field of induction B. Now a charge q is passed through the ring in a very small time interval Δt. As a result the ring ultimately just becomes vertical. Calculate the value of g(acceleration due to gravity) in terms of other given quantities. Assume that friction is sufficient to prevent slipping and ignore any loss in energy.
View SolutionA uniformly conducting wire is bent to form a ring of mass 'm' and radius 'r', and the ring is placed on a rough horizontal surface with its plane horizontal. There exists a uniform and constant horizontal magnetic field of induction B. Now a charge q is passed through the ring in a very small time interval Δt. As a result the ring ultimately just becomes vertical. Calculate the value of g(acceleration due to gravity) in terms of other given quantities. Assume that friction is sufficient to prevent slipping and ignore any loss in energy.
View SolutionA conducting ring of mass 2 kg and radius 0.5 m is placed on a smooth horizontal plane. The ring carries a current 4A . A horizontal magnetic field B = 10T is switched on at time t = 0 as shown in figure. The initial angular acceleration of the ring will be:
View SolutionA conducting ring of mass 2kg and radius 0.5m is placed on a smooth plane. The ring carries a current of i=4A. A horizontal magnetic field B=10T is switched on at time t=0 as shown in fig The initial angular acceleration of the ring will be
.
View SolutionA conducting ring of mass 2kg and radius 0.5m is placed on a smooth plane. The ring carries a current of i=4A. A horizontal magnetic field B=10T is switched on at time t=0 as shown in fig The initial angular acceleration of the ring will be
.
View Solution