Two solid sphres of density each and R and 2R are hung from a spring and a thread as shown if the thread is burnt.
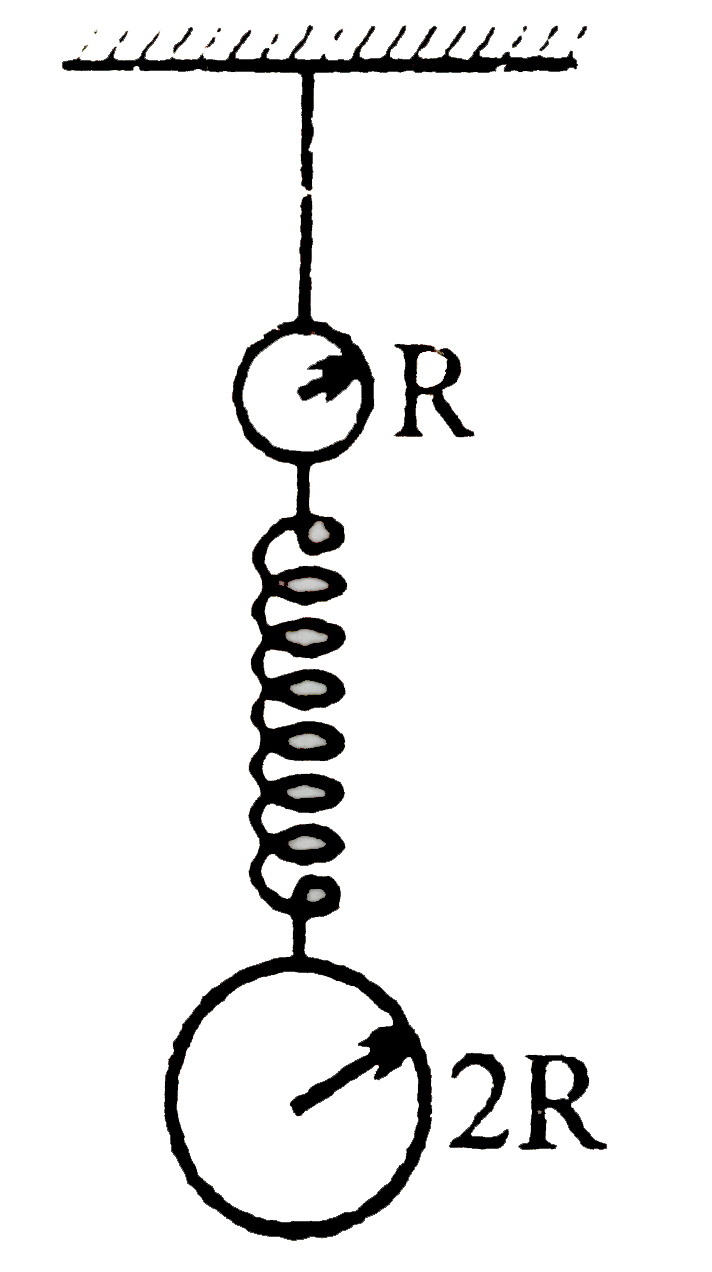
The initial acceleration of lower sphere is zero
The initial acceleration of upper sphere is
The initial acceleration of upper sphere is
The initial acceleration of lower sphere is
The correct Answer is:AB
Mass of upper spheres
Mass of lower sphere =
at equation kx=8 mg
when string is brunt force in spring will into change.
as force in spring doesn't change instantaneously.
Topper's Solved these Questions
- TEST PAPERSBOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - PHYSICS PART (A)
32 Videos
- TEST PAPERSBOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - PHYSICS PART (B)
3 Videos
- TEST PAPERSBOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - PHYSICS PART- C
4 Videos
- SEMICONDUCTORSBOOK - BANSALCHAPTER - SEMICONDUCTORSEXERCISE - CBSE Question
32 Videos
Similar Questions
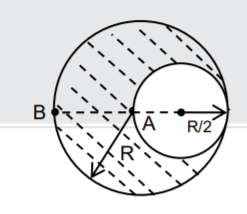
A solid sphere having radius R and uniform charge density ρ has a cavity of radius R2 as shown in figure then the value of ∣∣∣(EAEB)∣∣∣=m17 .Find the value of m
View SolutionTwo solid spheres A and B each of radius R are made of materials of densities ρA and ρB respectively. Their moments of inertia about a diameter are IA and IB respectively. The value of IAIB is
View Solution
Knowledge Check
The M.I. of the solid sphere of density 'rho' and radius 'R' about an axis passing through its centre is given by
A105176R2ρB176105R2ρC176105R5ρD105132R2ρA solid sphere of radius R and density ρ is attached to one end of a mass-less spring of force constant k. The other end of the spring is connected to another solid sphere of radius R and density 3ρ. The complete arrangement is placed in a liquid of density 2ρ and is allowed to reach equilibrium. The correct statements(s) is (are)
A(a) The net elongation of the spring is 4πR3ρg3kB(b) The net elongation of the spring is 8πRρg3kC(c) The light sphere is partially submergedD(d) The light sphere is completely submergedTwo solid hemispheres of radii R and R2 with centers O and O' respectively as shown in figure. The density of bigger hemisphere is ρ and that of smaller hemisphere is 2ρ. Taking center of bigger hemisphere is at origin and the distance between centres of two hemisphere OO' is R10 find co - ordinates of center of mass of the system.
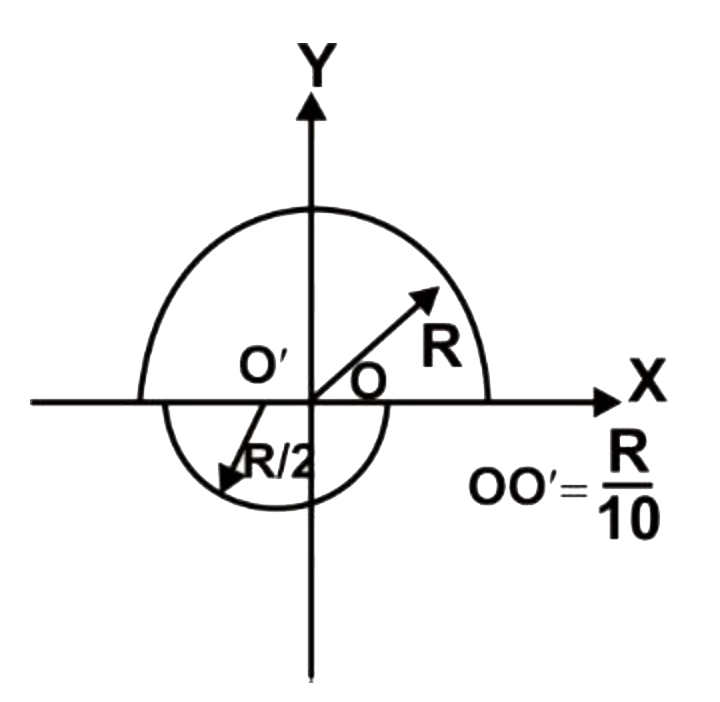 A(−R50,21R80)B(−R30,21R80)C(−R50,7R16)D(−R30,7R16)
A(−R50,21R80)B(−R30,21R80)C(−R50,7R16)D(−R30,7R16)
From a solid cylined of length 4R and radius R, two hemispheres of radius R and R2 are cut from both the ends as shown. The location of new centre of mass from point O is approximately

View SolutionA solid spher of mass m1 density ρ1 radius R1 and a solid cone of mass m2 density ρ2. Radius R2, are arranged as shown in the figure. The centre of mass is located at
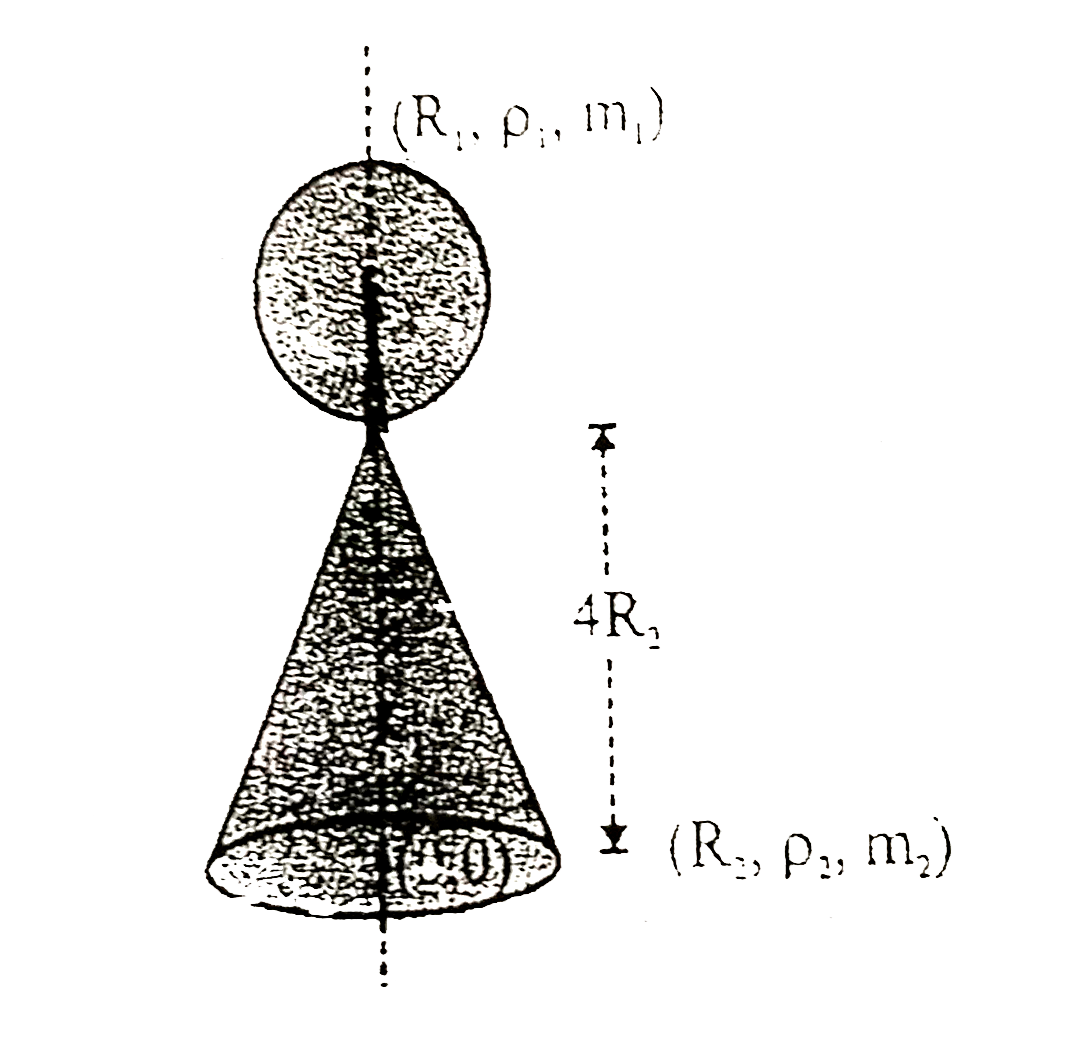
View SolutionTwo non-conducting solid spheres of radii R and 2R, having uniform volume charge densities ρ1 and ρ2 respectively, touch each other. The net electric field at a distance 2R from the centre of the smaller sphere, along the line joining the centres of the spheres, is zero. The ratio ρ1ρ2 can be
View SolutionA solid sphere having radius R and uniform charge density ρ has a cavity of radius R2 as shown in figure.Find the value of ∣∣∣EAEB∣∣∣
View SolutionIf a small sphere of mass m and charge q is hung from a silk thread at an angle θ with the surface of a vertical charged conducting plate, then for equilibrium of sphere, the surface charge density of the plate is
View Solution
