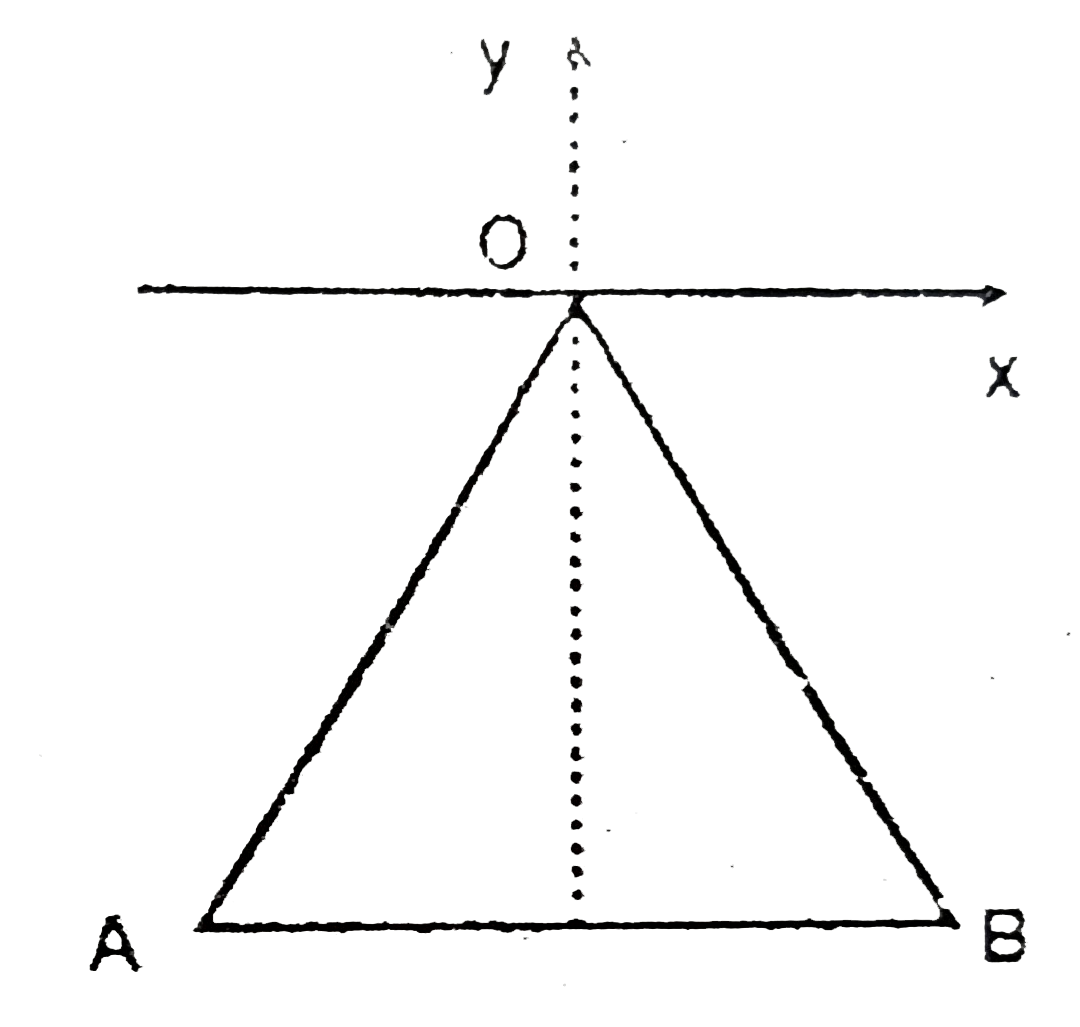
A triangular of rigid wire frame $' A O B'$ is made, in which length of each wire is $l$ and mass $m$ . The whole system is suspended from point $O$ and free to perform $S H M$ about $x$ -axis or about $z -$ axis. When it performs $S H M$ about $x -$ axis its time period of oscillation is $T_{1}$ and when it performs $S H M$ about $z -$ axis, its time-period of osciallation is $T_{2}$ , then choose the correct option.

$T_{1} < T_{2}$

$T_{1} > T_{2}$

$T_{1} = T_{2}$

None

Text Solution

Verified by Experts

$T = 2 π \sqrt{\frac{1}{M_{s y s} g d}}$ , $M_{s y s} = 3 m$ , $d = \frac{l \sqrt{3}}{2} \times \frac{2}{3} = \frac{l}{\sqrt{3}}$
Case I : System is performing SHM about $x -$ axis
$I_{X} = I_{A B} + I_{A O} + I_{B O} = \frac{3 m l^{2}}{4} + [\frac{m l^{2}}{3} {sin}^{2} 60^{\circ}] \times 2 = \frac{5 m l^{2}}{4}$
$T_{1} = 2 π \sqrt{\frac{I_{x}}{M_{s y s} g d}} = 2 π \sqrt{\frac{\frac{5 m l^{2}}{4} \times \sqrt{3}}{3 m \times g \times l}} = 2 π \sqrt{\frac{5 \sqrt{3} l}{12 g}}$
Case II : System is performing SHM about $z -$ axis
$I_{z} = I_{A B} + I_{A O} + I_{B O} = ⎡ ⎣ \frac{m l^{2}}{12} + m {(\frac{\sqrt{3} l}{2})}^{2} ⎤ ⎦ + \frac{m l^{2}}{3} + \frac{m l^{2}}{3} = m l^{2} [\frac{1}{12} + \frac{3}{4} + \frac{2}{3}]$
$= m l^{2} [\frac{1 + 9 + 8}{12}] = \frac{3 m l^{2}}{2}$
$T_{2} = 2 π \sqrt{\frac{I_{z}}{M_{s y s} g d}} = 2 π \sqrt{\frac{3 m l^{2} \times \sqrt{3}}{2 \times 3 m \times g \times l}} = 2 π \sqrt{\frac{\sqrt{3} l}{2 g}}$

Updated on:21/07/2023

A uniform rod of mass m and length l is suspended about its end. Time period of small angular oscillations is

A $2 π \sqrt{\frac{l}{g}}$
B $2 π \sqrt{\frac{2 l}{g}}$
C $2 π \sqrt{\frac{2 l}{3 g}}$
D $2 π \sqrt{\frac{l}{3 g}}$
A particle is performing SHM about mean position O as shown in the figure given below. Its amplitude is 5 m and time period of oscillation is 4 s. The minimum time taken by the particle to move from point A to B is nearly
A0.18 s
B0.20s
C0.15s
D0.24s
A particle performing SHM is found at its equilibrium position at t = 1s and it is found to have a speed 0.25 = m//s at t=2s. If the period of oscillation is 6. Calculate amplitude of oscillation.
A $\frac{3}{2 π} m$
B $\frac{3}{4 π} m$
C $\frac{6}{π}$ m
D $\frac{3}{8 π}$ m