Four equal charges of value +Q are placed at any four vertices of a regular hexagon of side a. By suitably choosing the vertices, what can be the maximum possible magnitude of electric field at the centre of the hexagon?
(A) Q4π∈0a2
(B) √2Q4π∈0a2
(C) √3Q4π∈0a2
(D) 2Q4π∈0a2Solution in Bengali
Q4π∈0a2
√2Q4π∈0a2
√3Q4π∈0a2
2Q4π∈0a2
If the four +Q charges are placed at four adjacent vertices, then the net electric field at point O will be maximum and the electric field (E1) due to each charge will be equal in magnitude.
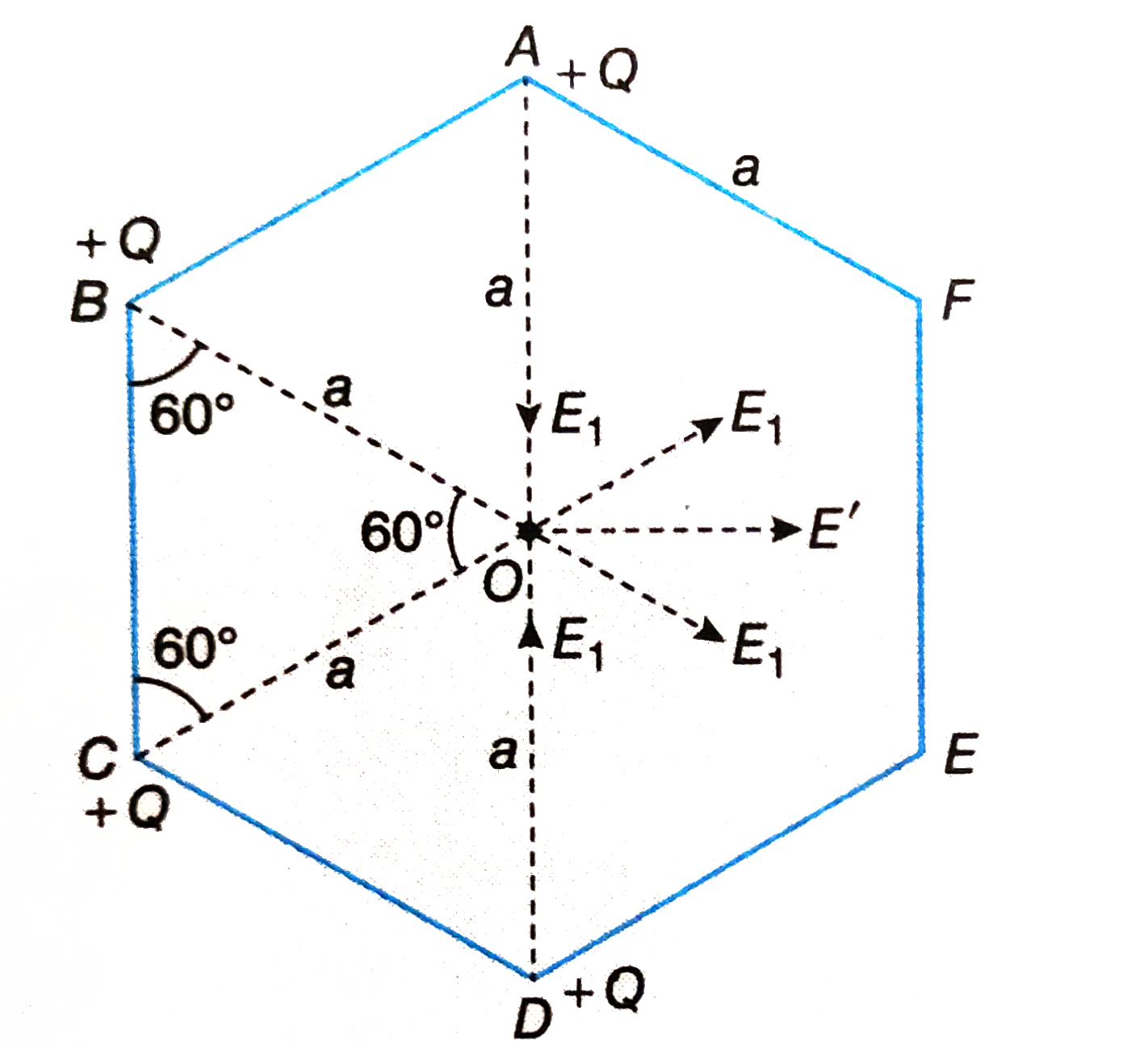
Since, ABCDEF, is a regular hexagon,
So, AO=BO=CO=DO=a
The net electric field at point O due to +Q charges placed at points A and D is zero. Hence the net electric field at point O due to +Q charges placed at points B and C is the maximum values of the electric field.
Now, E1=Q4π∈0a2
∴E'=√E21+E21+2E1E1cos60∘=√3Q4π∈0a2
Topper's Solved these Questions
- BOOK - CHHAYA PUBLICATIONCHAPTER - ELECTRIC FIELDEXERCISE - EXAMINATION ARCHIVE ( JEE MAIN )3videos
ELECTRIC FIELD
- BOOK - CHHAYA PUBLICATIONCHAPTER - ELECTRIC FIELDEXERCISE - EXAMINATION ARCHIVE ( AIPMT)1videos
ELECTRIC FIELD
- BOOK - CHHAYA PUBLICATIONCHAPTER - ELECTRIC FIELDEXERCISE - EXAMINATION ARCHIVE (WBCHSE)13videos
ELECTRIC FIELD
- BOOK - CHHAYA PUBLICATIONCHAPTER - ELECTRIC ENERGY AND POWEREXERCISE - CBSE SCANNER7videos
ELECTRIC ENERGY AND POWER
- BOOK - CHHAYA PUBLICATIONCHAPTER - ELECTRIC POTENTIAL EXERCISE - CBSE Scanner13videos
ELECTRIC POTENTIAL
Similar Questions
A long cylindrical shell carries positive surface charge σ in the upper half and negative surface charge −σ in the lower half. The electric field lines around the cylinder will look like figure given in (figures are schematic and not drawn to scale)
02:27View SolutionThe eletric field in a certina region is acting radially outward and is given by E = Ar. A charge contained in 'a' centred at the origin of the field will be givne by
02:19View SolutionFour point charges +q,+q,+q and −q are placed at the four comers of a square, length of each side of which is a.
Find the magnitude of the intensity of the electric field at the centre of the square.03:37View SolutionA light bulb is rated 100 W for 220 V ac supply of 50 Hz. Calculate
the resistance of the bulb ,03:42View SolutionA test charge q is moved without acceleration along the path from A to B from B to C in electric field E as shown in fig.

(i) Calculate the potential difference between A and C.02:30View SolutionFive point charge ( +q each) are placed at the five vertices of a regular hexagon of side 2a .what is the magnitude of the net electric field at the centre of the hexazon?
01:51View SolutionFive point charges, each of value +q are placed on five vertices of a regular hexagon of side Lm. What is the magnitude of the force on a point charge of value -q coulomb placed at the centre of the hexagon?
02:04View SolutionFind the magnitude of the electric field at the point P in the configuration shown in figure- 1.219 (a), (b) and (c ) for d>>a take 2qa=P.
[(a)q4π∈0d2,(b)2q2π∈0d3,(c)q4π∈0d2√1+4a2d2]
 04:08View Solution
04:08View SolutionFind the potential energy of a charge q0 placed at the centre of regular hexagon of side a, if charge q is placed at each vertex of regular hexagon ?
03:11View Solutionसिद्ध कीजिए की किसी गोलीय चालक की धारिता उसकी त्रिज्या की 4π∈0 गुनी होती है |
05:26View SolutionThe elctric potential at any point due to a point charge is v=14π∈0qr.In analogy with this ,What will be magnetic potential at any point due to a magnetic charge?
02:32View SolutionThe electric intensity at any point due to point charge is E=14π∈0×qr2.In analogy with this,What will be magnetic iduction at a point due to a magnetic charge?
02:45View SolutionFour identical point charges, each of charge q, are placed on the vertices of a regular hexagon of side a such that the net electric field at centre of hexagon is zero. If one of the charge is now removed, then the electric field at centre of hexagon is k- 4.60
04:24View SolutionFive point charge (+q each) are placed at the five vertices of a regular hexagon of side 2a.what is the magnitude of the net electric field at the centre of the hexazon?
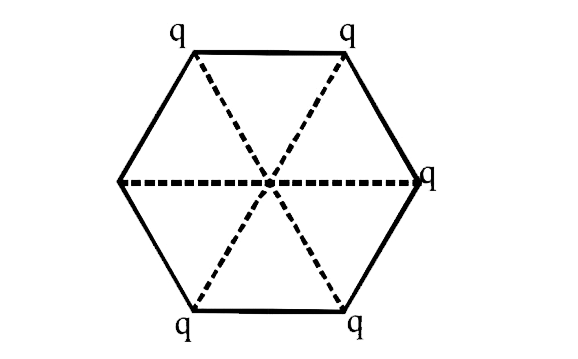
03:33View SolutionFive point charges, each of value +q coulomb, are placed on five vertices of a regular hexagon of side L meters. The magnitude of the force on the point charge of value -q coulomb, placed at the centre of the hexagon is…………….
 03:02View Solution
03:02View Solution

