An isosceles glass prism with base angles 40∘ is champed over a tray of water in a position such that the base is just dipped in water. A ray of light incident normally on the inclined face suffers total internal reflection at the base. If the refractive index of water is 1.33 then the condition imposed on the refractive index μ of the glass is -
μ<2.07
μ>2.07
μ<1.74
μ>1.74
The correct Answer is:B
Step by step video, text & image solution for An isosceles glass prism with base angles 40^(@) is champed over a tray of water in a position such that the base is just dipped in water. A ray of light incident normally on the inclined face suffers total internal reflection at the base. If the refractive index of water is 1.33 then the condition imposed on the refractive index mu of the glass is - by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
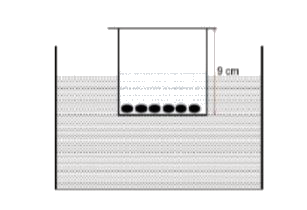
A wide bottom cylindrical massless plastic container of height 9 cm has 40 identical coins inside it and is floating on water with 3 cm inside the water. If we start putting more of such coins on its lid. It is observed that after N coins are put, its equilibrium changes from stable to unstable. Equilibrium in floating is stable if the geometric center of the submerged portion is above the center of mass of the object) The value of N is closed to
View SolutionA ray of light is incident on a glass prism of refractive index mu and refractive angle A. If it just suffers total internal reflection at the other face, obtain an expression relating the angle of incidence , angle of prism and critical angle.
View SolutionDetermine the polarising angle of the light ray moving from water of refractive index 1.33 to glass of refractive index 1.5.
View SolutionA ray of light is incident on a glass. The reflected ray gets totally polarised. The magnitude of incidence angle is (refractive index of glass = μ)
View SolutionA glass prism is immeresed in water as shown in the figure. When a beam of light incident is incident normally on the face ab, it is internally reflected at the face ad. If the refractive index of glass is 1.5 and that of water is 4//3 , then
View SolutionA ray of light incident normally on a refracting surface of the prism is totally reflected from the other refracting surface. If the prism is immersed in water how will the ray act? Refractive index of glass = 1.5, refractive index of water = 1.33.
View SolutionA glass prism of refractive index 1.5 is immersed in water of refractive index 1.33. In this condition what should be the limiting angle of the prism for no emergent ray?
View SolutionAn equilateral glass prism of refractive index 1.5 is immersed in water. Find the angle of deviation produced when a ray of light is incident at 42^(@) on one face of the prism. (Refractive index of water = 1.33)
View SolutionA prism of glass (mu=1.5) is dipped in to water as shown in the figure. If the refractive index of water is 4/3, then the incident ray will be totally reflected if
View SolutionA ray of light travelling in water is incident on a glass plate immersed in it. When the angle of incident is 51∘ the reflected ray is totally plane polarized. Find the refractive index of glass. Refractive index of water is 1.33.
View SolutionRefractive index of glass is 1.6 and that water is 1.33. Angle of polarisation for ray light inciding on glass from water is ......
View SolutionA ray of light is incident on a glass prism of refractive index μ and refractive angle A. If it just suffers total internal reflection at the other face, obtain an expression relating the angle of incidence , angle of prism and critical angle.
View SolutionA prism of glass is dipped in to water as shown in the figure. If the refractive index of water is 4//3 then the incident ray will be totally reflected if
View SolutionA prism of glass ( mu = 1.5) is dipped in to water as shown in the figure. If the refractive index of water is 4/3, then the incident ray will be totally reflected if
View SolutionFind the angle of incidence of a ray of light on one face of a 60°.prism, if the ray is just totally reflected on meeting the opposite face (refractive index of glass = 1.5).
View Solution
