Two identical thin rings each of radius R are coaxially placed at a distance R. If the rings have a uniform mass distribution and each has masses 2m and 4m respectively, then the work done in moving a mass m from centre of one ring to that of the other is
zero
√2Gm2R(1−√2)
Gm2√2R(√2−1)
Gm2√2R
The correct Answer is:B
To find the work done in moving a mass m from the center of one ring to the center of the other, we will calculate the gravitational potential energy at both positions and find the difference.
1. Identify the Configuration:
- We have two identical thin rings, each of radius R.
- The first ring has a mass m1=2m and the second ring has a mass m2=4m.
- The distance between the centers of the two rings is R.
2. Gravitational Potential due to a Ring:
- The gravitational potential U at a distance z from the center of a ring of mass M and radius R is given by:
U=−GM√R2+z2
- Here, G is the gravitational constant.
3. Calculate the Potential at the Center of the First Ring:
- At the center of the first ring (where the mass m starts), the distance z=0:
U1=−G⋅2mR
4. Calculate the Potential at the Center of the Second Ring:
- To find the potential at the center of the second ring, we need to consider the distance from the center of the first ring to the center of the second ring, which is R.
- The distance from the center of the second ring to the mass m is R:
U2=−G⋅4mR
5. Calculate the Work Done:
- The work done W in moving the mass m from the center of the first ring to the center of the second ring is given by the change in potential energy:
W=U2−U1
- Substituting the values:
W=(−G⋅4mR)−(−G⋅2mR)
- Simplifying:
W=−G⋅4mR+G⋅2mR=−G⋅2mR
6. Final Result:
- The work done in moving the mass m from the center of one ring to the center of the other is:
W=−2GmR
- We have two identical thin rings, each of radius R.
- The first ring has a mass m1=2m and the second ring has a mass m2=4m.
- The distance between the centers of the two rings is R.
- The gravitational potential U at a distance z from the center of a ring of mass M and radius R is given by:
U=−GM√R2+z2
- Here, G is the gravitational constant.
- At the center of the first ring (where the mass m starts), the distance z=0:
U1=−G⋅2mR
- To find the potential at the center of the second ring, we need to consider the distance from the center of the first ring to the center of the second ring, which is R.
- The distance from the center of the second ring to the mass m is R:
U2=−G⋅4mR
- The work done W in moving the mass m from the center of the first ring to the center of the second ring is given by the change in potential energy:
W=U2−U1
- Substituting the values:
W=(−G⋅4mR)−(−G⋅2mR)
- Simplifying:
W=−G⋅4mR+G⋅2mR=−G⋅2mR
- The work done in moving the mass m from the center of one ring to the center of the other is:
W=−2GmR
Topper's Solved these Questions
- BOOK - DC PANDEYCHAPTER - GRAVITATIONEXERCISE - (B) Chapter Exercises31videos
GRAVITATION
- BOOK - DC PANDEYCHAPTER - GRAVITATIONEXERCISE - (C) Chapter Exercises45videos
GRAVITATION
- BOOK - DC PANDEYCHAPTER - GRAVITATIONEXERCISE - Check Point 10.620videos
GRAVITATION
- BOOK - DC PANDEYCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE2videos
GENERAL PHYSICS
- BOOK - DC PANDEYCHAPTER - KINEMATICSEXERCISE - INTEGER_TYPE11videos
KINEMATICS
Similar Questions
Two identical thin rings, each of radius R, are coaxially placed a distance R apart. Q1 and Q2 are respectively the charges uniformly spread on the two rings. The work done in moving a charge q fróm the centre of one ring to that of the other is (√x−y)q(Q2−Q1)4√2πɛ0R. Find (x−y)
View SolutionFind the out the moment of inertia of a ring having uniform mass distribution of mass M and radius R about an axis which is tangent ot the ring and a in the plane of the ring b. perpendicular to the plane of the ring.
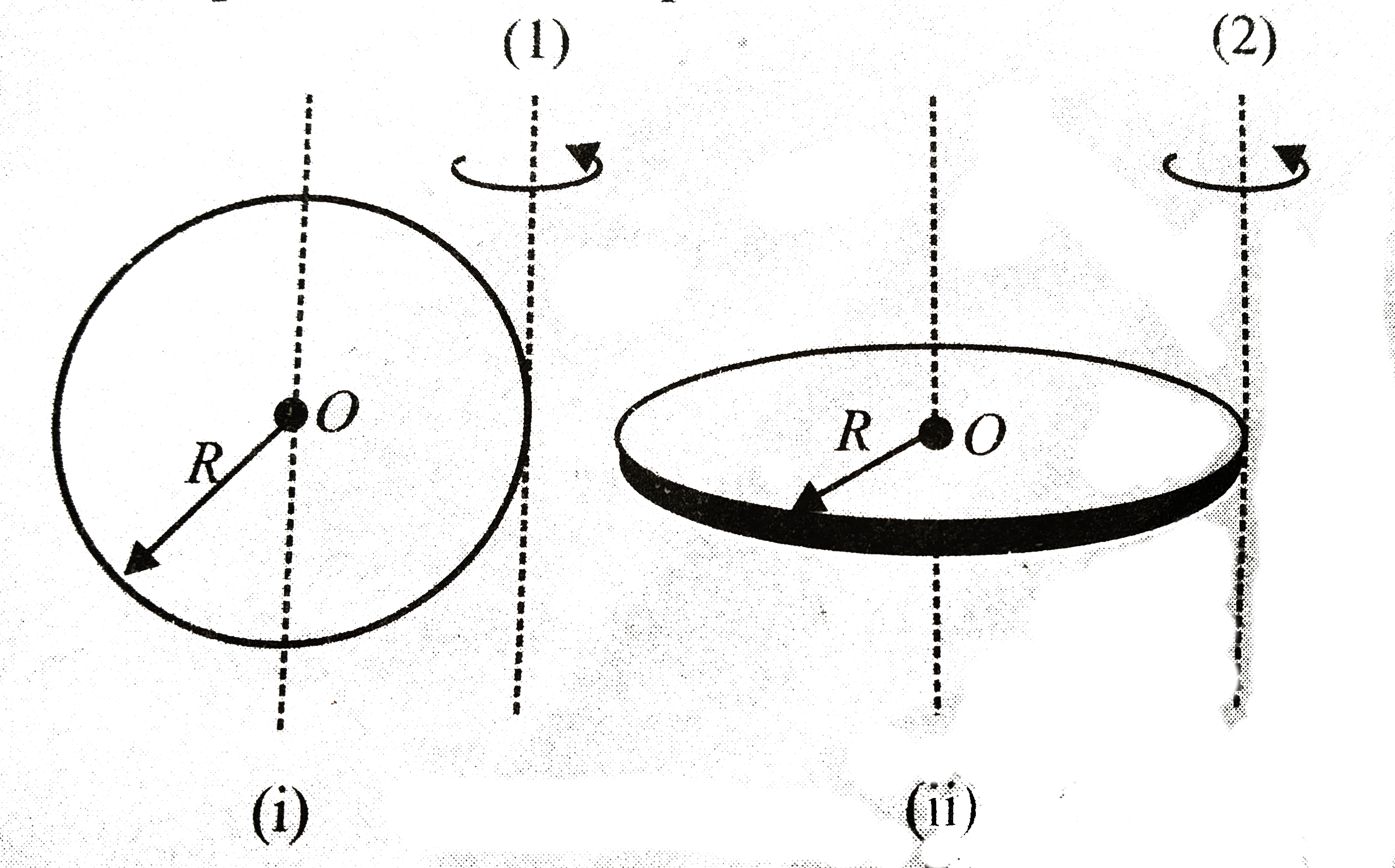 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
Two identical thin ring each of radius R are co-axially placed at a distance R. If the ring have a uniform mass distribution and each has mass m1 and m2 respectively, then the work done in moving a mass m from the centre of one ring to that of the other is :
AzeroBGm(m1−m2)(√2−1)√2RCGm√2(m1+m2)RDGm1m(√2+1)m2R - Question 2 - Select One
Two identical thin ring, each of radius R meters, are coaxially placed a distance R metres apart. If Q1 coulomb, and Q2 coulomb, are repectively the charges uniformly spread on the two rings, the work done in moving a charge q from the centre of one ring to that of the other is
A(a) zeroB(b) q(Q1−Q2)(√2−1)(4√2πε0R)C(c) q√2(Q1+Q2)(4πε0R)D(d) q(Q1+Q2)(√2+1)(4√2πε0R) - Question 3 - Select One
Three rings, each of mass m and radius r, are so placed that they touch each other. Find the moment of inertia about the axis as shown in Fig.
 A5mr2B57mr2C7mr2D72mr2
A5mr2B57mr2C7mr2D72mr2
Three rings each of mass M and radius R are placed in contact with each other as shown . Then MI of the system about YY' axis is
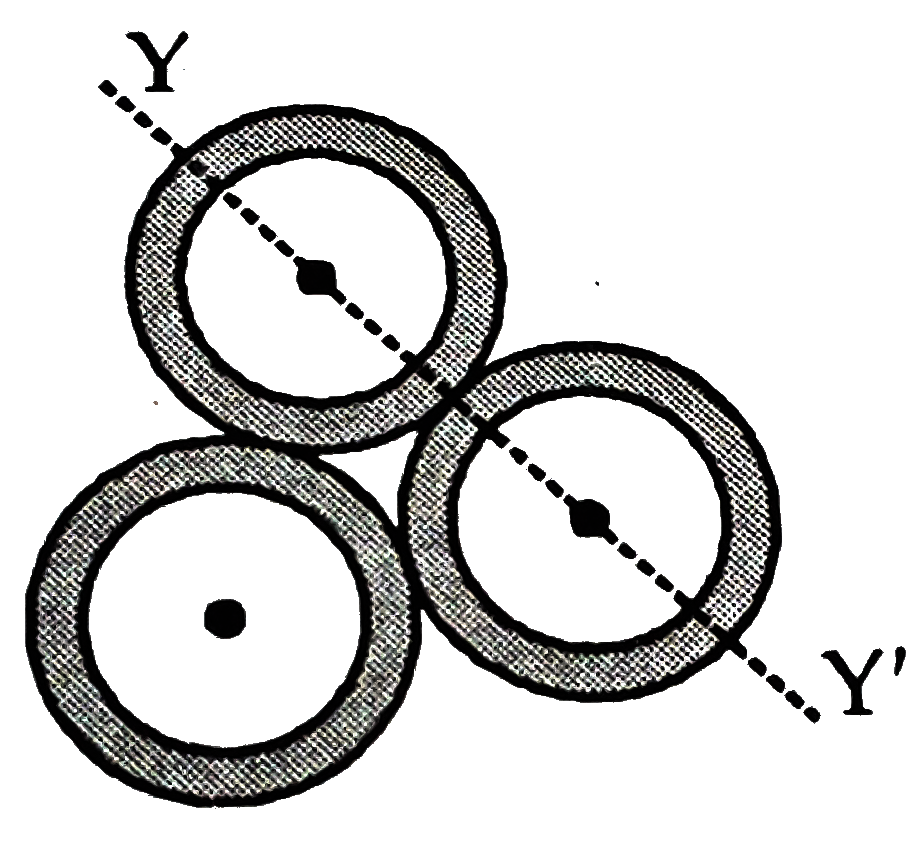 View Solution
View SolutionCalculate the moment of inertia of a ring having mass M, radius Rand having uniform mass distribution about an axis passing through the centre of the ring and perpendicular to the plane of the ring?
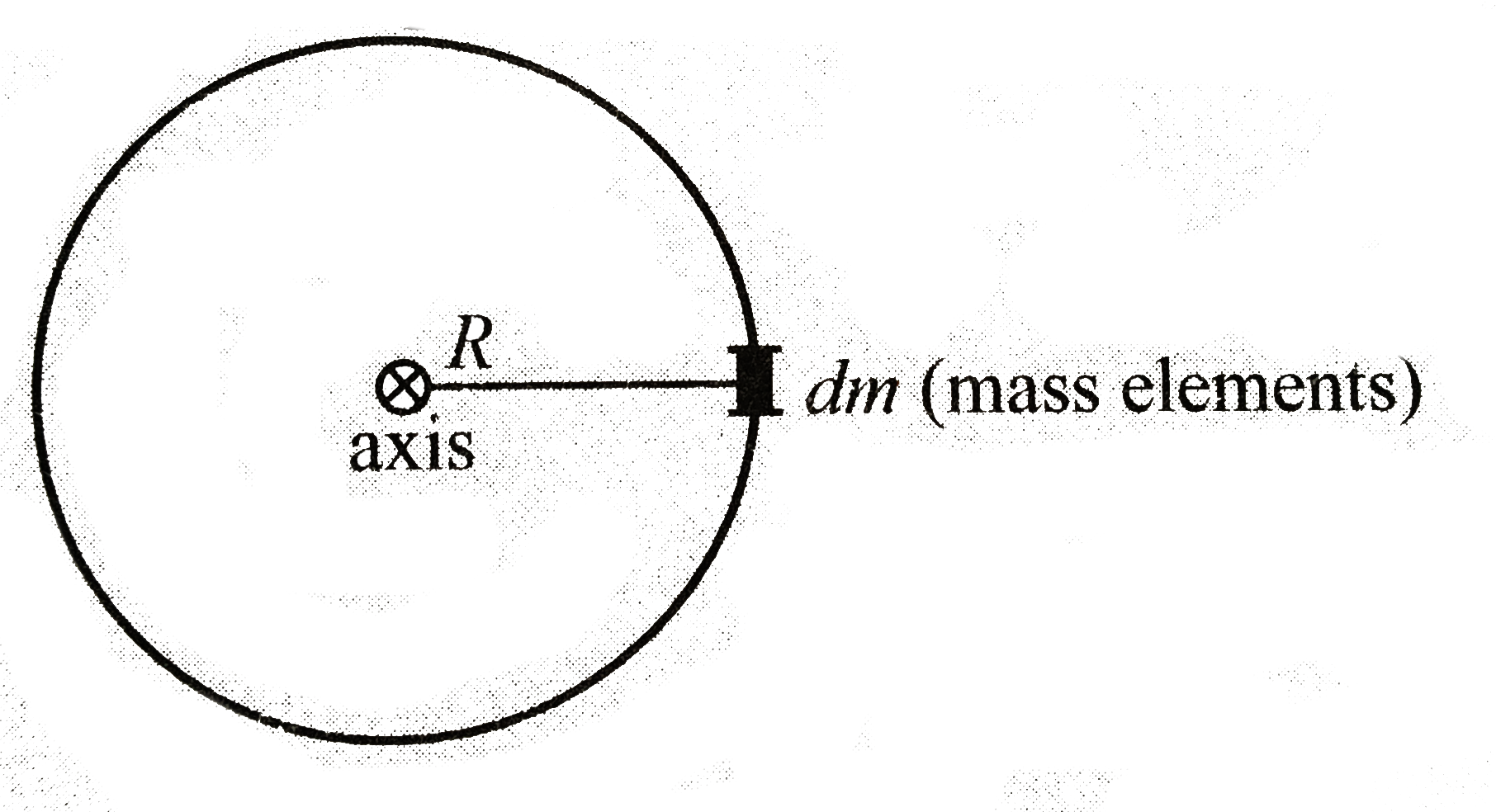 View Solution
View SolutionTwo thin wire rings each having a radius R are placed at a distance d apart with their axes coiciding. The charges on the two rings are +q and −q. The potential difference between the centres of the two rings is
View SolutionTwo identical concentric rings each of mass m and radius R are placed perpendicularly. What is the moment of inertia of the system about the axis of one of the rings ?
View SolutionTwo identical concentric rings each of mass m and radius R are placed perpendicularly. What is the moment of inertia of the system about the axis of one of the rings ?
View Solution

