Four equal masses (each of mass M) are placed at the corners of a square of side a. The escape velocity of a body from the centre O of the square is

A

$4 \sqrt{\frac{2 G M}{a}}$

B

$\sqrt{\frac{8 \sqrt{2} G M}{a}}$

C

$\frac{4 G M}{a}$

D

$\sqrt{\frac{4 \sqrt{2} G M}{a}}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

Potential at centre $= - \frac{4 G M}{r} = \frac{- 4 G M}{a / \sqrt{2}} = - \frac{4 \sqrt{2} G M}{a}$

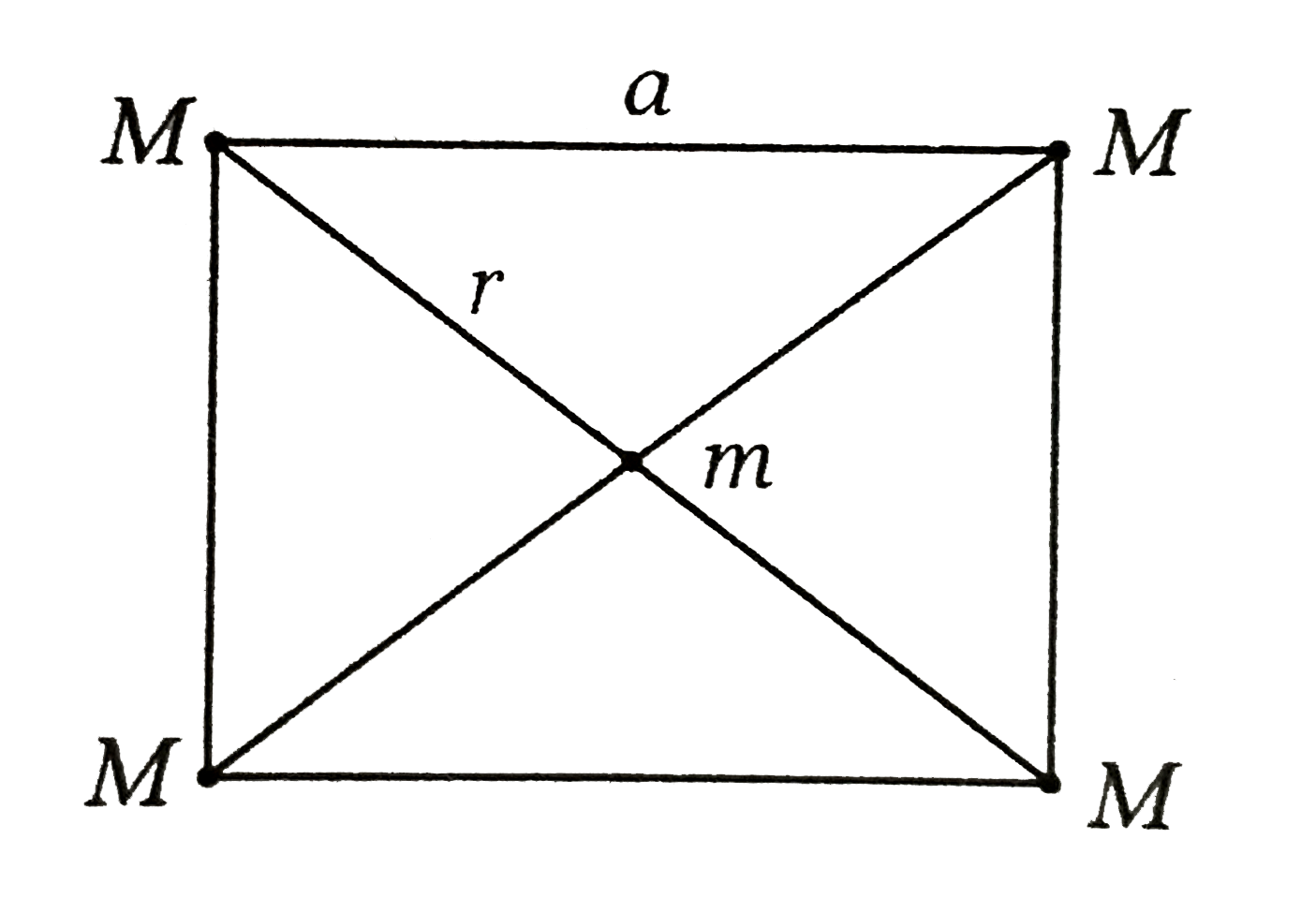
Potential energy $= \frac{- 4 \sqrt{2} G M m}{a}$
Binding energy $= \frac{4 \sqrt{2} G M m}{a}$
$∴ \frac{1}{2} m v_{e}^{2} = \frac{4 \sqrt{2} G M m}{a} or v_{e} = \sqrt{\frac{8 \sqrt{2} G M}{a}}$ .

|

Updated on:21/07/2023

Class 11 PHYSICS GRAVITATION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Four equal masses (each of mass M) are placed at the corners of a squares side a the escape velocity of a body from the centre O of the square is
A $4 \frac{\sqrt{2 G M}}{a}$
B $\frac{\sqrt{8 \sqrt{2 G M}}}{a}$
C $\frac{4 G M}{a}$
D $\frac{\sqrt{4 \sqrt{2} G M}}{a}$
Question 2 - Select One
Four particles each of mass m are placed at the vertices of a square of side $l$ . the potential at the centre of square is
A $- 2 \sqrt{2} \frac{G m}{l}$
B $- 3 \sqrt{2} \frac{G m}{l}$
C $- 2 \frac{G m}{l}$
D $- 4 \sqrt{2} \frac{G m}{l}$
Question 3 - Select One
Four identical charges i.e. $q$ is placed at the corners of a square of side $a$ . The charge $Q$ that must be placed at the centre of the square such that the whole system of charges in equilibrium is
A $\frac{q}{4}$
B $\frac{q}{4} [2 \sqrt{2} + 1]$
C $- \frac{q}{4} [2 \sqrt{2} + 1]$
D $- \frac{q}{4} [3 \sqrt{3} + 1]$

DC PANDEY-GRAVITATION-(A) Chapter Exercises

Home
Ask Doubt
Profile