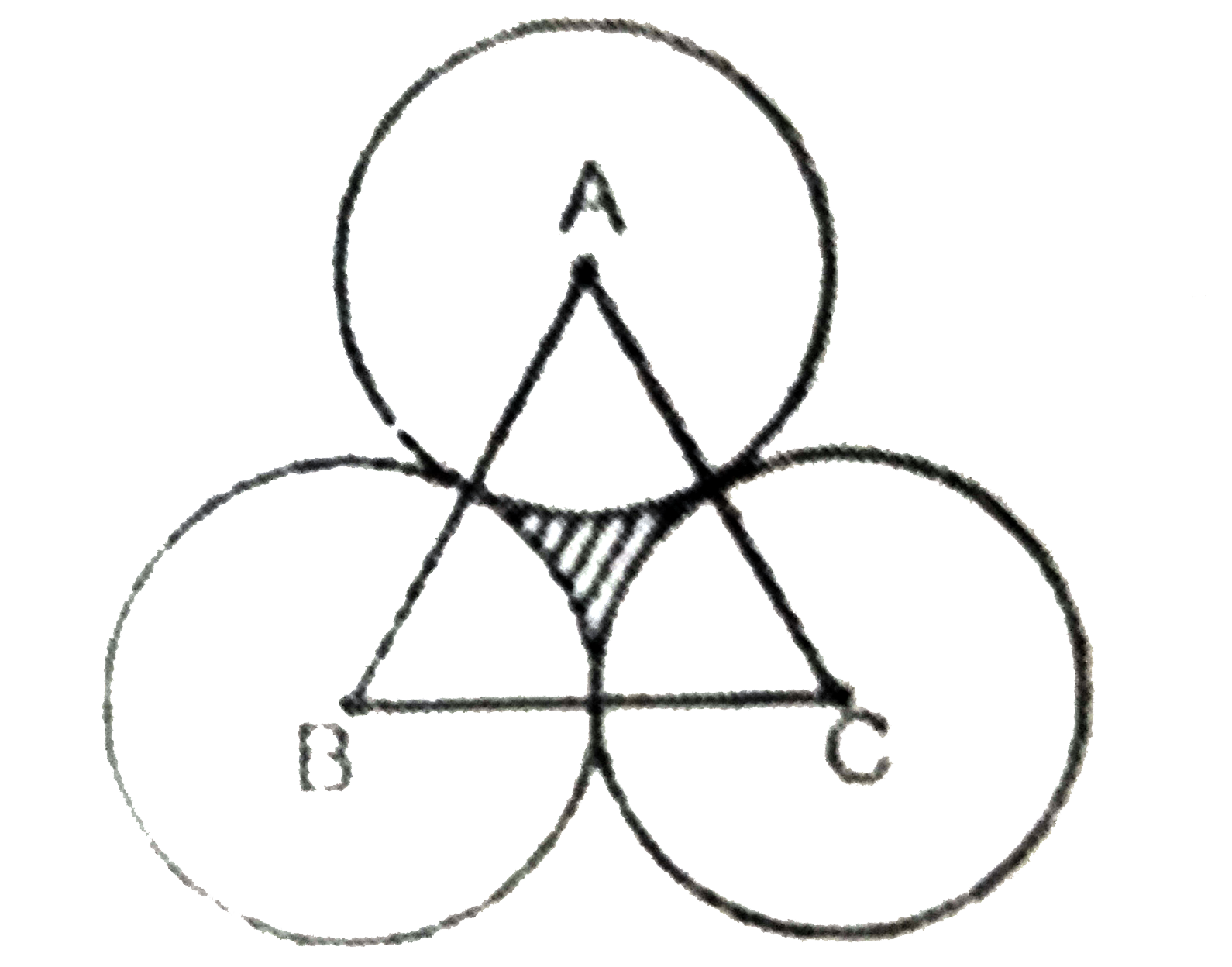
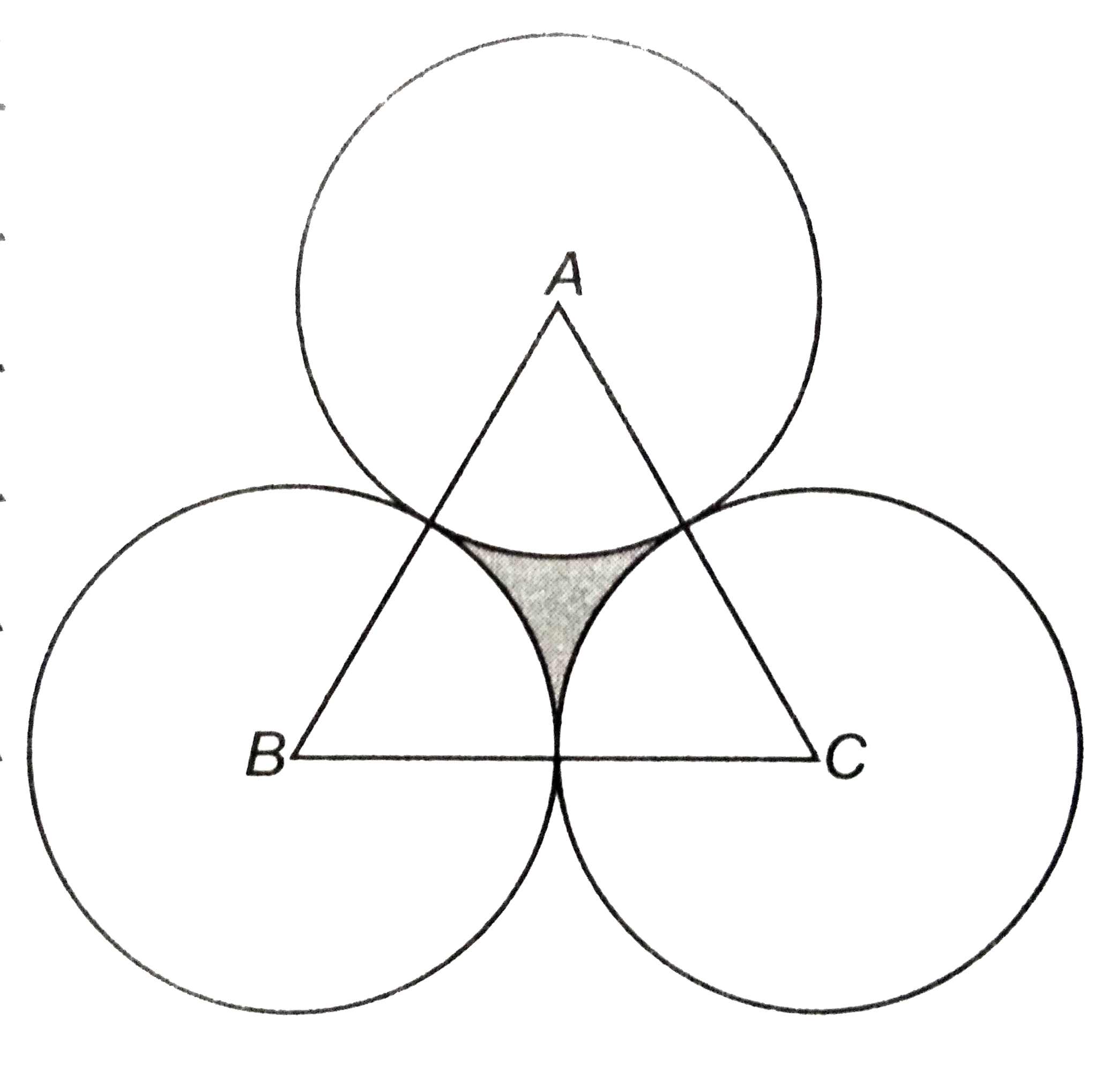
The area of an equilateral triangle ABC is 17320.5cm2. With each vertex of the trianlge as centre, a circle is drawn with radius equal to half the length of the side of the triangle. Find the area of the shaded region . Solution in Kannada
Step by step video & image solution for The area of an equilateral triangle ABC is 17320.5 cm^2. With each vertex of the trianlge as centre, a circle is drawn with radius equal to half the length of the side of the triangle. Find the area of the shaded region . by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- AREAS RELATED TO CIRCLESBOOK - SUBHASH PUBLICATIONCHAPTER - AREAS RELATED TO CIRCLESEXERCISE - EXERCISE 5.2
19 Videos
- ANNUAL EXAMINATION QUESTION PAPER JUNE-2019 (WITH ANSWERS)BOOK - SUBHASH PUBLICATIONCHAPTER - ANNUAL EXAMINATION QUESTION PAPER JUNE-2019 (WITH ANSWERS)EXERCISE - EXERCISE
35 Videos
- ARITHMETIC PROGRESSIONBOOK - SUBHASH PUBLICATIONCHAPTER - ARITHMETIC PROGRESSIONEXERCISE - Exericise 1.3
31 Videos
Similar Questions
Find the area of a sector of a circle with radius 6 cm if angle of the sector is 60∘. use π=227
View SolutionWrite the formula to find the sum of first n terms of an Arithmetic progression, whose first term is a and the last term is an.
View SolutionFind the sum of the following APs :
2,7,12,…to 10th terms.
View SolutionThe area of an equilateral triangle ABC is 17320.5 cm2. With each vertex of the triangle as centre, a circle is drawn with radius equal to half the length of the side of the triangle (see Fig. 12.28). Find the area of the shaded region. (U
View SolutionThe area of an equilateral ΔABC is 17320.5 cm2. With each vertex of the triangle as centre, a circle is drawn with radius equal to half the length of the side of the triangle (see the figure). Find the area of the shaded region. [Take π = 3.14 and √3 = 1.73205]
View SolutionFind the area of a shaded region in the figure, where a circular arc of radius 7cm has been drawn with vertex A of an equilateral triangle ABC of side 14cm as centre.
View Solutionएक समबाहु त्रिभुज ABC का क्षेत्रफल 17320.5 cm2 है। इस त्रिभुज के प्रत्येक शीर्ष को केन्द्र मानकर त्रिभुज की भुजा के आधे के बराबर की त्रिज्या लेकर एक वृत्त खींचा जाता है। छायांकित भाग का क्षेत्रफल ज्ञात कीजिए।
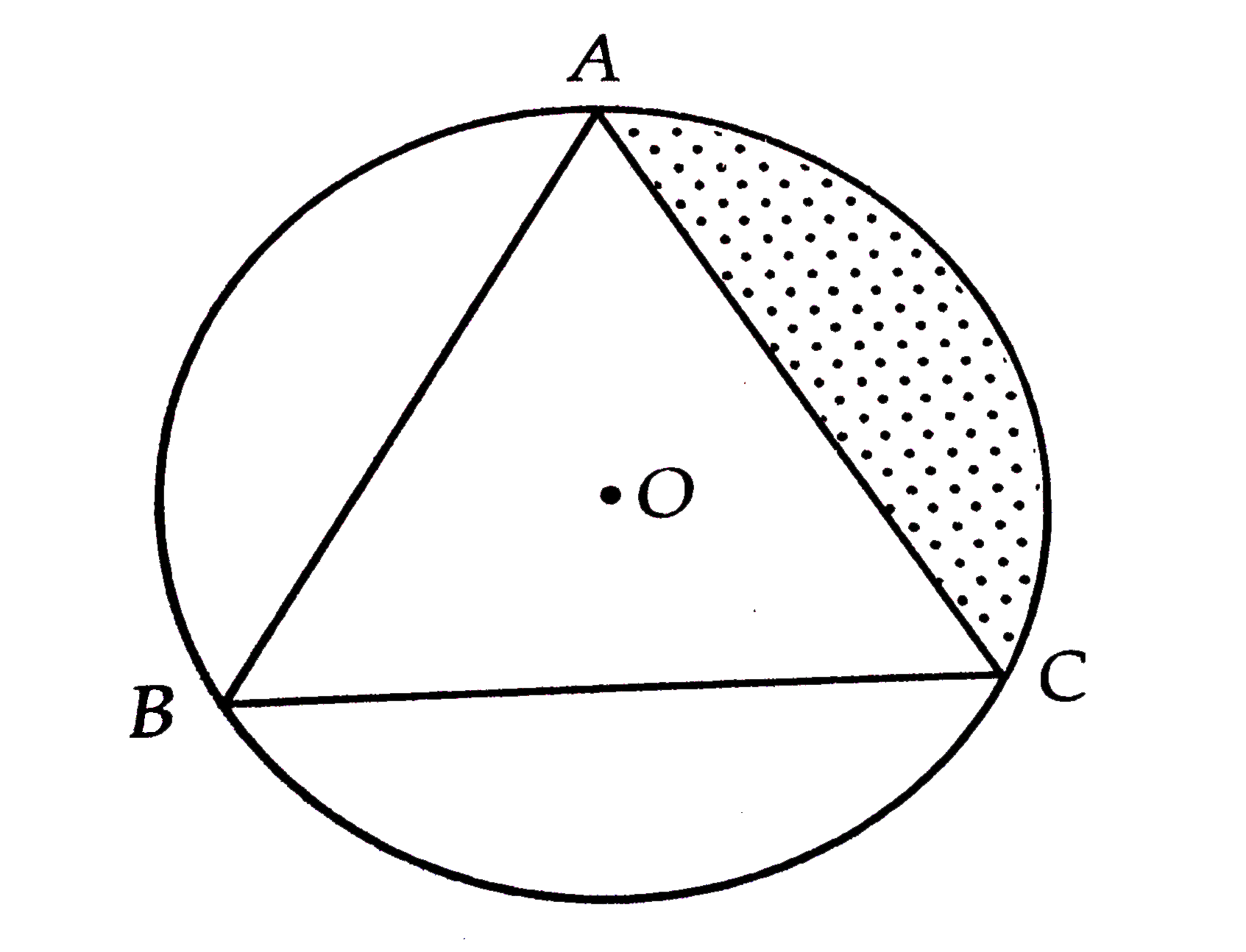
View Solutionचित्र में, केन्द्र O तथा 4 सेमी. त्रिज्या वाले वृत्त के अन्दर एक समबाहु त्रिभुज ABC बनाया गया है | छायांकित भाग का क्षेत्रफल ज्ञात कीजिए |
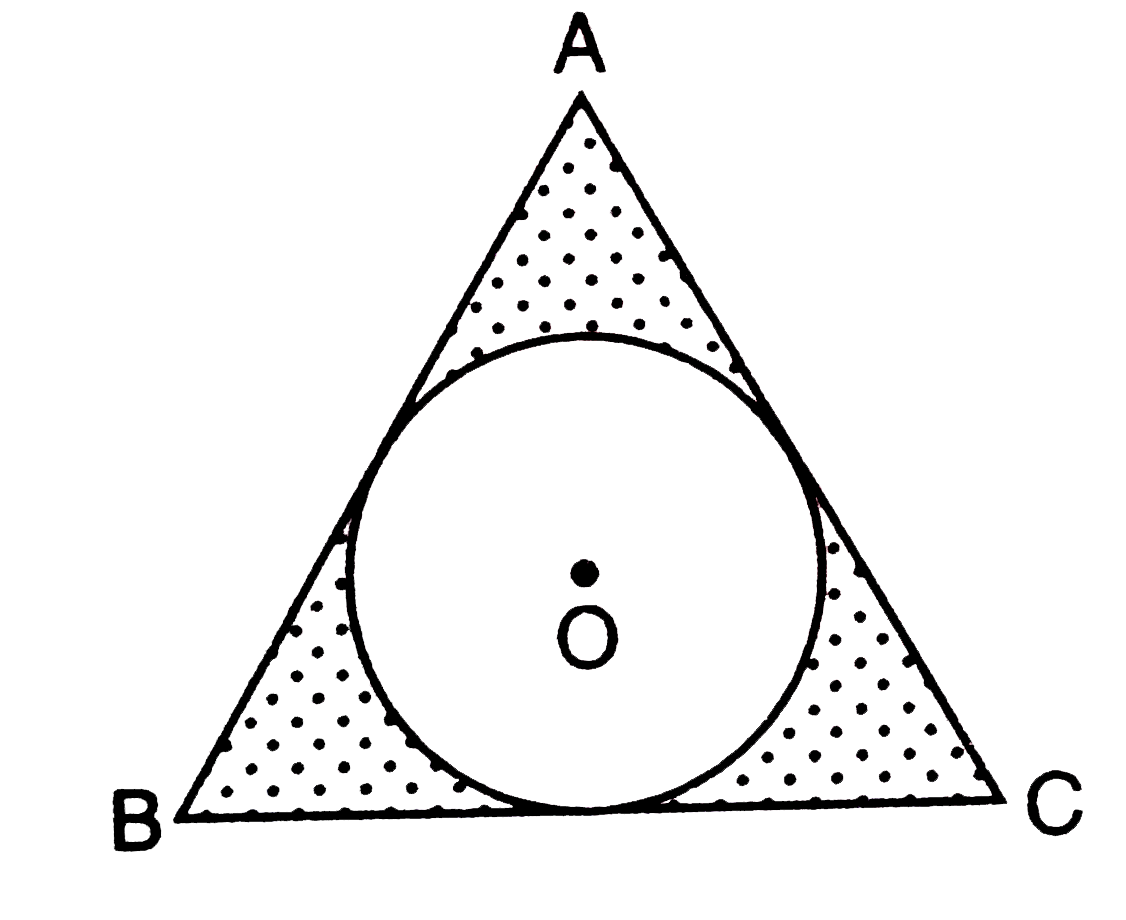
View Solutionएक 12 सेमी. भुजा वाले समबाहु त्रिभुज ABC के अन्दर एक वृत्त बनाया गया है, जो इसकी भुजाओं को स्पर्श करता है | वृत्त की त्रिज्या एवं छायांकित भाग का क्षेत्रफल ज्ञात कीजिए |
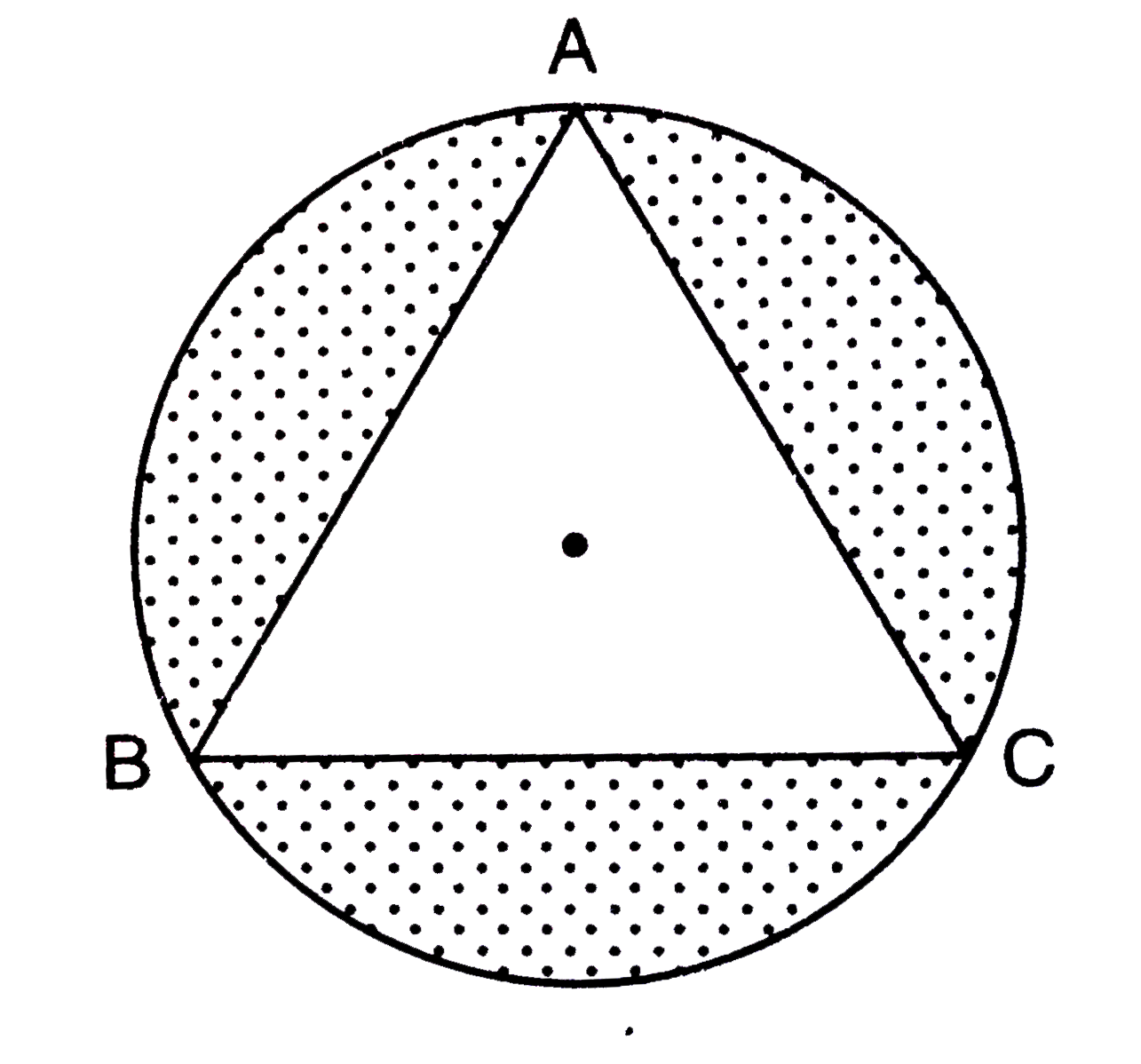
View Solutionचित्र में, 6 सेमी. भुजा वाला एक समबाहु त्रिभुज ABC एक वृत्त के अन्दर बनाया गया है | छायांकित भाग का क्षेत्रफल ज्ञात कीजिए | (π=3.14)
View Solutionएक समबाहु त्रिभुज ABC का क्षेत्रफल 17320.5 सेमी2 है । इस त्रिभुज के प्रत्येक शीर्ष को केंद्र मानकर त्रिभुज की भुजा के आधे के बराबर की त्रिज्या लेकर एक वृत्त खींचा जाता है ( देखिए आकृति )।छायांकित भाग का क्षेत्रफल ज्ञात कीजिए । ( π=3.14 और √3=1.73205 लीजिए )
View Solutionएक समबाहु त्रिभुज का क्षेत्रफल 17300cm2 है। त्रिभुज के प्रत्येक शीर्ष को केन्द्र लेकर एक वृत्त खींचा गया ह। वृत्त की त्रिज्या समबाहु त्रिभुज की भुजा की लंबाई के आधा है । त्रिभुज के उस भाग का क्षेत्रफल ज्ञात कीजिए जो वृत्त में नहीं है। (π=3.14 और √3=1.73)
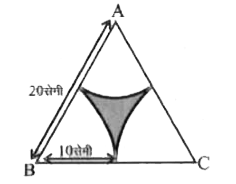
View Solutionचित्र में ABC एक समबाहु त्रिभुज है जिसकी एक भुजा 20 सेमी है। त्रिभुज के प्रत्येक शीर्ष से 10 सेमी त्रिज्या के वृत्त खींचे गये हैं। छायांकित भाग का क्षेत्रफल ज्ञात कीजिए। (π=3.14 लीजिए)
View SolutionThe area of an equilateral triangle ABC is 17320.5 cm2. With each vertex of the triangle as centre, a circle is drawn with radius equal to half the length of the side of the triangle (see Fig. 12.28). Find the area of the shaded region. (Use π=3.14and√3= 1.73205)
View SolutionThe area of an equilateral triangle ABC is 17320.5cm2. With each vertex of the trianlge as centre, a circle is drawn with radius equal to half the length of the side of the triangle. Find the area of the shaded region .
View SolutionArea of an equilateral triangle is 17300 cm2. By taking each vertex of the triangle as centre, a circle is drawn at each vertex. The radius of thecircleis half of the length of the side of an equilateral triangle. Find the area of that portion of the triangle which is not included in the circle. (π=3.14 and √3=1.73)
View Solution
