The number of ways of factoring 91,000 into two factors m & n such that m>1,n>1 and ged (m,n)=1 is
A
70
B
15
C
32
D
None of these
Text Solution
The correct Answer is:D
91000=13.7.1000=13.7.103
∴91000=23.53.13.7
[m=2a.5b.7c.13dn=2p.5q.7r.13s
gcd (m,n)=2∘5∘7∘13∘
N=2.2.2.22−1 (When 91,000 & 1) = 7
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
The number of ways of factoring 91,000 into two factors, m and n, such that m>1,n>1 and gcd (m, n) = 1 is
A7B15C32D37The sum of all natural numbers 'n' such that 100<n<200andHCF(91,n)>1 is
A3203B3303C3221D3121In which of the following, m>n(m,n∈R)?
Am=(log25)2andn=log220Bm=log102andn=log103√10Cm=log105⋅log1020andn=1Dm=log1/2(13)andn=log1/3(12)
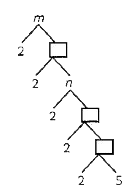
In the adjoining factor tree, find the numbers m and n
View SolutionThe number of solutions of the equation n−|x||m−|x∣∣=1 (where m,n>1 and n>m) is
View SolutionThe number of ways in which m men and n women can be seated in a row, so that no two women sit together is
(i) m!(m+1)!(m+n−1)!
(ii) m!(m+1)!(m−n+1)!
(iii) n!(m+1)!(m−n+1)!
(iv) m!(n+1)!(m+n−1)!
View SolutionThe total number of injective mappings from a sct with m elements to a set with n elements for, m>n, is :
View SolutionIf m,n are the positive integers (n>1) such that mn=121 , then value of (m−1)n+1 is :
View Solution
