A stell rod of length 2l corss sectional area A and mass M is set rotating in a horizotnal plane about an axis passing through its centre and perpendicular to its length with constant angular velcoty $ω .$ If Y is the Young's modulus for steel, find the extension in the lenght of the rod. (Assume the rod is uniform.)

Video Solution

Text Solution

Verified by Experts

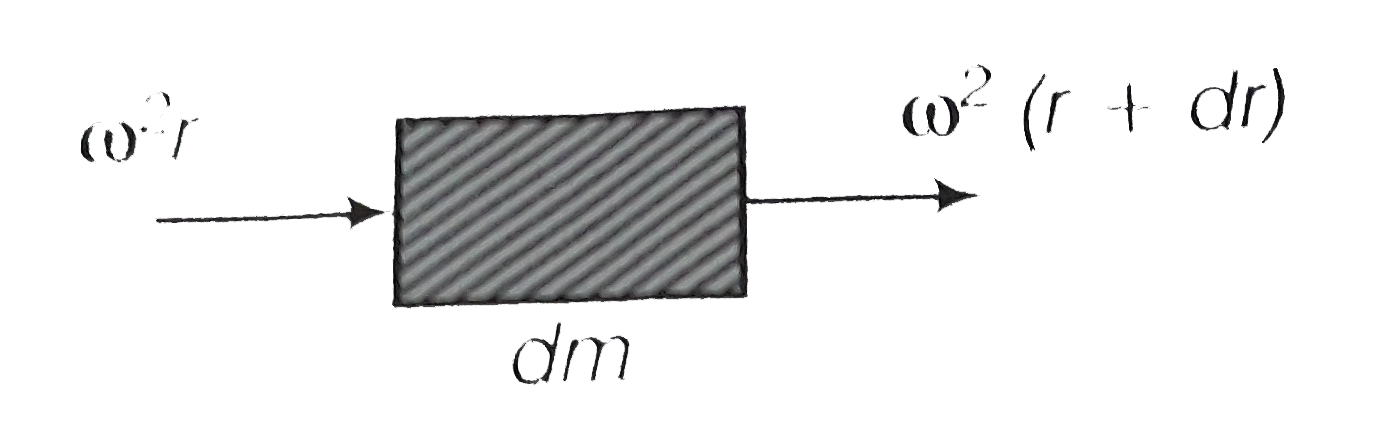
Consider an element of width dr at r as shown in the diagram.
Let T(r ) and T (r + dr) be the tensions at r and r + dr respectively.
Net centrifugal force on the element $= ω^{2}$ rdm (where $ω$ is angular velocity of the rod)
$= ω^{2} r μ d r (∵ μ = mass / length)$
$\Rightarrow T (r) = T (r + d r) = μ ω^{2} r d r$
$\Rightarrow - d T = μ ω^{2} r d r$
$\Rightarrow [∵ Tension and centrifugal forces are opposite]$
$∴ - T \int T = 0 d T = r = r \int r = l μ ω^{2} r d r [∵ T = 0 at r = l]$
$\Rightarrow T (r) = \frac{μ ω^{2}}{2} (l^{2} - r^{2})$

So, Young's modulus Y $= \frac{Stress}{Strain} = \frac{T (r) / A}{\frac{Δ r}{d r}}$
$∴ \frac{Δ r}{d r} = \frac{T (r)}{A} = \frac{μ ω^{2}}{2 Y A} (l^{2} - r^{2})$
$∴ Δ r = \frac{1}{Y A} \frac{μ ω^{2}}{2} (l^{2} - r^{2}) d r$
$∴ Δ$ = change in length in right part $= \frac{1}{Y A} \frac{μ ω^{2}}{2} l \int 0 (l^{2} - r^{2}) d r$
$= (\frac{1}{Y A}) \frac{μ ω^{2}}{2} [l^{3} - \frac{l^{3}}{3}] = \frac{1}{3 Y A} μ ω^{2} L^{2}$
$∴$ Total change in length $= 2 Δ = \frac{2}{3 Y A} μ ω^{2} l^{2}$

Updated on:21/07/2023