A stone of mass m is tied to an elastic string of negligble mass and spring constant k. The unstretched length of the string is L and has negligible mass. The other end of the string is fixed to a nail at a point p. Initially the stone is at the same level as the point P. The stone is dropped vertically from point P.
(a) Find the distance y from the top when the mass comes to rest for an instant, for the first time.
(b) What is the maximum velocity attained by the stone in this drop?
(c) What shall be the nature of the motion after the stone has reached its lowest point?
Consider the diagram the stone is dropped from point p.
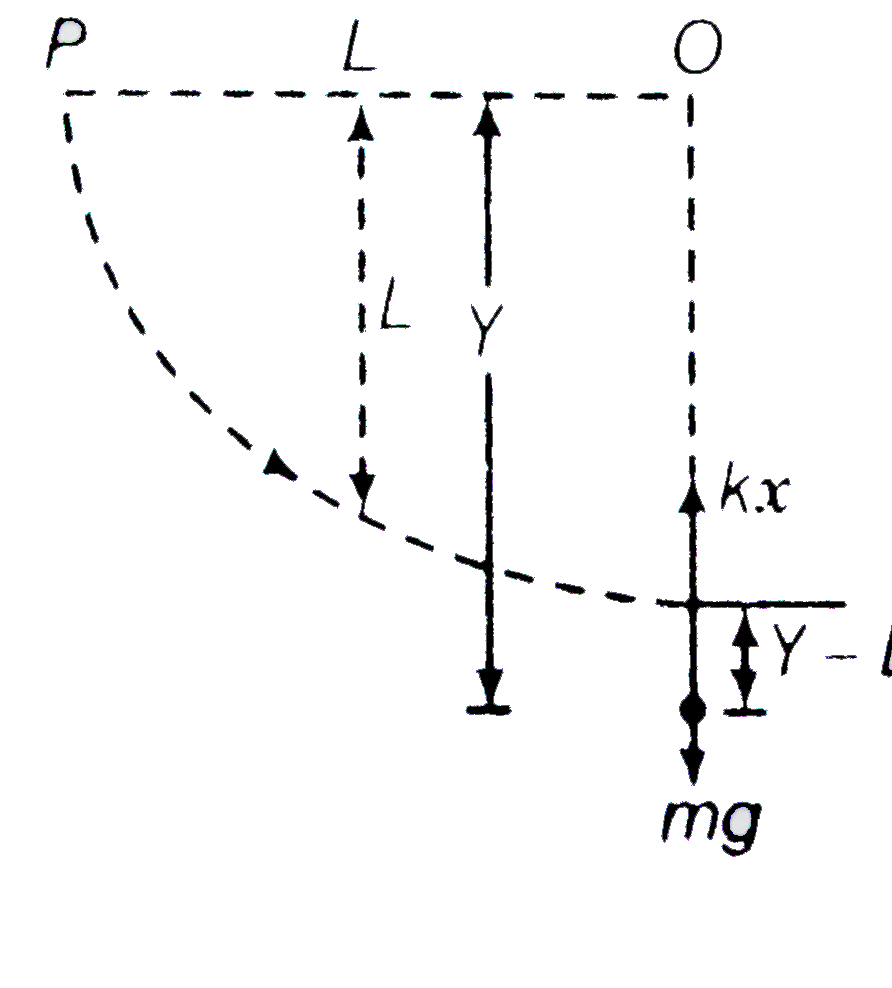
Till the stone drops through a length L it will be in free fall.
After that the elasticity of the string will force it to a SHM.
Let the stone come to rest instantaneously at y.
The loss in PE of the stone is the PE stored in the stretched string.
mgy=12k(y−L)2
⇒ mgy=12ky2−kyL+12kL2⇒12ky2−(kL+mg)y+14kL2=0
y=(kL+mg)±√(kL+mg)2−k2L2k=(kL+mg)±√2mgkL+m2g2k
Retain the positive sign.
∴ y=(kL+mg)+√2mgkL+m2g2k
(b) In SHM, the maximum velocity is attained when the body passes, through the ''equilibrium, position'' i.e., when the instantaneous acceleration is zero. That is mg−kx=0, where x is the extension from L.
⇒ mg=kx
Let the velocity be v. Then,
12mv2+12kx2=mg(L+x) (from conservation of energy)
12mv2=mg(L+x)−12kx2
Now, mg=kx⇒x=mgk
∴ 12mv2=mg(L+mgk)−12km2g2k2=mgL+m2g2k−12m2g2k
12mv2=mgL+12m2g2k
∴ v2=2gL+mg2/k
v=(2gL+mg2/k)1/2
When stone is at the lowest position i.e., at instantaneous distance y from p, then equation of motion of the stone is
md2ydt2=mg−k(y−L)⇒d2ydt2+km(y−L)−g=0
Make a transformation of variables, z=km(y−L)−g
∴ d2zdt2+kmz=0
It is differential equation of second order which represents SHM.
Comparing with equation d2zdt2+ω2z=0
Angular frequency of harmonic motion ω=√km
The solution of above equation will be of the type z=Acos(ωt+ϕ), where ω=√km
y=(L+mgk)+Acos(ωt+ϕ)
Thus, the stone will perform SHM with angular frequency ω=√k/m about a point y0=L+mgk.
Topper's Solved these Questions
- BOOK - NCERT EXEMPLARCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - LONG SHORT ANSWER TYPE QUESTION16videos
MECHANICAL PROPERTIES OF SOLIDS
- BOOK - NCERT EXEMPLARCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - Long Answer Type Questions3videos
MECHANICAL PROPERTIES OF FLUIDS
- BOOK - NCERT EXEMPLARCHAPTER - MOTION IN A PLANEEXERCISE - Multiple Choice Questions37videos
MOTION IN A PLANE
Similar Questions
A stone of mass m tied to a string of length l is rotated in a circle with the other end of the string as the centre. The speed of the stone is v. If the string breaks, the stone will move
View SolutionA stone of a mass m tied to a string of length /is rotated in a circle with the other end of the string as the centre. The speed of the stone is V. If the string breaks, the stone will
View Solution
Knowledge Check
- Question 1 - Select One
A stone of mass m is tied to a string of length l and rotated in a circle with a constant speed v . If the string is released, the stone flies
ARadially outwardBRadialy inwardCTangentially outwardDWith acceleration mv2l - Question 2 - Select One
A stone of mass ied to a string of lengh l rotating along & a circular path with constant speed v. The torque on the stone is
Amv2lBmvlCmvlDzero - Question 3 - Select One
A body of mass m is attached by an inelastic string to a suspended spring of spring constant k. Both the string and the spring have negligible mass and the string is inextensible and of length L. Initially, the mass m is at rest.
The largest amplitude Amax, for which the string will remain taut throughout the motion isAmg2kBmgkC2mg3kDL
A stone of mass m tied to a string of length l rotates along circumference of a circle with constant speed v. The torque on the stone is
View Solution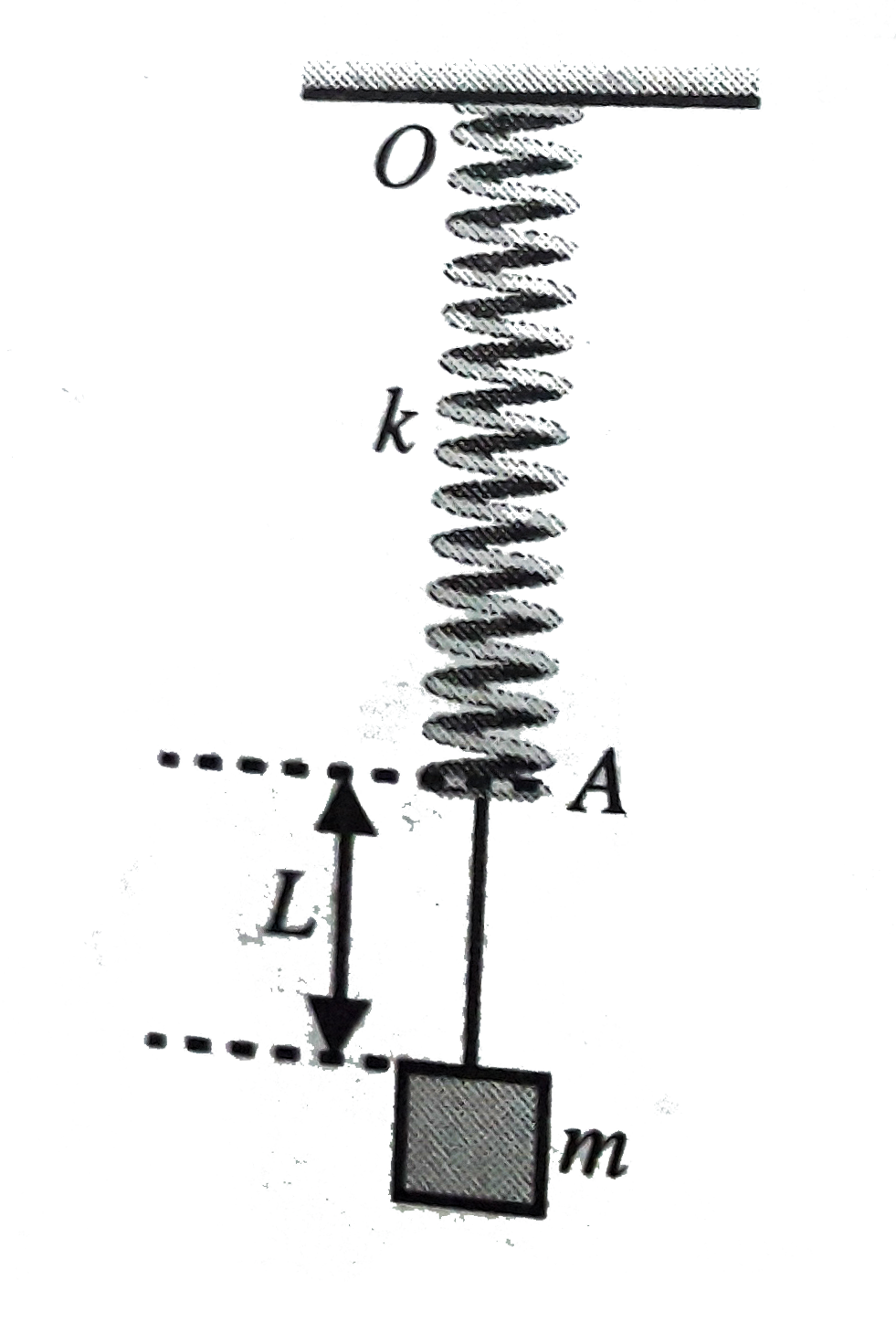
A body of mass m is attached by an inelastic string to a suspended spring of spring constant k. Both the string and the spring have negligible mass and the string is inextensible and of length L. Initially, the mass m is at rest.
Q. If the mass m is now raised up to point A (the top end of the string see fig. and allowed to fall from rest, the maximum extension of the spring in the subsequent motion will beView SolutionWhen a stone tied to a string is whirled in a circle, the work done on it by the string is
View SolutionA body of mass m is attached by an inelastic string to a suspended spring of spring constant k. Both the string and the spring have negligible mass and the string is inextensible and of length L. Initially, the mass m is at rest.
If the mass m, from the initial position of rest is pulled down a distance A and then released, assuming that the string remains taut throughout the motion, the maximum (downwards) acceleration of the oscillating body will beView SolutionA stone is tied to the end of a string of length 1 and whirled in a horizental circle. When the string breaks then stone
View Solution

