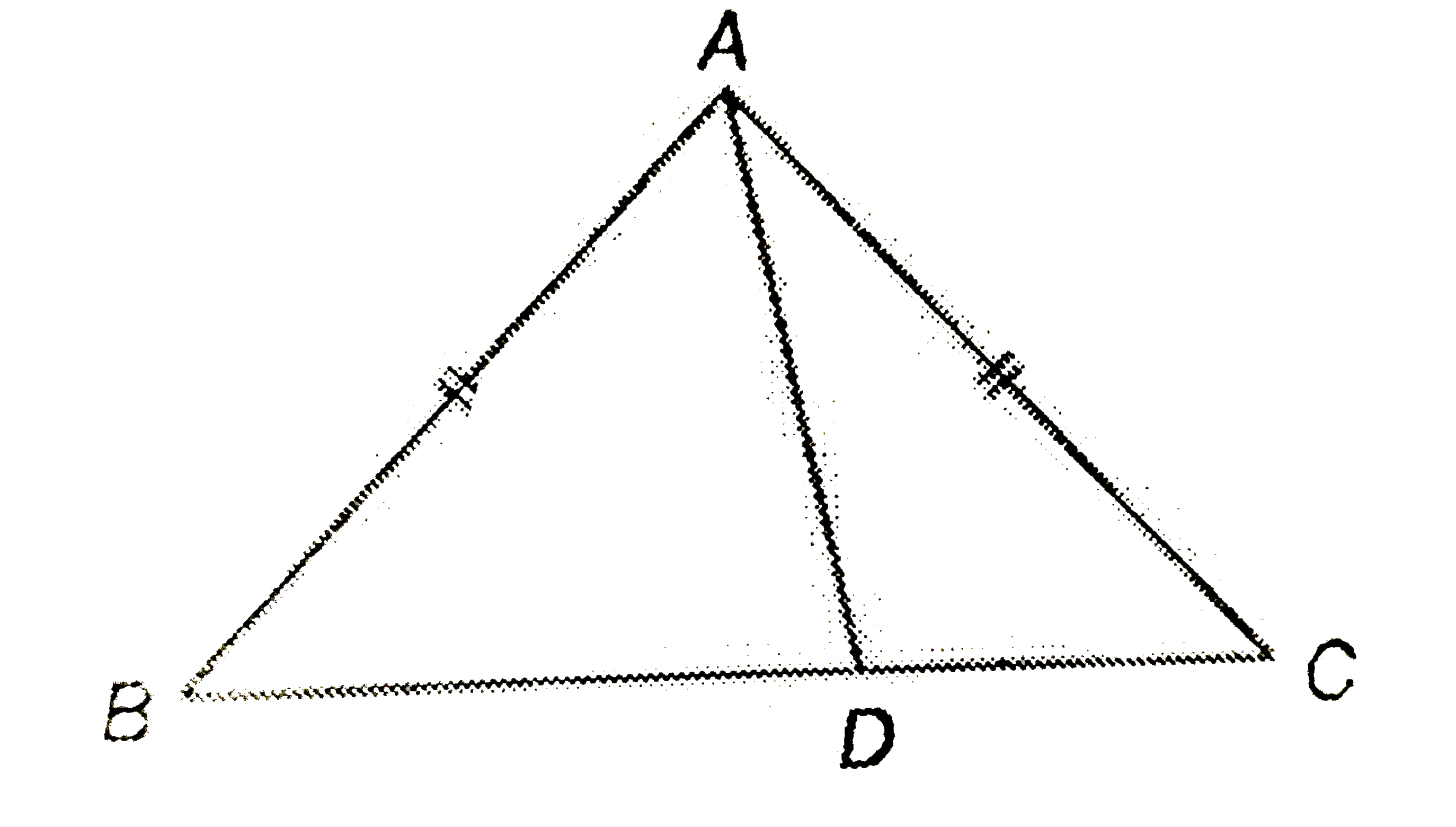
If D is any point on the base BC produced, of an isosceles triangle ABC , prove that AD>AB.
In △ABC
we haveAB=AC
∠ABC=∠ACB .....(1)
[ since Angle opp. to equal sides are equal]
In △ABD
we have Ext∠ABC>∠ADB
this implies∠ABC>∠ADB.....(2)From (1) and (2), we get∠ACB>∠ADB
∠ACD>∠ADC
[ since∠ACB=∠ACD,∠ADB=∠ADC]
this implies AD>AC
[since side opp. to greater angle is larger]
this implies AD>AB [since AB=AC]
Topper's Solved these Questions
Similar Questions
If D is any point on the base BC produced, of an isosceles triangle ABC, prove that AD>AB .
View SolutionIf D is any point on the base BC produced, of an isosceles triangle ABC , prove that AD>AB.
View SolutionIf D any point on the BC of an isosceles triangle ABC then prove that AB>AD and AC>AD.
 View Solution
View SolutionIf D is a point of the base BC of an isosceles triangle ABC such that AD is perpendicular to AC, then a=
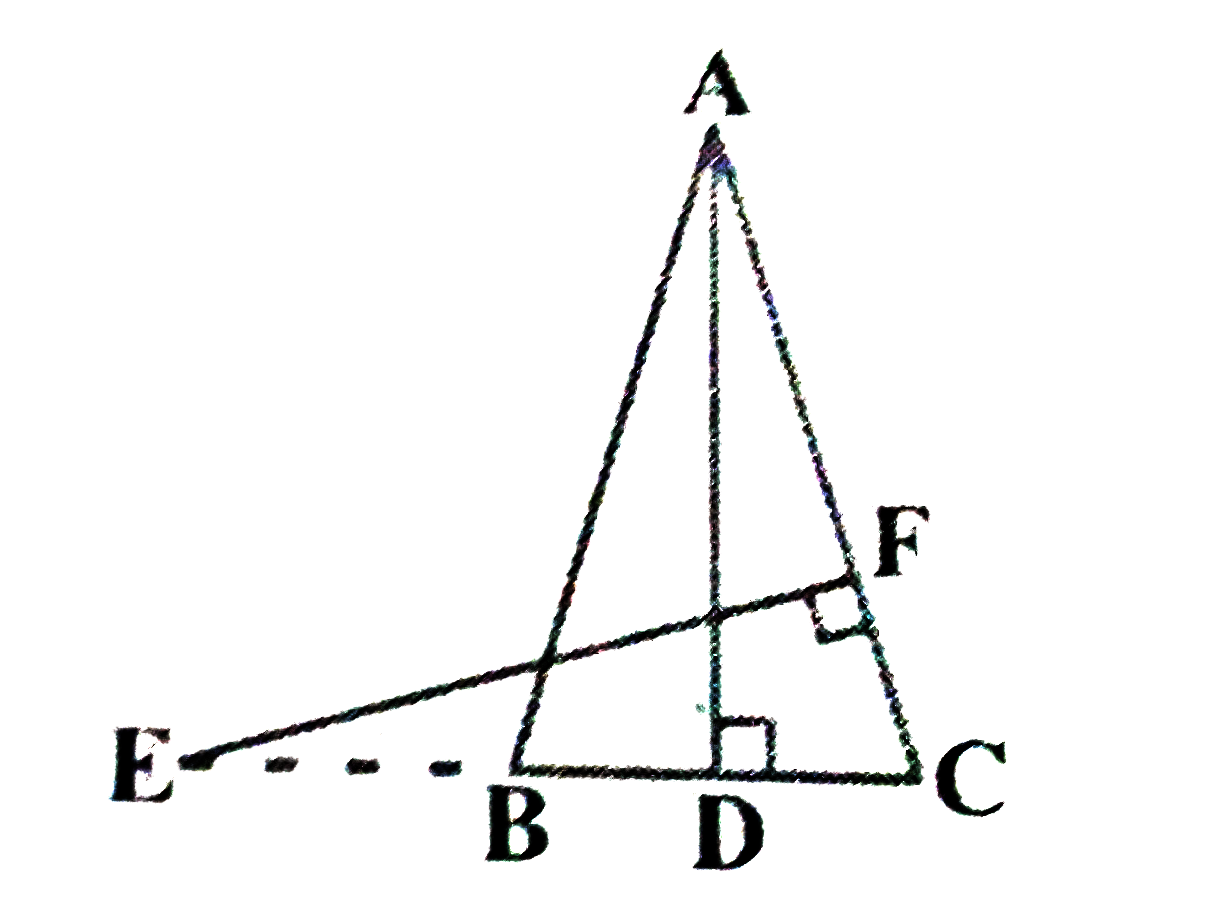
View SolutionIn Fig. 4.124, E is a point on side CB produced of an isosceles triangle ABC with AB=AC . If AD⊥BC and EF⊥AC , prove that (i) ABDECF (ii) AB×EF=AD×EC . (FIGURE)
View SolutionIn figure E is a point on side CB produced of an isosceles triangle ABC with AB = AC. If AD⊥BCandEF⊥AC, prove thatΔABD~ΔECF.
 View Solution
View SolutionIf D is the mid-point of the hypotenuse AC of a right triangle ABC , prove that BD=12AC . GIVEN : A ABC in which ∠B=900 and D is the mid-point of AC. TO PROVE : BD=12AC CONSTRUCTION Produce BD to E so that BD=DE. Join EC.
View SolutionDandE are points on equal sides ABandAC of an isosceles triangle ABC such that AD=AE . Prove that B,C,D,E are concylic.
View SolutionD and E are points on equal sides AB and AC of an isosceles triangle ABC such that AD=AE. Prove that B, C, D, E are concyclic.
View SolutionO is any point on the diagonal BD of the parallelogram ABCD. Prove that ar(OAB)=ar(OBC)
View Solution

