Question

The median BE and CF of a triangle ABC intersect at G. Prove that the area of ${\displaystyle \Delta GBC=}$ area of the quadrilateral AFGE.

Explanation

Given In `DeltaABC`, medians BE and CF intersect each other at G. To prove `ar (DeltaGBC) = ar (AFGE)`
Proof Since, BE is the median of `DeltaABC` and we know that a median of a triangle divides it into two parts of equal area.

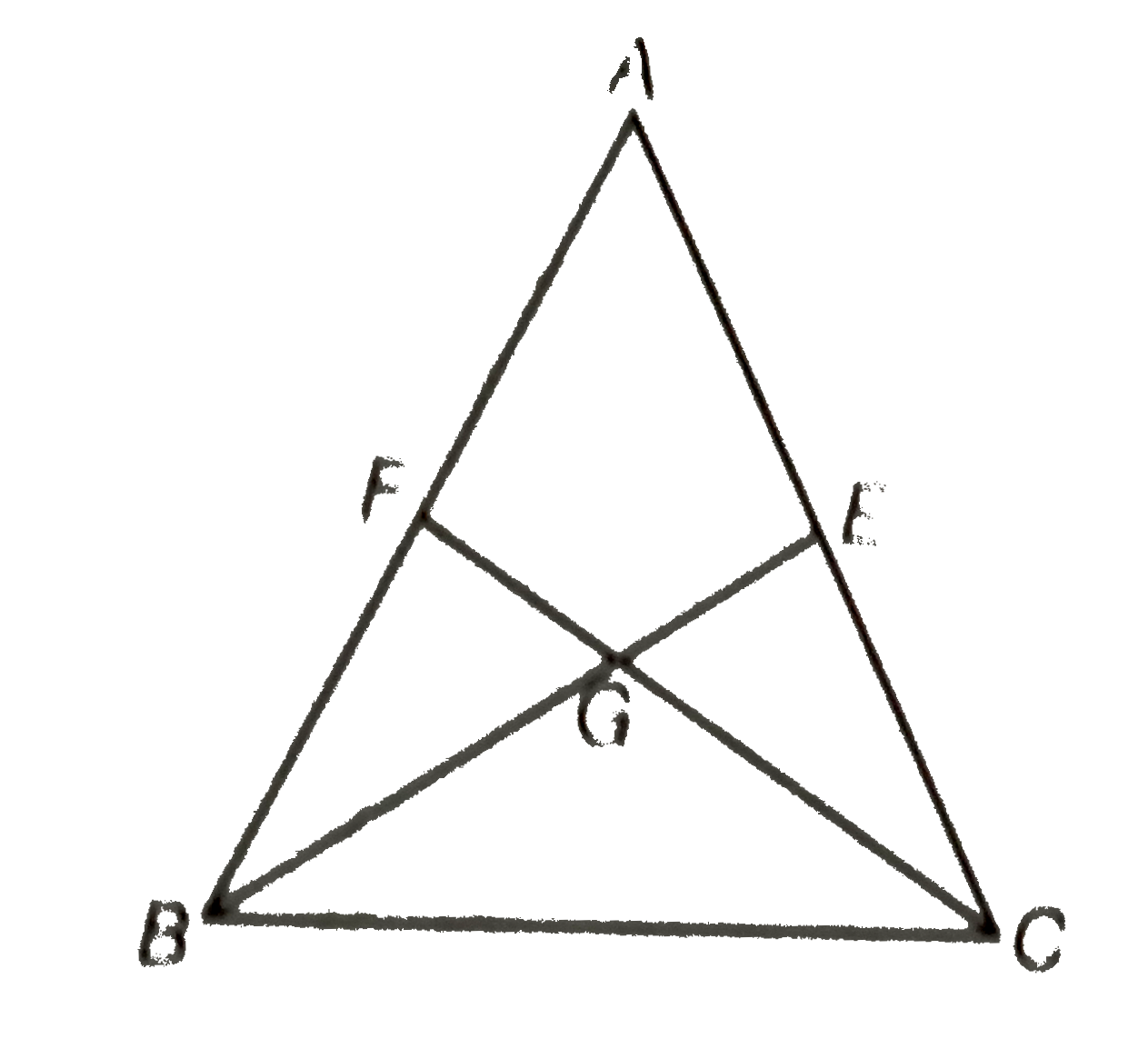
So, `" " ar (DeltaABE) = ar (DeltaCBE)`
`rArr" "` `ar (DeltaABE) = (1)/(2)ar (DeltaABC)" "` ...(i)
Similarly, CF is the median of `DeltaABC`.
Then, `" "ar (DeltaACF) = ar (DeltaBCF)`
`rArr" "` `ar (DeltaBCF) = (1)/(2)ar (DeltaABC)" "` ...(ii)
From Eqs. (i) and (ii),
`ar (DeltaABE) = ar (DeltaBCF)" "` ...(iii)
On substracting `ar (DeltaGBF)` from both sides of Eq. (iii), we get
`ar (DeltaABE) - ar (DeltaGBF) = ar (DeltaBCF) - ar (DeltaGBF)`
`rArr" "` `ar (AFGE) = ar (GBC)" "` Hence proved.