In ΔABC , if L and M are the points on AB and AC, respectively such that LM∣∣BC. Prove that ar(ΔLOB)=ar(ΔMOC).
Video Solution
Text Solution
Given In ΔABC, L and M are points on AB and AC respectively such that LM∣∣BC.
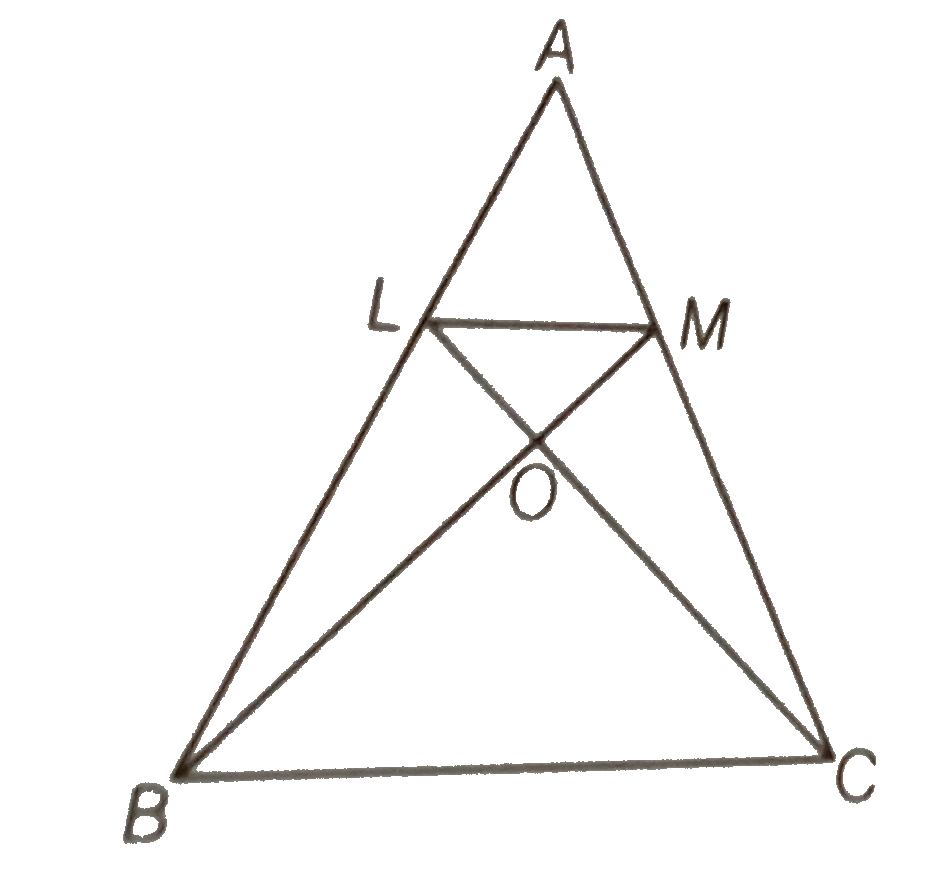
To prove ar(ΔLOB)=ar(ΔMOC)
Proof We know that, triangles on the same base and between the same parallels are equal in area.
Hence, ΔLBC and ΔMBC lie on the same base BC and between the same parallels BC and LM.
So, ar(ΔLBC)=ar(ΔMBC)
⇒ ar(ΔLOB)+ar(ΔBOC)=ar(ΔMOC)+ar(ΔBOC)
On eliminating D ar(ΔBOC) from both sides, we get
ar(ΔLOB)=ar(ΔMOC) Hence proved.
|
Updated on:21/07/2023
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
In ΔABC, D and E are points on AB and AC respectively such that DE||BC and DE divides the ΔABC into two parts of equal areas. Then ratio of AD and BD is
A1:1B1:√2−1C1:√2D1:√2+1 - Question 2 - Select One
In ΔABC, D and E are points on AB and AC respectively such that DE is parallel to BC. If AD= 2 cm, BD = 3 cm, then ar(ΔADE)ar(ΔABC) is
A49B1681C425D25 - Question 3 - Select One
L and M are the mid points of AB and BC respectively of ΔABC , right angled at B . 4LC2=
 AAB2+4BC2BBC2+4AB2CAC2+4AB2DNone of these
AAB2+4BC2BBC2+4AB2CAC2+4AB2DNone of these
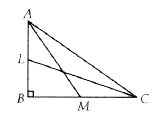
A point D is taken on the side BC of a ΔABC such that BD=2DC. Prove that ar(ΔABD)=2ar(ΔADC)
View SolutionABCD is a parallelogram. L and M are points on AB and DC respectively and AL=CM. prove that LM and BD bisect each other.
View SolutionIn the given Fig. 3, in ΔABC,DandE are the mid point of the sides AB and AC respectively. Find the length of DE. Prove that DE=12BC.
 View Solution
View SolutionIn the given figure, ABCD is a parallelogram. E and F are any two points on AB and BC, respectively. Prove that ar(△ADF)=ar(△DCE).
View SolutionIn ΔABC, two points D and E are taken on the lines AB and BC respectively in such a way that AC is parallel to DE. Then ΔABCandΔDBEare
View Solution