A random variable X as the following probability distribution: xi:−2−10123 pi:0.1k0.22k0.3k Find (i) Find the value of k. (ii) Calculate the mean ofX. Calculate the variance of X.
∑Pi=1
0.1+k+0.2+2k+0.3+k=1
0.6+4k=1
4k=0.4
k=0.1
Now, ¯x=∑Xip1
¯x=−0.2+(−0.1)+0+0.2+0.6+0.3
=−0.3+1.1
=0.8
now, var(x)=∑x21pi−(∑X1Pi)2
∑X2iPi=0.4+0.1+0+0.2+1.2+0.9=2.8
Var(X)=2.8−(0.8)2
2.8−0.64=2.16
Topper's Solved these Questions
Similar Questions
A random variable X as the following probability distribution: xi:−2−10123 pi:0.1k0.22k0.3k Find (i) Find the value of k. (ii) Calculate the mean ofX. Calculate the variance of X.
View SolutionA random variable X has the following probability distribution find K.
 View Solution
View SolutionA random variable X has the follwing probability distribution:
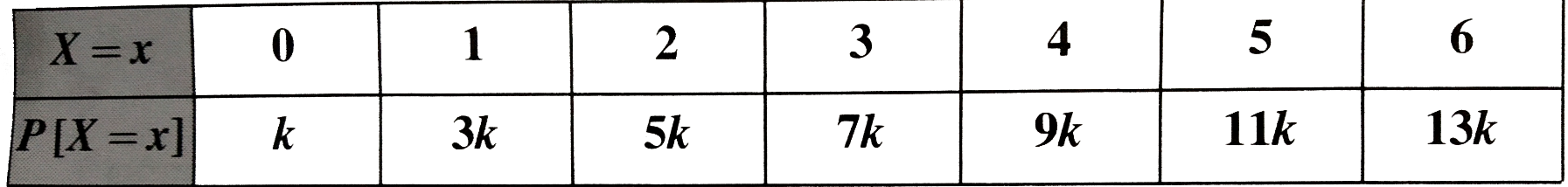
Find k.View SolutionA random variable X has the following probability distribution: Values of X: −2 −1 0 1 2 3 P(X):0.1 k 0.2 2k 0.3 k
View SolutionA random variable X has the follwing probability distribution:
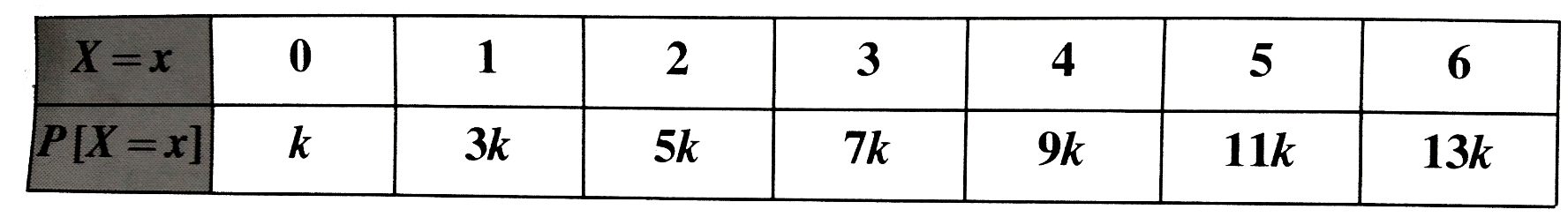
Find P(0<X<4)View SolutionA random variable X has the following probability distribution:Determine (i) k (ii) P(X<3)(iii) P(X>6) (iv) P(0<X<3)
View SolutionA random variable X has the following probability distribution: X=xi: 1 2 3 4 P(X=xi): k 2k 3k 4k Write the value of P(X≥3) .
View SolutionA random variable X has the following probability distribution:
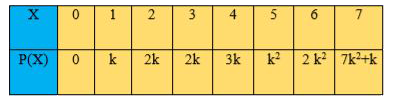
Determine : (i) k (ii) P(X<3) (iii) P(X>4)View SolutionA random variable X has the following probability distribution:
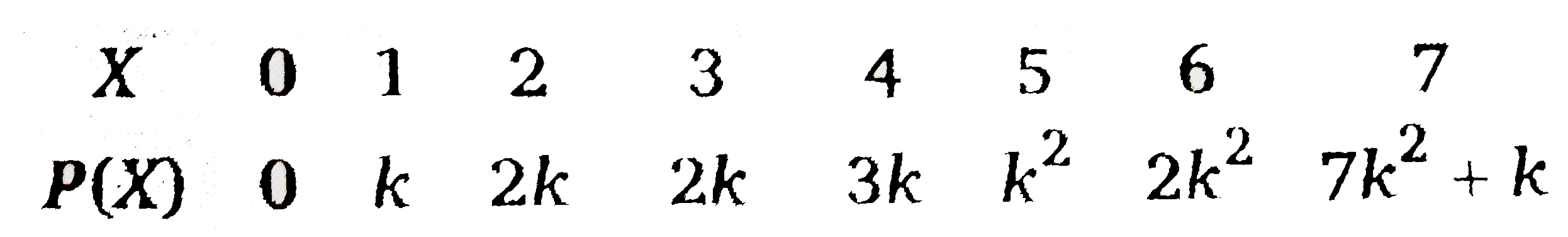
Determine :
(i) k
(ii) P(X<3)
(iii) P(X>6)
(iv) P(0<X<3)View SolutionA random variable 'X' has the following probability distribution :

Find : (i) the value of K (ii) P(X≤1)
(iii) P(X≥0).View Solution

