Find the electric field on the axis of a charged disc.
Consider a disc of uniform surface charge density 'σ'.
Let us calculate the electric field due to a charged ring of radius r. from the centre and having a width, dr. Here we are using the expression for electric field intensity for a charged ring of radius r at a point on the axis at a distance x from the centre.
E=kxq(x2+r2)3/2 directed along the axis outwards from the centre.
Thus electric field due to elementary ring.
dE=kxdq(x2+r2)3/2, directed along the line OP.
Now, the area of the ring, dS=2πr.dr
Charge on the elementary ring
⇒dq=σ2πrdr,
Thus, electric field due to entire disc
|E|p=∫dE=kxR∫0σ2πrdr(x2+r2)3/2=14π∈0x.σπR∫02r(x2+r2)3/2dr
E=σ2∈0[1−x(x2+R2)1/2]=σ2ε0(1−cosθ), where θ = semi vertical angle subtend by the disc at P.
Also, as R→∞,E=σ2∈∘, which is the electric field in front of an infinite plane sheet of charge.

Topper's Solved these Questions
- ELECTROSTANTICSBOOK - FIITJEECHAPTER - ELECTROSTANTICSEXERCISE - SOLVED PROBLEMS (OBJECTIVE)
15 Videos
- ELECTROSTANTICSBOOK - FIITJEECHAPTER - ELECTROSTANTICSEXERCISE - EXERCISE
10 Videos
- ELECTROSTANTICSBOOK - FIITJEECHAPTER - ELECTROSTANTICSEXERCISE - MATRIX MATCH TYPE
1 Videos
- ELECTROMAGNETIC WAVE & WAVE OPTICSBOOK - FIITJEECHAPTER - ELECTROMAGNETIC WAVE & WAVE OPTICSEXERCISE - ASSIGNMENT PROBLEMS Objective : Level-II (MULTI CHOICE SINGLE CORRECT)
21 Videos
- ELECTROSTATICSBOOK - FIITJEECHAPTER - ELECTROSTATICSEXERCISE - Example
14 Videos
Similar Questions
A ring of radius a contains a charge q distributed. uniformly ober its length. Find the electric field at a point. on the axis of the ring at a distance x from the centre.
View SolutionThe surface charge density of a thin charged disc of radius R is σ. The value of the electric field at the centre of the disc σ2∈0. With respect to the field at the centre, the electric field along the axis at a distance R from the centre of the disc is
View Solution
Knowledge Check
The surface charge density of a thin charged disc of radius R is σ. The value of the electric field at the centre of the disc is σ2∈o. With respect to the field at the centre, the electric field along the axis at a distance R from the centre of the disc reduces by
Areduces by 70.7%Breduces by 29.3%Creduces by 9.7%Dreduces by 14.6%A ring of radius a contains a charge q distributed uniformly over its length. Find the electric field at a point on the axis of the ring at a distance x from the centre.
Akq2x(a2+x2)32B2kq2x(a2+x2)32Ckq2x(a2+x2)32Dkq22x(a2+x2)32
The surface charge density of a thin charged disc of radius R is σ. The value of the electric field at the centre of the disc is σ2∈o. With respect to the field at the centre, the electric field along the axis at a distance R from the centre of the disc reduces by
View SolutionA thin copper ring of radius a is charged with q units of electricity. Calculate the electric field at a point at a distance x from the centre and on the axis of the ring. Use the result to find the electirc field due to a charged disc of surface density of charge ε.
View SolutionContinuous charge distribution(linear, surface and volumetric), Electric field due to slender rod, Electric field on the axis of a uniformly charged ring and disk
View Solution3 charges are placed in a circle of radius d as shown in figure. Find the electric field along x-axis at centre of circle.
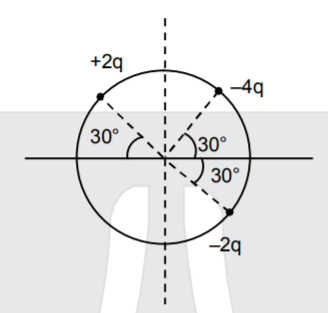
View Solution3 charges are placed in a circle of radius d as shown in figure. Find the electric field along x-axis at centre of circle.

View Solution3 charges are placed in a circle of radius d as shown in figure. Find the electric field along x-axis at centre of circle.

View Solution
