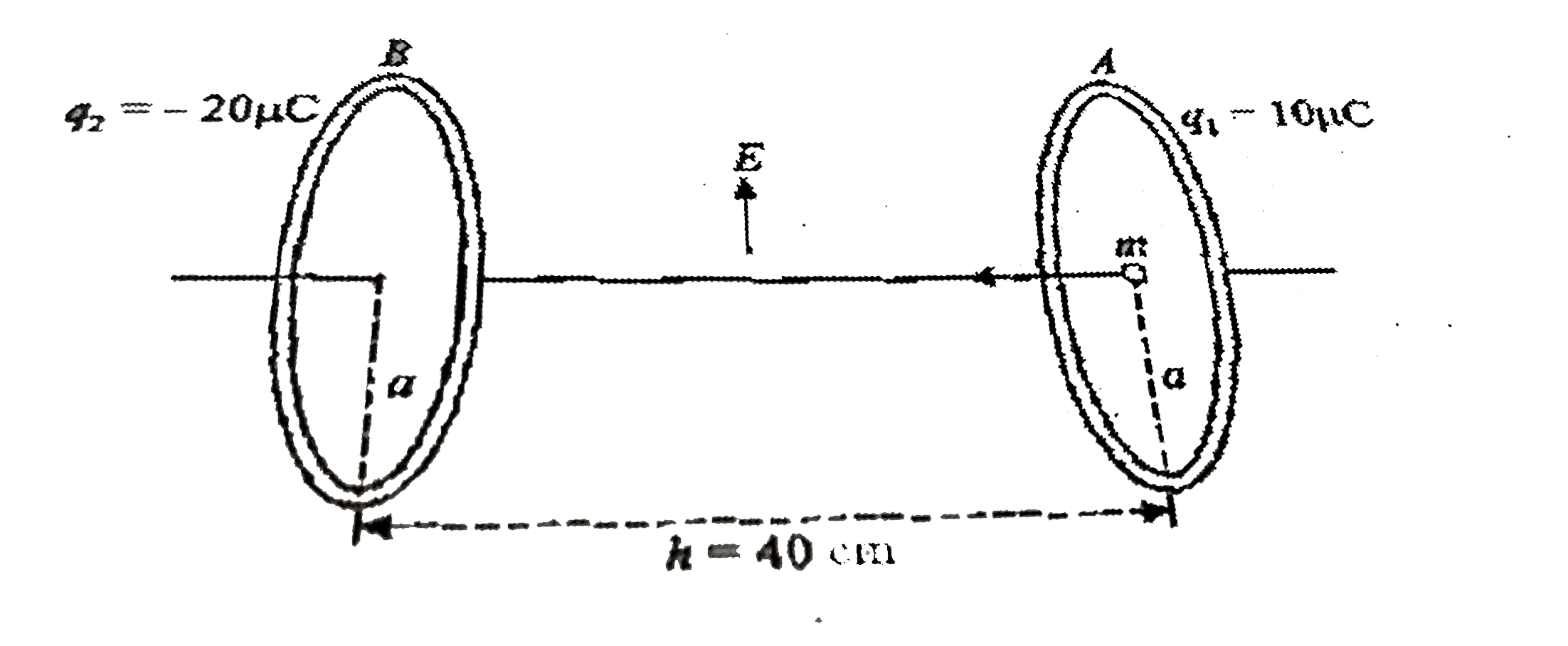
Two circular rings A and B each of radius a=30cm are placed co-axially with their axes horizontal in a uniform electric field E=105N/C directed vertically upward as shown in the figure. The distance between the centers of these rings A and B is h=40cm. Ring A has a positive charge q1=10μC while ring B has a negative charge of magnitude q2=20μC. A particle of mass m = 100 gm and carrying a positive charge q=10μC is released from rest at the centre of the ring A. Calculate its velocity when it has moved a distance 40 cm.

Weight of the particle W=mg=1001000×10=1 Newton
Force due to electrostatic field E=qE=10×10−6×105=1 Newton.
Thus the weight of the particle is balanced by the electrostatic force.
The particle experiences net force in the through the centers of ring A and B and is accelerated towards the centre of ring B. At the centre of ring B.
KE = Loss of PE
⇒12mv2=UA−UB=14π∈0qq1a+14π∈0q(−q2)√a2+h2−14π∈0qq1√a2+h2−14π∈0q(−q2)a
12mv2=q4π∈0[q1(1a−1√a2+h2)+q2(1a−1√a2+h2)]
=q4π∈0[(q1+q2)(√a2+h2−aa√a2+h2)]
⇒v=
⎷2q(q1+q2)4π∈0m[√a2+h2−aa√a2+h2]
For the given value v=6√2m/s
Topper's Solved these Questions
- BOOK - FIITJEECHAPTER - ELECTROSTANTICSEXERCISE - SOLVED PROBLEMS (OBJECTIVE)15videos
ELECTROSTANTICS
- BOOK - FIITJEECHAPTER - ELECTROSTANTICSEXERCISE - EXERCISE10videos
ELECTROSTANTICS
- BOOK - FIITJEECHAPTER - ELECTROSTANTICSEXERCISE - MATRIX MATCH TYPE1videos
ELECTROSTANTICS
- BOOK - FIITJEECHAPTER - ELECTROMAGNETIC WAVE & WAVE OPTICSEXERCISE - ASSIGNMENT PROBLEMS Objective : Level-II (MULTI CHOICE SINGLE CORRECT)21videos
ELECTROMAGNETIC WAVE & WAVE OPTICS
- BOOK - FIITJEECHAPTER - ELECTROSTATICSEXERCISE - Example14videos
ELECTROSTATICS
Similar Questions
Two identical circular rings A and B of radius 30 cm are placed coaxially with their axes horizontal in a uniform electric field E.=105N/C directed vertically upward as shown in figure. Distance between centres ofthese rings A and B is40cm. Ring A has a positive charge 10μC while ring B has a negative charge of magnitude 20μC. A particle of mass 100g and carrying a positive charge 10μC is released from rest at the centre of the ring A. Calculate its velocity when it has moved a distanceof40cm. Take g=10m/s2
[6√2m/s]View SolutionTwo circular rings A and B each of radius a = 30 cm are placed coaxially with their axis horizontal in a uniform electric field E=105NC−1 directed vertically upward as shown in figure. Distance between centers of the rings A and B (CAandCB)is40cm. Ring A has positive charge qA=10μC and B has a negative charge qB=−20μC. A particle of mass m and charge q=10μC is released from rest at the center of ring A. If particle moves along CACB, then
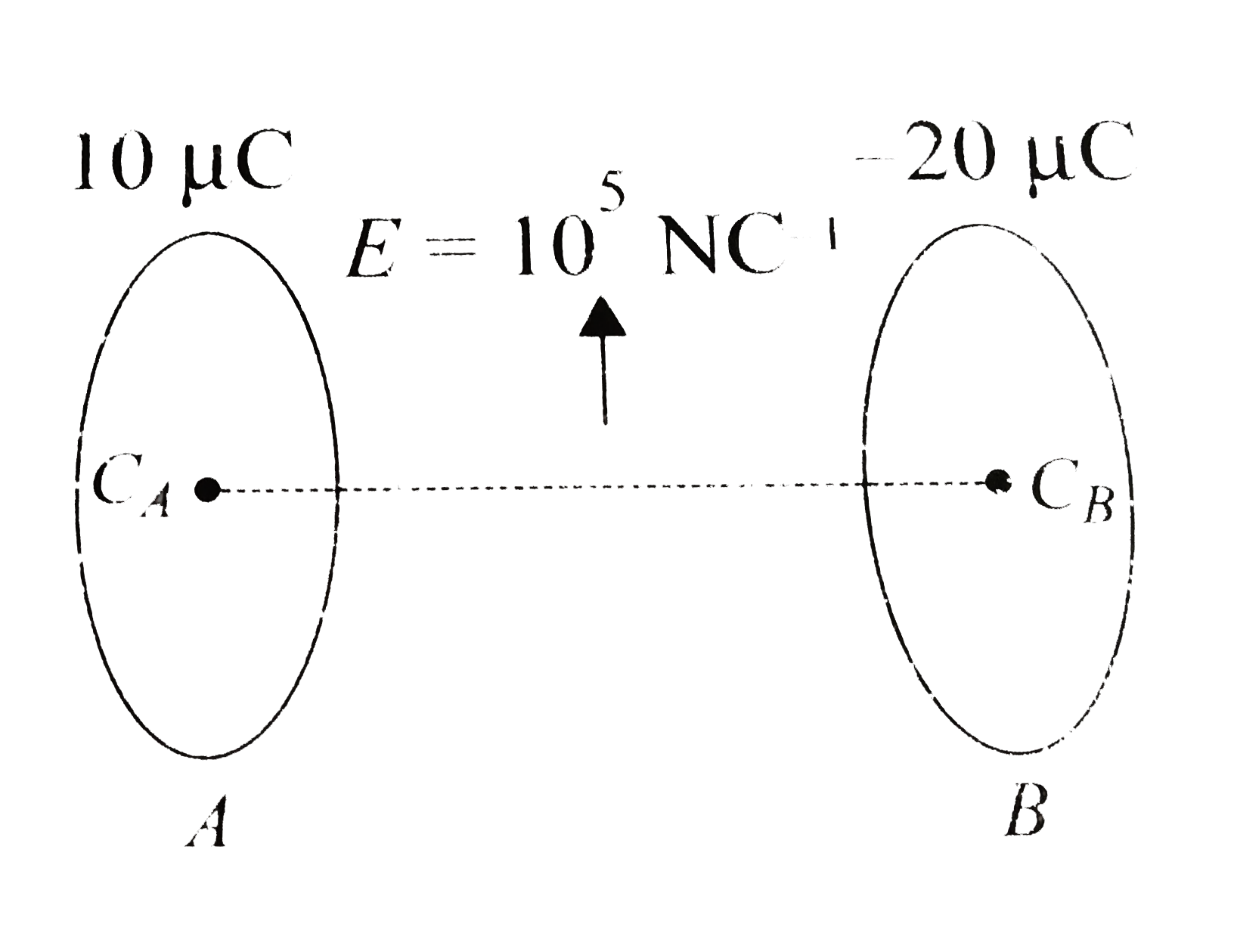
Speed of particle when it reaches at center of B isView Solution
Knowledge Check
- Question 1 - Select One
Two circular rings A and B each of radius a = 30 cm are placed coaxially with their axis horizontal in a uniform electric field E=105NC−1 directed vertically upward as shown in figure. Distance between centers of the rings A and B (CAandCB)is40cm. Ring A has positive charge qA=10μC and B has a negative charge qB=−20μC. A particle of mass m and charge q=10μC is released from rest at the center of ring A. If particle moves along CACB, then
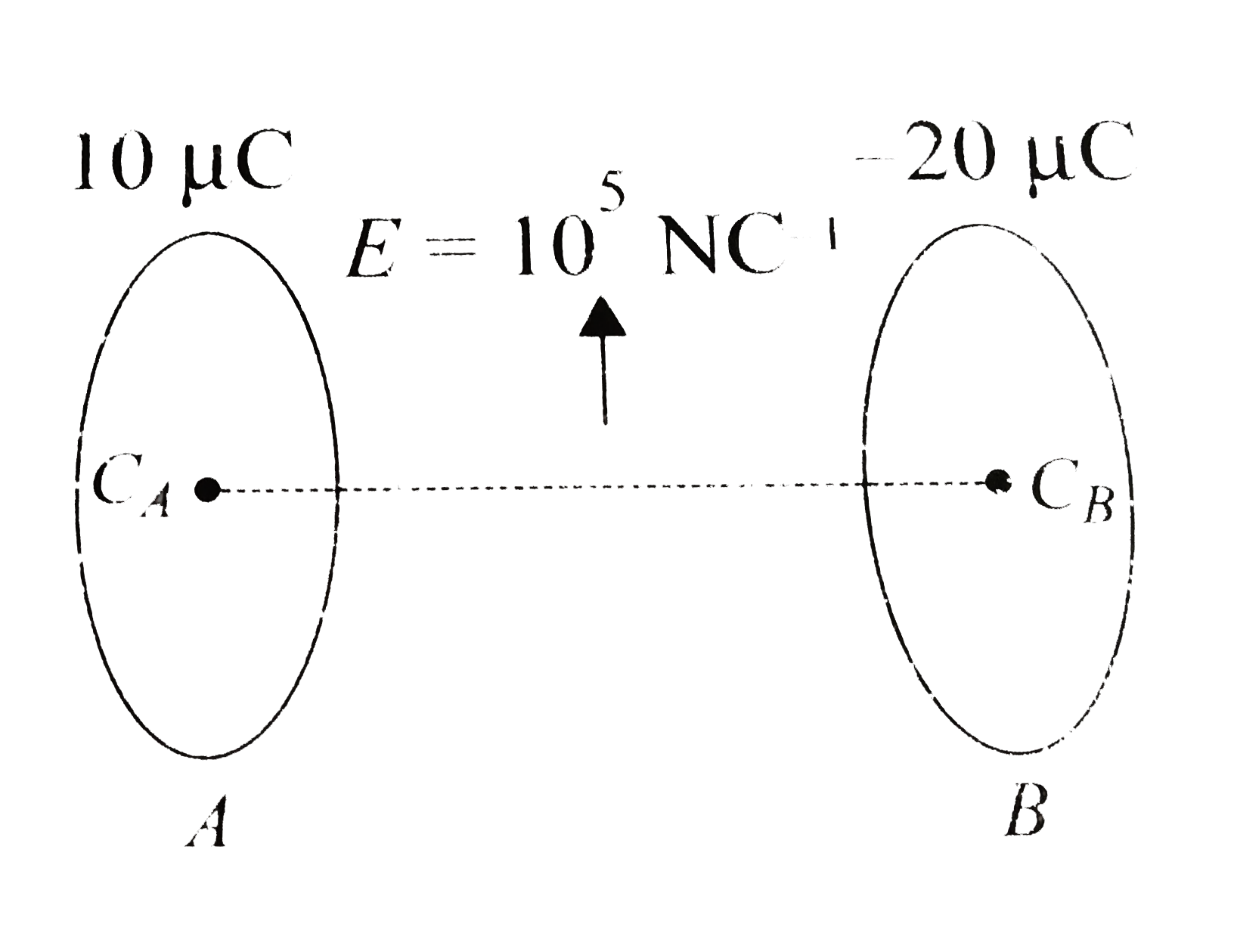
Speed of particle when it reaches at center of B isA6√2m/sB12√2m/sC2√6m/sD4√6m/s - Question 2 - Select One
Two circular rings A and B each of radius a = 30 cm are placed coaxially with their axis horizontal in a uniform electric field E=105NC−1 directed vertically upward as shown in figure. Distance between centers of the rings A and B (CAandCB)is40cm. Ring A has positive charge qA=10μC and B has a negative charge qB=−20μC. A particle of mass m and charge q=10μC is released from rest at the center of ring A. If particle moves along CACB, then
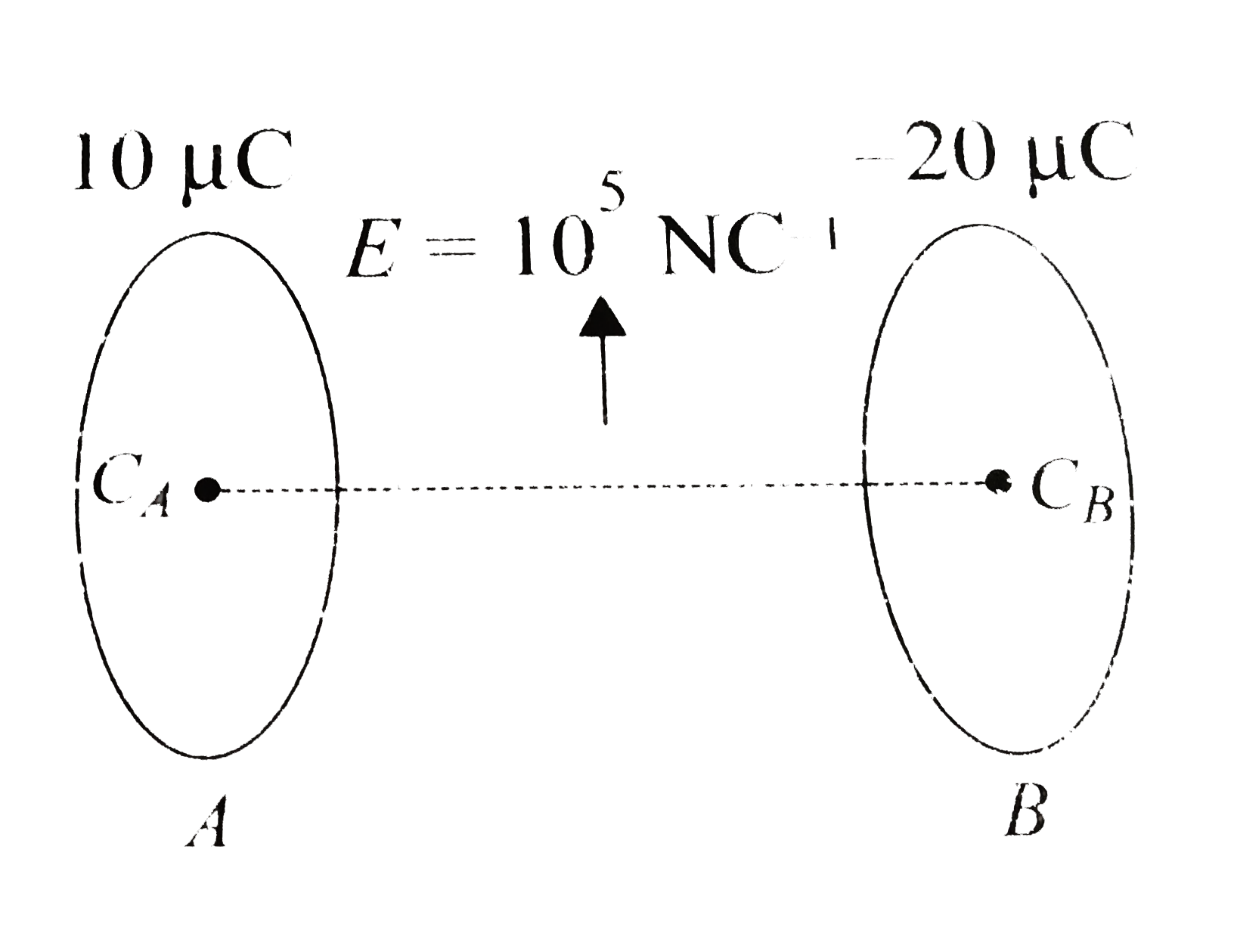
Work done by electric field, when particle moves from CA→CB isA−1.2JB1.2 JC−3.6JD3.6J - Question 3 - Select One
Two thin wire rings each having a radius R are placed at a distance d apart with their axes coiciding. The charges on the two rings are +q and −q. The potential difference between the centres of the two rings is
A(a) q2π∈0[1R−1√R2−d2]B(b) qR4π∈0d2C(c) q4π∈0[1R−1√R2+d2]D(d) zero
Two circular rings A and B each of radius a = 30 cm are placed coaxially with their axis horizontal in a uniform electric field E=105NC−1 directed vertically upward as shown in figure. Distance between centers of the rings A and B (CAandCB)is40cm. Ring A has positive charge qA=10μC and B has a negative charge qB=−20μC. A particle of mass m and charge q=10μC is released from rest at the center of ring A. If particle moves along CACB, then
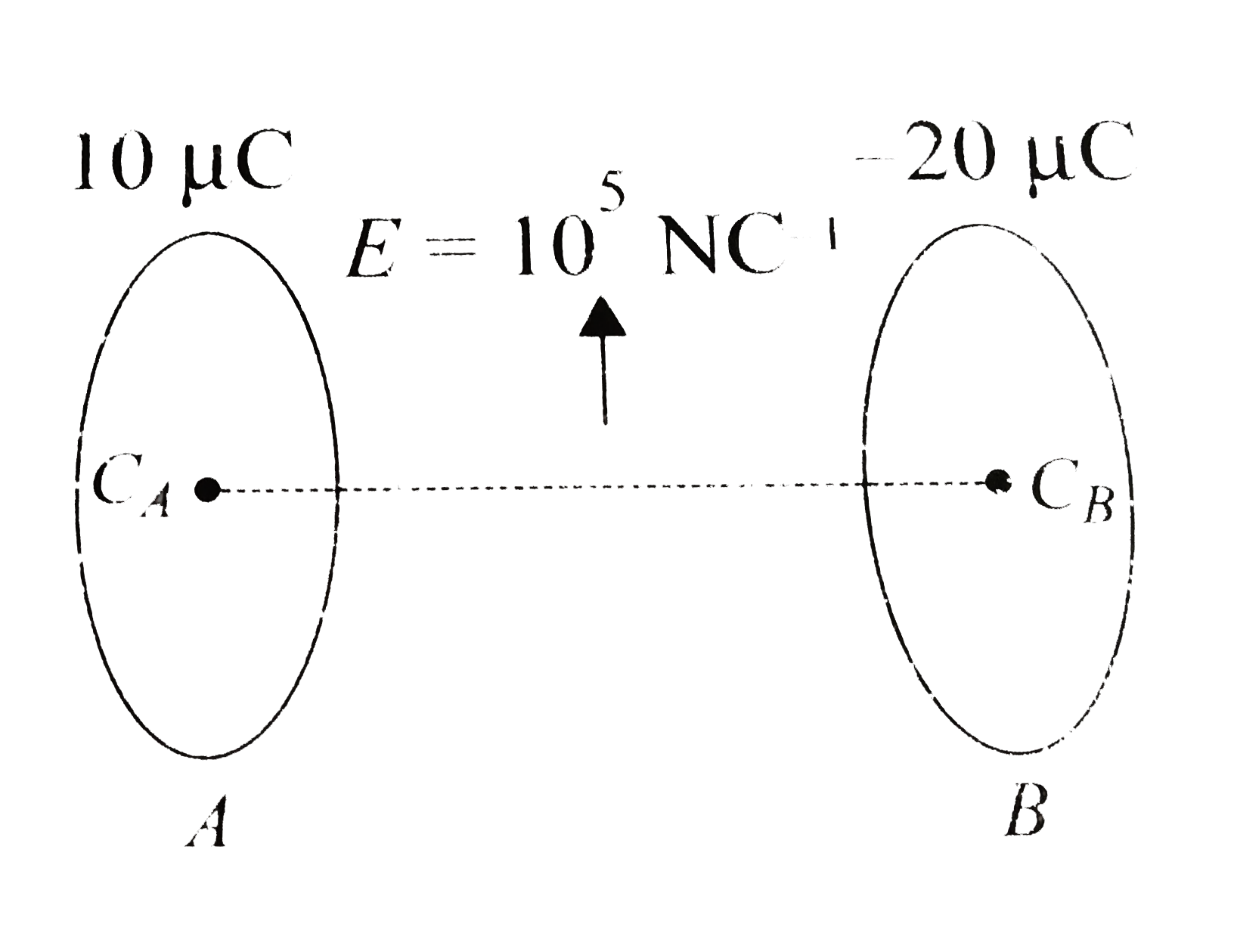
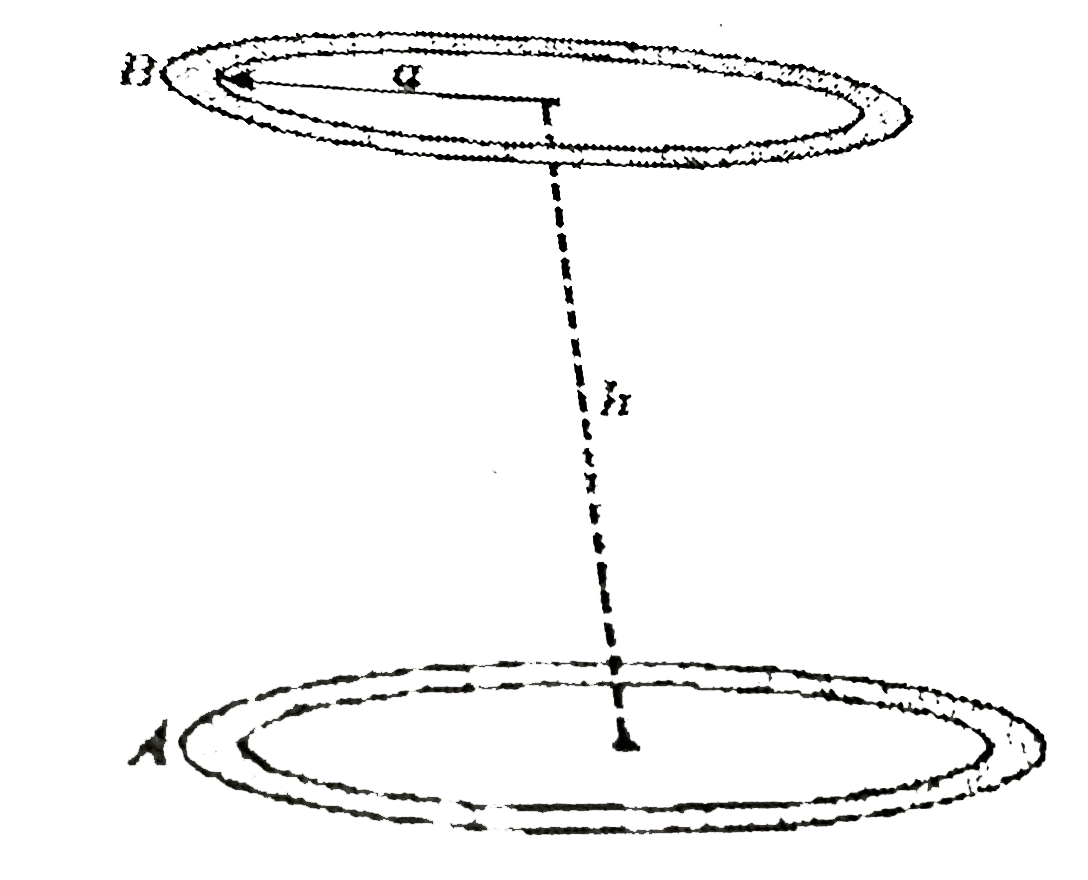
Work done by electric field, when particle moves from CA→CB isView SolutionTwo circular rings A and B, each of radius a = 30cm, are placed coaxiallywith their axes vertical as shown in figure−1.456. Distance between centres of these rings is h=40cm. Lower ring A has a positive charge of 10μC, while upper ringB has a negative charge of 20μC. A particle of mass m=100 gm carrying a positive charge of q=10μ C is released from rest at the centre of the ring ltbergt (a) Calculate initial acceleration of the particle.
(b) Calculate velocity of particle when it reaches at the centre ofupper ring B. (g=10ms−2)
 View Solution
View SolutionA positive charge Q is uniformly distributed along a circular ring of radius R.a small test charge q is placed at the centre of the ring.
 View Solution
View SolutionA positive charge Q is uniformly distributed along a circular ring of radius R.a small test charge q is placed at the centre of the ring .The
 View Solution
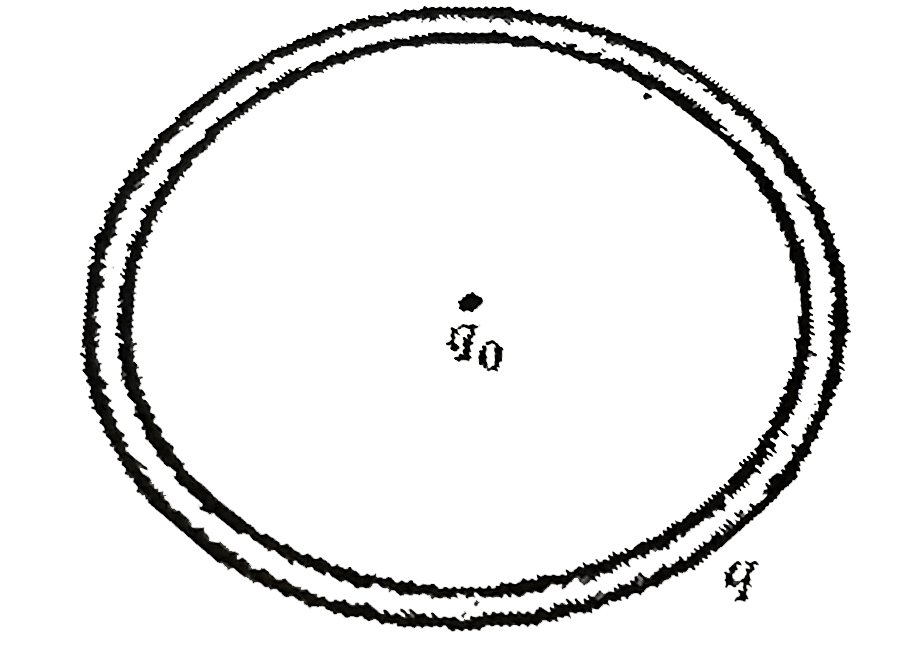
View SolutionA ring of radius R with a uniformly distributed charge q as shown in figure. A charge q0, is now placed at the centre of the ring. Find the increment in-the tension in ring.
 View Solution
View Solution
