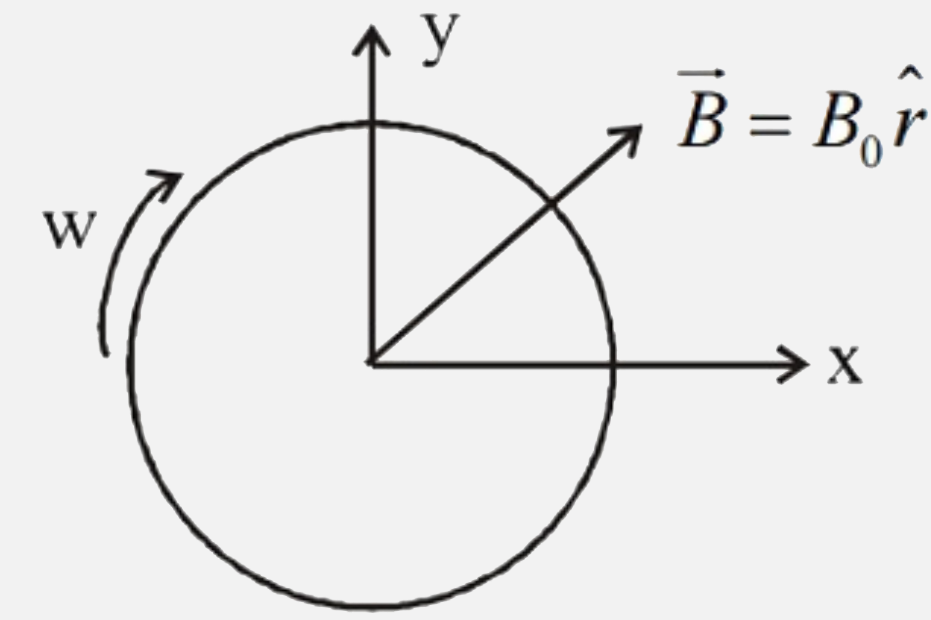
A thin non conducting disc of mass M = 2 kg, charge Q=2×102−C and radius R=16m is placed on a frictionless horizontal plane with its centre at the origin of the coordinate system. A non- uniform, radial magnetic field →B=B0ˆr is exists in space, where B0=10T and ˆr is a unit vector in the radially outward direction. The disc is set in motion with an angular velocity ω=x×102 rad s−1, about an axis passing through its centre and perpendicular to its plane, as shown in the figure. At what value of x, the disc will lift off from the surface.
The correct Answer is:9
Step by step video & image solution for A thin non conducting disc of mass M = 2 kg, charge Q = 2 xx 10^(2-) C and radius R = (1)/(6)m is placed on a frictionless horizontal plane with its centre at the origin of the coordinate system. A non- uniform, radial magnetic field vecB = B_(0) hat(r) is exists in space, where B_(0)= 10 T and hat(r) is a unit vector in the radially outward direction. The disc is set in motion with an angular velocity omega = x xx 10^(2)" rad s"^(-1), about an axis passing through its centre and perpendicular to its plane, as shown in the figure. At what value of x, the disc will lift off from the surface. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
A charge q is uniformly distributed on a non-conducting disc of radius R. It is rotated with an angular speed co about an axis passing through the centre of mass of the disc and perpendicular to its plane. Find the magnetic moment of the disc.
View SolutionAn electric field →E=Bxˆi exists in space, where B=20V/m2. Taking the potential at (2m, 4m) to be zero, find the potential at the origin.
View Solution
Knowledge Check
A thin uniform circular disc of mass M and radius R is rotating in a horizontal plane about an axis passing through its centre and perpendicular to its plane with an angular velocity ω. Another disc of same dimensions but of mass M/4 is placed gently on the first disc coaxially. The angular velocity of the system now is
A2ω√2B4ω5C5ω4D3ω4A thin uniform circular disc of mass M and radius R is rotating in a horizontal plane about an axis passing through its centre and perpendicular to its plane with an angular velocity ω . Another disc of same dimensions but of mass 14 M is placed gently on the first disc co-axially. The angular velocity of the system is
A23ωB45ωC34ωD13ωA bar magnet having a magnetic moment of 2×104JT−1 is free to rotate in a horizontal plane. A horizontal magnetic field B=6×10−4 T exists in the space. The work done in taking the magnet slowly from a direction parallel to the field to a direction 60∘ from the field is
A12JB6JC2JD0.6J
An electric field →E=ˆiCx exists in the space, where C = 10 V/m2 . Taking the potential at (10 m, 20 m ) to be zero, find the potential at the origin.
View SolutionA thin disc of mass M and radius R has mass per unit area σ(r)=kr2, where r is the distnace from its centre. Its moment of inertia about an axis going through its centre of mass and perpendicular to its plane is xMR2, where x is _________
View SolutionIf the work required to move the conductor shown in figure, one full turn in the positive direction at a rotational frequency N revolutions per minute, if →B=B0ˆar(B0 is positive constant and ˆar is a unit vector in radial direciton ), is yπrB0il. Then y is .

View SolutionA magnetic field →B=−B0ˆi exists within a sphere of radius R=v0T√3 where T is the time period of one revolution of a charged particle starting its motion form origin and moving with a velocity vce(v)0=v02√3ˆi−v02ˆj. Find the number of turns that the particle will take to come out of the magnetic field.
View SolutionA non - conducting ring of mass m = 4 kg and radius R = 10 cm has charge Q = 2 C uniformly distributed over its circumference. The ring is placed on a rough horizontal surface such that the plane of the ring is parallel to the surface. A vertical magnetic field B=4t3T is switched on at t = 0. At t = 5 s ring starts to rotate about the vertical axis through the centre. The coefficient of friction between the ring and the surface is found to be k24. Then the value of k is
View Solution
